- #1
karnten07
- 213
- 0
Homework Statement
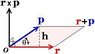
Consider a body falling from a tower in the northern hemisphere at approx 40 degrees latitude. The body is seen to have a displacement to the east. Explain the origin of this displacement qualitatively from the point of view of a non-inertial observer. What local effect is responsible for this phenomenon.
[Hint: recall the righthand rule]
Homework Equations
The Attempt at a Solution
The rotation of the Earth causes acceleration of the local frame. An inertial force throws the body outwards away from the Earth's axis of rotation.
I don't understand how to apply the right hand rule here and how this shows me that the body has a displacement to the east.