What's the fundamental group of a punctured torus?

In summary, the fundamental group of a punctured torus is Z*Z*Z, which can be shown by continuously deforming the punctured torus into two cylinders glued to a square patch. This is because the fundamental group of the punctured torus is equivalent to the fundamental group of the torus with an extra loop, which is the edge circle of the punctured hole. However, the fundamental group of the torus itself is ZxZ.
  • #1
kakarotyjn
98
0
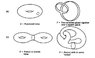
The fundamental group of a torus is Z[tex]*[/tex]Z,then the fundamental group of a punctured torus is Z[tex]*[/tex]Z[tex]*[/tex]Z.

But I've ever done a problem,it said a punctured torus can be continuously deformed into two cylinders glued to a square patch.Really?

If that is right,then the fundamental group of punctured torus is Z[tex]*[/tex]Z.

Which is right?Need help:smile:
 

Attachments

  • http_imgload.jpg
    http_imgload.jpg
    21.7 KB · Views: 2,284
Physics news on Phys.org
  • #2
Yes, it's true... imagine making the hole bigger and bigger.. make it as big as you can withouth changing the topology. You're left with the two strips glued on a square patch.
 
  • #3
oh,i see.the fundamental group should be Z*Z. i consider an extra loop,which is the edge circle of the punctured hole. but now I know it's the 2 power of a generator.
 
  • #4
The fundamental group of the torus is not Z*Z though, it is ZxZ.
 
  • #5


Both answers are correct, it just depends on how you define the punctured torus. The fundamental group is a topological invariant, meaning it does not change under continuous deformations. So, if you define a punctured torus as a torus with a single point removed, then the fundamental group would be Z*Z*Z. However, if you define a punctured torus as two cylinders glued to a square patch, then the fundamental group would be Z*Z. Both definitions are valid and give different fundamental groups. It is important to carefully define the objects being studied in order to get the correct fundamental group.
 

1. What is a punctured torus?

A punctured torus is a two-dimensional surface that is topologically equivalent to a donut with a hole in the middle. It is a common object studied in topology and geometry.

2. What is the fundamental group of a punctured torus?

The fundamental group of a punctured torus is a mathematical group that describes the possible ways to continuously deform a loop on the surface of the torus without tearing or gluing it. It is denoted by the symbol π1(T), where T represents the punctured torus.

3. How is the fundamental group of a punctured torus calculated?

The fundamental group of a punctured torus can be calculated using the Seifert-van Kampen theorem, which states that the fundamental group of a space can be obtained by combining the fundamental groups of its subspaces. In this case, the punctured torus can be decomposed into two subspaces, a torus and a circle, whose fundamental groups can be easily determined.

4. What is the significance of the fundamental group of a punctured torus?

The fundamental group of a punctured torus is a fundamental concept in algebraic topology and has many applications in mathematics and physics. It provides a way to classify and distinguish different types of surfaces, and it also has connections to other areas of mathematics such as group theory and differential geometry.

5. Can the fundamental group of a punctured torus be generalized to higher dimensions?

Yes, the concept of the fundamental group can be extended to higher dimensions. In three dimensions, the fundamental group is replaced by the fundamental groupoid, which is a more general version that allows for the consideration of multiple base points. In higher dimensions, the concept of homotopy groups is used to describe the possible ways to deform higher-dimensional objects without changing their topological properties.

Similar threads

Fundamental Group of the Torus-Figure 8
    • Differential Geometry
Replies
4
Views
4K
I Why isn't this a Lie group?
    • Differential Geometry
Replies
17
Views
3K
A About Chern number of U(1) principal bundle on a torus
    • Differential Geometry
Replies
28
Views
5K
A Classification of reductive groups via root datum
    • Linear and Abstract Algebra
Replies
3
Views
789
A Fundamental group of n connect tori with one point removed
    • Topology and Analysis
Replies
9
Views
2K
A Can I find a smooth vector field on the patches of a torus?
    • Differential Geometry
Replies
4
Views
2K
Fundemental group, Torus, SVK
    • Differential Geometry
Replies
3
Views
3K
A Fundamental group of Project Plane with 2 points missing
    • Topology and Analysis
Replies
6
Views
2K
DeRham Cohomology of n-torus
    • Differential Geometry
Replies
4
Views
5K
Mapping Class Group of the Torus.
    • Differential Geometry
Replies
4
Views
4K

Hot Threads

Recent Insights

Back
Top