- #1
Nikitin
- 735
- 27
Hi. I never understood why the momentum wave function ##\phi (p)## is the Fourier transform/integral of the real space wave function ##\psi (x)##. Basically, the second pair of formulas here http://quantummechanics.ucsd.edu/ph130a/130_notes/node82.html,
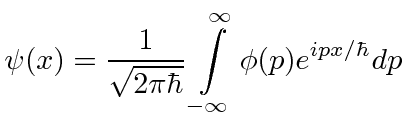 and
and
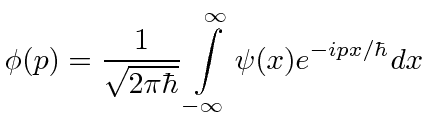 (and the rest of the text, for that matter) makes little sense to me. Is there any intuitive reason behind the formulas?
(and the rest of the text, for that matter) makes little sense to me. Is there any intuitive reason behind the formulas?
Could you guys also help me understand the dirac delta normation?
Thanks for any help!
Could you guys also help me understand the dirac delta normation?
Thanks for any help!
Last edited: