- #1
Eus
- 94
- 0
Hi Ho!
I know that many books show the way to derive the moment inertia of a solid and a hollow sphere in many ways, each according to the lines of reasoning of their authors.
I also have my own line of reasoning that I have successfully applied in finding the moment inertia of a solid sphere, but not the one of the hollow sphere.
I have asked two of my lecturers to help me find the mistake in my line of reasoning. But, both just showed me their own lines of reasoning. The first one directly pointed out to me that I should had used a spherical coordinate system when I had barely said, "Sir, I have a problem in deriving the moment inertia of a hollow sphere..." The other one said that I should had consulted a mathematician instead after he explained his way of deriving the moment inertia. Therefore, would you please help me find the mistake in my line of reasoning that I write down below?
Moment inertia of a point object is defined as: [itex]I=M\ R^2[/itex].
Because I know that [itex]dx[/itex] means a very very small quantity, firstly I tried to calculate the moment inertia of a solid sphere [itex]\left ( I=\frac{2}{5}\ M\ R^2 \right )[/itex] as follows:
1. I used a 3-D Cartesian coordinate and I put a solid sphere having a radius [itex]R[/itex], a total mass [itex]M[/itex], and a uniform mass distribution, centered at the origin and rotated about the [itex]z[/itex]-axis.
2. Because the solid sphere was symmetric with respect to the [itex]x-y[/itex] plane, I only considered the upper half of it, which was located in the [itex]+z[/itex] direction.
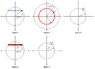
3. I thinly sliced the upper half part of the sphere parallel with the [itex]x-y[/itex] plane. This resulted in many circular disks with varying diameter depending on their distances from the [itex]x-y[/itex] plane. One such disk was depicted in Figure 1 as a blue rectangle. This particular disk, which was as thin as [itex]dz[/itex], was located at distance [itex]z[/itex] units from the [itex]x-y[/itex] plane, and therefore it has a diameter of [itex]\sqrt{R^2-z^2}[/itex].
4. Because the thin disks were not point objects, I could not apply the formula yet. Therefore, I took one such thin disk and started to thinly slice it into many rings with varying diameter. One such ring was depicted in Figure 2.
5. Again, because the thin rings, which were as thin as [itex]dr[/itex], were not point objects, I could not apply the formula yet. Therefore, I took one such thin ring and started to thinly slice it into many small boxes of size [itex]dz[/itex] x [itex]dr[/itex] x [itex]ds[/itex] as depicted in Figure 3.
6. Because the boxes were very very small, they can be regarded as point objects. Therefore, I could apply the formula now.
7. First, the formula called for the mass of the point object. The mass of the solid sphere could be related to its volume as [itex]M=\rho\ V[/itex]. Because the solid sphere had a uniform mass distribution, the boxes had very very small masses [itex]dm[/itex] that could be related to its very very small volumes [itex]dv[/itex], where [itex]dv=(dz)(dr)(ds)[/itex], as [itex]dm=\rho\ dv[/itex].
8. Looking at Figure 3, [itex]di_{box}=r^2\ dm[/itex]. To get the total moment inertia for the thin ring, the moment inertia of each box along the ring was summed up as follows:
[tex]
di_{ring}=\int^{2\pi}_{0}di_{box}
[/tex]
[tex]
di_{ring}=\int^{2\pi}_{0}r^2\ dm
[/tex]
9. Because the integration over the whole ring had to be in terms of [itex]ds[/itex], the [itex]dm[/itex] term was decomposed as follows:
[tex]
di_{ring}=\int^{2\pi}_{0}r^2\ (\rho\ dv)
[/tex]
[tex]
di_{ring}=\rho\ \int^{2\pi}_{0}r^2\ dv
[/tex]
[tex]
di_{ring}=\rho\ \int^{2\pi}_{0}r^2\ (dz\ dr\ ds)
[/tex]
[tex]
di_{ring}=\rho\ r^2\ dz\ dr\ \int^{2\pi}_{0}ds
[/tex]
10. Therefore, [itex]di_{ring}=\rho\ r^2\ dz\ dr\ (2\pi\ r)[/itex], which was simply [itex]di_{ring}=2\pi\ \rho\ r^3\ dz\ dr[/itex].
11. Next, the moment inertia of each thin ring was integrated over the whole thin disk in terms of [itex]dr[/itex] to get the moment inertia of each thin disk as follows:
[tex]
di_{disk}=\int^{\sqrt{R^2-z^2}}_{0}di_{ring}
[/tex]
[tex]
di_{disk}=\int^{\sqrt{R^2-z^2}}_{0}2\pi\ \rho\ r^3\ dz\ dr
[/tex]
[tex]
di_{disk}=2\pi\ \rho\ dz\ \int^{\sqrt{R^2-z^2}}_{0}r^3\ dr
[/tex]
[tex]
di_{disk}=2\pi\ \rho\ dz\ \left (\frac{1}{4}\ \left \vert r^4 \right \vert ^{\sqrt{R^2-z^2}}_{0}\right )
[/tex]
[tex]
di_{disk}=\frac{1}{2}\ \pi\ \rho\ dz\ \left(R^2-z^2\right)^2
[/tex]
12. Finally, the moment inertia of the upper half of the sphere could be found by integrating the moment inertia of each thin disk over the whole length [itex]R[/itex] along the [itex]+z[/itex]-axis as follows:
[tex]
I_{\frac{1}{2}solid\ sphere}=\int^{R}_{0}di_{disk}
[/tex]
[tex]
I_{\frac{1}{2}solid\ sphere}=\int^{R}_{0}\frac{1}{2}\ \pi\ \rho\ \left(R^2-z^2\right)^2\ dz
[/tex]
[tex]
I_{\frac{1}{2}solid\ sphere}=\frac{1}{2}\pi\ \rho\ \int^{R}_{0}\left(R^4-2R^2\ z^2+z^4\right)\ dz
[/tex]
[tex]
I_{\frac{1}{2}solid\ sphere}=\frac{1}{2}\ \pi\ \rho\ \left \vert R^4\ z-\frac{2}{3}\ R^2\ z^3+\frac{1}{5}\ z^5\ \right \vert ^{R}_{0}
[/tex]
[tex]
I_{\frac{1}{2}solid\ sphere}=\frac{1}{2}\ \pi\ \rho\ \left(R^5-\frac{2}{3}\ R^5+\frac{1}{5}\ R^5\right)
[/tex]
[tex]
I_{\frac{1}{2}solid\ sphere}=\frac{1}{2}\ \pi\ \rho\ \left(\frac{8}{15}\ R^5\right)
[/tex]
[tex]
I_{\frac{1}{2}solid\ sphere}=\frac{4}{15}\ \pi\ \rho\ R^5
[/tex]
13. To find the moment inertia of the whole solid sphere, the moment inertia of the upper half of the solid sphere was multiplied by two as follows:
[tex]
I_{solid\ sphere}=2I_{\frac{1}{2}solid\ sphere}
[/tex]
[tex]
I_{solid\ sphere}=2\left(\frac{4}{15}\ \pi\ \rho\ R^5\right)
[/tex]
[tex]
I_{solid\ sphere}=\frac{8}{15}\ \pi\ \rho\ R^5
[/tex]
14. As the last step, the moment inertia of the whole solid sphere was given in terms of its mass [itex]M[/itex]. The relation of [itex]M=\rho\ V[/itex] where [itex]V=\frac{4}{3}\pi\ R^3[/itex] was substituted as follows:
[tex]
I_{solid\ sphere}=\frac{8}{15}\ \pi\ \rho\ R^5
[/tex]
[tex]
I_{solid\ sphere}=\frac{8}{15}\ \pi\ \left(\frac{3}{4}\ \frac{1}{\pi}\ \frac{M}{R^3}\right)\ R^5
[/tex]
[tex]
I_{solid\ sphere}=\frac{2}{5}\ M\ R^2
[/tex]
Yup, I got the right result!
Now since I was able to calculate the moment inertia of a solid sphere with the idea of [itex]dx[/itex] as a very very small quantity, finally I tried to calculate the moment inertia of a hollow sphere (a thin spherical shell), which was actually only a very very thin plate as the surface of a hollow sphere, [itex]\left (\frac{2}{3}\ M\ R^2\right )[/itex] as follows:
1. I used a 3-D Cartesian coordinate and I put a hollow sphere having a radius [itex]R[/itex], a total mass [itex]M[/itex], and a uniform mass distribution, centered at the origin and rotated about the [itex]z[/itex]-axis.
2. Because the solid sphere was symmetric with respect to the [itex]x-y[/itex] plane, I only considered the upper half of it, which was located in the [itex]+z[/itex] direction.
3. I thinly sliced the upper half part of the sphere parallel with the [itex]x-y[/itex] plane. This resulted in many circular rings with varying diameter depending on their distances from the [itex]x-y[/itex] plane. One such ring was depicted in Figure 4 as a red rectangle. This particular ring, which was as thin as [itex]dz[/itex], was located at distance [itex]z[/itex] units from the [itex]x-y[/itex] plane, and therefore it has a diameter of [itex]\sqrt{R^2-z^2}[/itex].
4. Because the thin rings were not point objects, I could not apply the formula yet. Therefore, I took one such thin ring and started to thinly slice it into many small plates of size [itex]dz[/itex] x [itex]ds[/itex] as depicted in Figure 5.
5. Because the plates were very very small, they can be regarded as point objects. Therefore, I could apply the formula now.
6. First, the formula called for the mass of the point object. The mass of the hollow sphere could be related to its surface area as [itex]M=\rho\ A[/itex]. Because the hollow sphere had a uniform mass distribution, the plates had very very small masses [itex]dm[/itex] that could be related to its very very small areas [itex]da[/itex], where [itex]da=(dz)(ds)[/itex], as [itex]dm=\rho\ da[/itex].
7. Looking at Figure 5, [itex]di_{plate}=(\sqrt{R^2-z^2})^2\ dm[/itex]. To get the total moment inertia for the thin ring, the moment inertia of each plate along the ring was summed up as follows:
[tex]
di_{ring}=\int^{2\pi}_{0}di_{plate}
[/tex]
[tex]
di_{ring}=\int^{2\pi}_{0}(R^2-z^2)\ dm
[/tex]
8. Because the integration over the whole ring had to be in terms of [itex]ds[/itex], the [itex]dm[/itex] term was decomposed as follows:
[tex]
di_{ring}=\int^{2\pi}_{0}(R^2-z^2)(\rho\ da)
[/tex]
[tex]
di_{ring}=\rho\ \int^{2\pi}_{0}(R^2-z^2)\ da
[/tex]
[tex]
di_{ring}=\rho\ \int^{2\pi}_{0}(R^2-z^2)(dz\ ds)
[/tex]
[tex]
di_{ring}=\rho\ (R^2-z^2)\ dz\ \int^{2\pi}_{0}\ ds
[/tex]
9. Therefore, [itex]di_{ring}=\rho\ (R^2-z^2)\ dz\ (2\pi\ \sqrt{R^2-z^2})[/itex], which was simply [itex]di_{ring}=2\pi\ \rho\ (R^2-z^2)^{\frac{3}{2}}\ dz[/itex].
10. Finally, the moment inertia of the upper half of the hollow sphere could be found by integrating the moment inertia of each thin ring over the whole length [itex]R[/itex] along the [itex]+z[/itex]-axis as follows:
[tex]
I_{\frac{1}{2}\ hollow\ sphere}=\int^{R}_{0}di_{ring}
[/tex]
[tex]
I_{\frac{1}{2}\ hollow\ sphere}=\int^{R}_{0}2\pi\ \rho\ (R^2-z^2)^{\frac{3}{2}}\ dz
[/tex]
[tex]
I_{\frac{1}{2}\ hollow\ sphere}=2\pi\ \rho\ \int^{R}_{0}(R^2-z^2)^{\frac{3}{2}}\ dz
[/tex]
It can be calculated that:
[tex]
\int (a^2-x^2)^{\frac{3}{2}}\ dx=\frac{1}{8}((5a^2-2x^2)(x\ \sqrt{a^2-x^2})+3a^4\ \arcsin(\frac{x}{a}))+c
[/tex]
Therefore, the calculation of [itex]I_{\frac{1}{2}\ hollow\ sphere}[/itex] can be continued as follows:
[tex]
I_{\frac{1}{2}\ hollow\ sphere}=2\pi\ \rho\ \frac{1}{8} \left \vert (5R^2-2z^2)(z\ \sqrt{R^2-z^2})+3R^4\ \arcsin(\frac{z}{R}) \right \vert^{R}{0}
[/tex]
[tex]
I_{\frac{1}{2}\ hollow\ sphere}=2\pi\ \rho\ \frac{1}{8}\ (3R^4\ \arcsin(\frac{R}{R}))
[/tex]
[tex]
I_{\frac{1}{2}\ hollow\ sphere}=2\pi\ \rho\ \frac{1}{8}\ (3R^4\ \frac{\pi}{2})
[/tex]
[tex]
I_{\frac{1}{2}\ hollow\ sphere}=\frac{3}{8}\ \pi^2\ \rho\ R^4
[/tex]
11. To find the moment inertia of the whole hollow sphere, the moment inertia of the upper half of the hollow sphere was multiplied by two as follows:
[tex]
I_{hollow\ sphere}=2I_{\frac{1}{2}\ hollow\ sphere}
[/tex]
[tex]
I_{hollow\ sphere}=2(\frac{3}{8}\ \pi^2\ \rho\ R^4)
[/tex]
[tex]
I_{hollow\ sphere}=\frac{3}{4}\ \pi^2\ \rho\ R^4
[/tex]
12. As the last step, the moment inertia of the whole hollow sphere was given in terms of its mass [itex]M[/itex]. The relation of [itex]M=\rho\ A[/itex] where [itex]A=4\pi\ R^2[/itex] was substituted as follows:
[tex]
I_{hollow\ sphere}=\frac{3}{4}\ \pi^2\ \rho\ R^4
[/tex]
[tex]
I_{hollow\ sphere}=\frac{3}{4}\ \pi^2\ \left (\frac{M}{4\pi\ R^2}\right )\ R^4
[/tex]
[tex]
I_{hollow\ sphere}=\frac{3}{16}\ \pi\ M\ R^2
[/tex]
I got the wrong result this time. But, why? Isn't that I had successfully applied my line of reasoning in finding the moment inertia of a solid sphere? What's wrong with my line of reasoning? Did I miss some important points in finding the moment inertia of a hollow sphere?
Thank you very much.
Eus
I know that many books show the way to derive the moment inertia of a solid and a hollow sphere in many ways, each according to the lines of reasoning of their authors.
I also have my own line of reasoning that I have successfully applied in finding the moment inertia of a solid sphere, but not the one of the hollow sphere.
I have asked two of my lecturers to help me find the mistake in my line of reasoning. But, both just showed me their own lines of reasoning. The first one directly pointed out to me that I should had used a spherical coordinate system when I had barely said, "Sir, I have a problem in deriving the moment inertia of a hollow sphere..." The other one said that I should had consulted a mathematician instead after he explained his way of deriving the moment inertia. Therefore, would you please help me find the mistake in my line of reasoning that I write down below?
Moment inertia of a point object is defined as: [itex]I=M\ R^2[/itex].
Because I know that [itex]dx[/itex] means a very very small quantity, firstly I tried to calculate the moment inertia of a solid sphere [itex]\left ( I=\frac{2}{5}\ M\ R^2 \right )[/itex] as follows:
1. I used a 3-D Cartesian coordinate and I put a solid sphere having a radius [itex]R[/itex], a total mass [itex]M[/itex], and a uniform mass distribution, centered at the origin and rotated about the [itex]z[/itex]-axis.
2. Because the solid sphere was symmetric with respect to the [itex]x-y[/itex] plane, I only considered the upper half of it, which was located in the [itex]+z[/itex] direction.
3. I thinly sliced the upper half part of the sphere parallel with the [itex]x-y[/itex] plane. This resulted in many circular disks with varying diameter depending on their distances from the [itex]x-y[/itex] plane. One such disk was depicted in Figure 1 as a blue rectangle. This particular disk, which was as thin as [itex]dz[/itex], was located at distance [itex]z[/itex] units from the [itex]x-y[/itex] plane, and therefore it has a diameter of [itex]\sqrt{R^2-z^2}[/itex].
4. Because the thin disks were not point objects, I could not apply the formula yet. Therefore, I took one such thin disk and started to thinly slice it into many rings with varying diameter. One such ring was depicted in Figure 2.
5. Again, because the thin rings, which were as thin as [itex]dr[/itex], were not point objects, I could not apply the formula yet. Therefore, I took one such thin ring and started to thinly slice it into many small boxes of size [itex]dz[/itex] x [itex]dr[/itex] x [itex]ds[/itex] as depicted in Figure 3.
6. Because the boxes were very very small, they can be regarded as point objects. Therefore, I could apply the formula now.
7. First, the formula called for the mass of the point object. The mass of the solid sphere could be related to its volume as [itex]M=\rho\ V[/itex]. Because the solid sphere had a uniform mass distribution, the boxes had very very small masses [itex]dm[/itex] that could be related to its very very small volumes [itex]dv[/itex], where [itex]dv=(dz)(dr)(ds)[/itex], as [itex]dm=\rho\ dv[/itex].
8. Looking at Figure 3, [itex]di_{box}=r^2\ dm[/itex]. To get the total moment inertia for the thin ring, the moment inertia of each box along the ring was summed up as follows:
[tex]
di_{ring}=\int^{2\pi}_{0}di_{box}
[/tex]
[tex]
di_{ring}=\int^{2\pi}_{0}r^2\ dm
[/tex]
9. Because the integration over the whole ring had to be in terms of [itex]ds[/itex], the [itex]dm[/itex] term was decomposed as follows:
[tex]
di_{ring}=\int^{2\pi}_{0}r^2\ (\rho\ dv)
[/tex]
[tex]
di_{ring}=\rho\ \int^{2\pi}_{0}r^2\ dv
[/tex]
[tex]
di_{ring}=\rho\ \int^{2\pi}_{0}r^2\ (dz\ dr\ ds)
[/tex]
[tex]
di_{ring}=\rho\ r^2\ dz\ dr\ \int^{2\pi}_{0}ds
[/tex]
10. Therefore, [itex]di_{ring}=\rho\ r^2\ dz\ dr\ (2\pi\ r)[/itex], which was simply [itex]di_{ring}=2\pi\ \rho\ r^3\ dz\ dr[/itex].
11. Next, the moment inertia of each thin ring was integrated over the whole thin disk in terms of [itex]dr[/itex] to get the moment inertia of each thin disk as follows:
[tex]
di_{disk}=\int^{\sqrt{R^2-z^2}}_{0}di_{ring}
[/tex]
[tex]
di_{disk}=\int^{\sqrt{R^2-z^2}}_{0}2\pi\ \rho\ r^3\ dz\ dr
[/tex]
[tex]
di_{disk}=2\pi\ \rho\ dz\ \int^{\sqrt{R^2-z^2}}_{0}r^3\ dr
[/tex]
[tex]
di_{disk}=2\pi\ \rho\ dz\ \left (\frac{1}{4}\ \left \vert r^4 \right \vert ^{\sqrt{R^2-z^2}}_{0}\right )
[/tex]
[tex]
di_{disk}=\frac{1}{2}\ \pi\ \rho\ dz\ \left(R^2-z^2\right)^2
[/tex]
12. Finally, the moment inertia of the upper half of the sphere could be found by integrating the moment inertia of each thin disk over the whole length [itex]R[/itex] along the [itex]+z[/itex]-axis as follows:
[tex]
I_{\frac{1}{2}solid\ sphere}=\int^{R}_{0}di_{disk}
[/tex]
[tex]
I_{\frac{1}{2}solid\ sphere}=\int^{R}_{0}\frac{1}{2}\ \pi\ \rho\ \left(R^2-z^2\right)^2\ dz
[/tex]
[tex]
I_{\frac{1}{2}solid\ sphere}=\frac{1}{2}\pi\ \rho\ \int^{R}_{0}\left(R^4-2R^2\ z^2+z^4\right)\ dz
[/tex]
[tex]
I_{\frac{1}{2}solid\ sphere}=\frac{1}{2}\ \pi\ \rho\ \left \vert R^4\ z-\frac{2}{3}\ R^2\ z^3+\frac{1}{5}\ z^5\ \right \vert ^{R}_{0}
[/tex]
[tex]
I_{\frac{1}{2}solid\ sphere}=\frac{1}{2}\ \pi\ \rho\ \left(R^5-\frac{2}{3}\ R^5+\frac{1}{5}\ R^5\right)
[/tex]
[tex]
I_{\frac{1}{2}solid\ sphere}=\frac{1}{2}\ \pi\ \rho\ \left(\frac{8}{15}\ R^5\right)
[/tex]
[tex]
I_{\frac{1}{2}solid\ sphere}=\frac{4}{15}\ \pi\ \rho\ R^5
[/tex]
13. To find the moment inertia of the whole solid sphere, the moment inertia of the upper half of the solid sphere was multiplied by two as follows:
[tex]
I_{solid\ sphere}=2I_{\frac{1}{2}solid\ sphere}
[/tex]
[tex]
I_{solid\ sphere}=2\left(\frac{4}{15}\ \pi\ \rho\ R^5\right)
[/tex]
[tex]
I_{solid\ sphere}=\frac{8}{15}\ \pi\ \rho\ R^5
[/tex]
14. As the last step, the moment inertia of the whole solid sphere was given in terms of its mass [itex]M[/itex]. The relation of [itex]M=\rho\ V[/itex] where [itex]V=\frac{4}{3}\pi\ R^3[/itex] was substituted as follows:
[tex]
I_{solid\ sphere}=\frac{8}{15}\ \pi\ \rho\ R^5
[/tex]
[tex]
I_{solid\ sphere}=\frac{8}{15}\ \pi\ \left(\frac{3}{4}\ \frac{1}{\pi}\ \frac{M}{R^3}\right)\ R^5
[/tex]
[tex]
I_{solid\ sphere}=\frac{2}{5}\ M\ R^2
[/tex]
Yup, I got the right result!
Now since I was able to calculate the moment inertia of a solid sphere with the idea of [itex]dx[/itex] as a very very small quantity, finally I tried to calculate the moment inertia of a hollow sphere (a thin spherical shell), which was actually only a very very thin plate as the surface of a hollow sphere, [itex]\left (\frac{2}{3}\ M\ R^2\right )[/itex] as follows:
1. I used a 3-D Cartesian coordinate and I put a hollow sphere having a radius [itex]R[/itex], a total mass [itex]M[/itex], and a uniform mass distribution, centered at the origin and rotated about the [itex]z[/itex]-axis.
2. Because the solid sphere was symmetric with respect to the [itex]x-y[/itex] plane, I only considered the upper half of it, which was located in the [itex]+z[/itex] direction.
3. I thinly sliced the upper half part of the sphere parallel with the [itex]x-y[/itex] plane. This resulted in many circular rings with varying diameter depending on their distances from the [itex]x-y[/itex] plane. One such ring was depicted in Figure 4 as a red rectangle. This particular ring, which was as thin as [itex]dz[/itex], was located at distance [itex]z[/itex] units from the [itex]x-y[/itex] plane, and therefore it has a diameter of [itex]\sqrt{R^2-z^2}[/itex].
4. Because the thin rings were not point objects, I could not apply the formula yet. Therefore, I took one such thin ring and started to thinly slice it into many small plates of size [itex]dz[/itex] x [itex]ds[/itex] as depicted in Figure 5.
5. Because the plates were very very small, they can be regarded as point objects. Therefore, I could apply the formula now.
6. First, the formula called for the mass of the point object. The mass of the hollow sphere could be related to its surface area as [itex]M=\rho\ A[/itex]. Because the hollow sphere had a uniform mass distribution, the plates had very very small masses [itex]dm[/itex] that could be related to its very very small areas [itex]da[/itex], where [itex]da=(dz)(ds)[/itex], as [itex]dm=\rho\ da[/itex].
7. Looking at Figure 5, [itex]di_{plate}=(\sqrt{R^2-z^2})^2\ dm[/itex]. To get the total moment inertia for the thin ring, the moment inertia of each plate along the ring was summed up as follows:
[tex]
di_{ring}=\int^{2\pi}_{0}di_{plate}
[/tex]
[tex]
di_{ring}=\int^{2\pi}_{0}(R^2-z^2)\ dm
[/tex]
8. Because the integration over the whole ring had to be in terms of [itex]ds[/itex], the [itex]dm[/itex] term was decomposed as follows:
[tex]
di_{ring}=\int^{2\pi}_{0}(R^2-z^2)(\rho\ da)
[/tex]
[tex]
di_{ring}=\rho\ \int^{2\pi}_{0}(R^2-z^2)\ da
[/tex]
[tex]
di_{ring}=\rho\ \int^{2\pi}_{0}(R^2-z^2)(dz\ ds)
[/tex]
[tex]
di_{ring}=\rho\ (R^2-z^2)\ dz\ \int^{2\pi}_{0}\ ds
[/tex]
9. Therefore, [itex]di_{ring}=\rho\ (R^2-z^2)\ dz\ (2\pi\ \sqrt{R^2-z^2})[/itex], which was simply [itex]di_{ring}=2\pi\ \rho\ (R^2-z^2)^{\frac{3}{2}}\ dz[/itex].
10. Finally, the moment inertia of the upper half of the hollow sphere could be found by integrating the moment inertia of each thin ring over the whole length [itex]R[/itex] along the [itex]+z[/itex]-axis as follows:
[tex]
I_{\frac{1}{2}\ hollow\ sphere}=\int^{R}_{0}di_{ring}
[/tex]
[tex]
I_{\frac{1}{2}\ hollow\ sphere}=\int^{R}_{0}2\pi\ \rho\ (R^2-z^2)^{\frac{3}{2}}\ dz
[/tex]
[tex]
I_{\frac{1}{2}\ hollow\ sphere}=2\pi\ \rho\ \int^{R}_{0}(R^2-z^2)^{\frac{3}{2}}\ dz
[/tex]
It can be calculated that:
[tex]
\int (a^2-x^2)^{\frac{3}{2}}\ dx=\frac{1}{8}((5a^2-2x^2)(x\ \sqrt{a^2-x^2})+3a^4\ \arcsin(\frac{x}{a}))+c
[/tex]
Therefore, the calculation of [itex]I_{\frac{1}{2}\ hollow\ sphere}[/itex] can be continued as follows:
[tex]
I_{\frac{1}{2}\ hollow\ sphere}=2\pi\ \rho\ \frac{1}{8} \left \vert (5R^2-2z^2)(z\ \sqrt{R^2-z^2})+3R^4\ \arcsin(\frac{z}{R}) \right \vert^{R}{0}
[/tex]
[tex]
I_{\frac{1}{2}\ hollow\ sphere}=2\pi\ \rho\ \frac{1}{8}\ (3R^4\ \arcsin(\frac{R}{R}))
[/tex]
[tex]
I_{\frac{1}{2}\ hollow\ sphere}=2\pi\ \rho\ \frac{1}{8}\ (3R^4\ \frac{\pi}{2})
[/tex]
[tex]
I_{\frac{1}{2}\ hollow\ sphere}=\frac{3}{8}\ \pi^2\ \rho\ R^4
[/tex]
11. To find the moment inertia of the whole hollow sphere, the moment inertia of the upper half of the hollow sphere was multiplied by two as follows:
[tex]
I_{hollow\ sphere}=2I_{\frac{1}{2}\ hollow\ sphere}
[/tex]
[tex]
I_{hollow\ sphere}=2(\frac{3}{8}\ \pi^2\ \rho\ R^4)
[/tex]
[tex]
I_{hollow\ sphere}=\frac{3}{4}\ \pi^2\ \rho\ R^4
[/tex]
12. As the last step, the moment inertia of the whole hollow sphere was given in terms of its mass [itex]M[/itex]. The relation of [itex]M=\rho\ A[/itex] where [itex]A=4\pi\ R^2[/itex] was substituted as follows:
[tex]
I_{hollow\ sphere}=\frac{3}{4}\ \pi^2\ \rho\ R^4
[/tex]
[tex]
I_{hollow\ sphere}=\frac{3}{4}\ \pi^2\ \left (\frac{M}{4\pi\ R^2}\right )\ R^4
[/tex]
[tex]
I_{hollow\ sphere}=\frac{3}{16}\ \pi\ M\ R^2
[/tex]
I got the wrong result this time. But, why? Isn't that I had successfully applied my line of reasoning in finding the moment inertia of a solid sphere? What's wrong with my line of reasoning? Did I miss some important points in finding the moment inertia of a hollow sphere?
Thank you very much.
Eus