- #1
- 1,782
- 32
Hi,
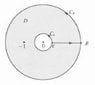
In my fluids work I have come to integrals of the type:
[tex]
\int_{0}^{\infty}\frac{e^{ikx}}{ak^{2}+bk+c}dk
[/tex]
I was thinking of evaluating this via residue calculus but I can't think of the right contour, any suggestions?
Mat
In my fluids work I have come to integrals of the type:
[tex]
\int_{0}^{\infty}\frac{e^{ikx}}{ak^{2}+bk+c}dk
[/tex]
I was thinking of evaluating this via residue calculus but I can't think of the right contour, any suggestions?
Mat
Last edited: