- #1
Saladsamurai
- 3,020
- 7
I cannot seem to figure out how to compute this dot product?!
If [itex](\nabla\times \mathbf{v})=(4z^2-2x)\hat{i}+2z\hat{k}[/itex] and [itex]d\mathbf{a}=dydz\hat{i}[/itex]
Then shouldn't the DOT PODUCT be:
[itex](\nabla\times \mathbf{v})\cdot d\mathbf{a}=(4z^2-2x)\hat{i}*dydz\hat{i}=(4z^2-2x)dydz[/itex] ?
But the book says its just [itex]4z^2dydz[/itex]
What am I doing wrong here??
Here is the original question:
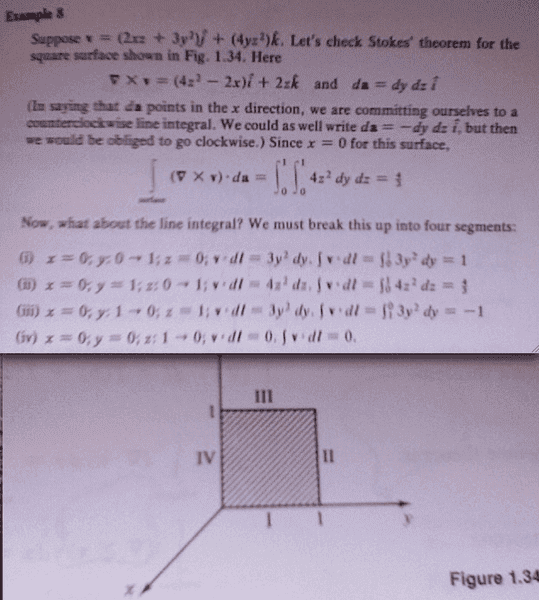
If [itex](\nabla\times \mathbf{v})=(4z^2-2x)\hat{i}+2z\hat{k}[/itex] and [itex]d\mathbf{a}=dydz\hat{i}[/itex]
Then shouldn't the DOT PODUCT be:
[itex](\nabla\times \mathbf{v})\cdot d\mathbf{a}=(4z^2-2x)\hat{i}*dydz\hat{i}=(4z^2-2x)dydz[/itex] ?
But the book says its just [itex]4z^2dydz[/itex]
What am I doing wrong here??
Here is the original question: