What is the motion of a physical pendulum with a rotating rigid body?

[fluidistic]

Homework Statement

Hello,
I've tried all I could and get very confused about how to approach the problem.
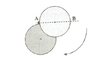
Here it comes : A disk of mass M and radius R can rotates around an axis passing by the point A without friction situated on its circumference. The disk is in a vertical plane. We let it move freely when point B is at the same high than point A and point B is opposed to point A. (See figure to get clarification).

a)What is the tangential acceleration of point B just after the disk has been released?
b)What is its velocity when it pass by its lower point?
c)What is the acceleration of point B in function of the disk's position.

2. The attempt at a solution
First I drew the applied forces, which I think are the normal and the weight. The weight is applied in the center of mass of the disk that is in its center while the normal force is applied at point A and is constantly changing so I don't even know its magnitude nor its direction.
I believe that from a formula, $$\vec v_{B}=\vec{\alpha}\wedge \vec{r_{B}}+\vec{a}_{CM}$$.
I consider the origin of the system as being the point A. I want to know $$\vec{a}_{CM}$$.
From Newton, $$\frac{d\vec{P}}{dt}=m\vec{a}_{CM}=\vec{F}_e=Mgj+\vec{N}\Leftrightarrow \vec{a}_{CM}=gj+\frac{\vec N}{M}$$.
Thanks to the right hand rule, I got that $$\vec{\alpha}=-\alpha k$$.
So $$\vec v_{B}=-\alpha k \wedge Ri+gj+\frac{\vec N }{M}$$. The only unknowns remaining are the normal force and the angular acceleration. I've no clue how to find them.
I also tried other things, like writing down $$\frac{d\vec L}{dt}$$ or even $$\vec L= \vec L_o + \vec L_s$$ where $$\vec L_o$$ is $$L$$ orbital and $$\vec L_s$$ is $$L$$ spin. I calculated $$L_s$$ to be worth $$\frac{3MR^2\vec{\omega}}{4}$$ and I believe that $$L_o=\vec R_{CM} \wedge mv_{CM}$$ and I have much more unknowns including $$mv_{CM}$$ which seems at least as hard as to find $$v_{B}$$...
I must be missing many things. Can you help me please?

 

[Doc Al]

Keep it simple. Start by using Newton's 2nd law applied to rotation to find the angular acceleration:
$$\tau = I \alpha$$

 

[fluidistic]

Thank you very much for your help Doc Al.
Your $$\tau=I\alpha$$ is my $$\frac{d\vec L}{dt}$$ and I sadly didn't realize it was worth $$I\alpha$$.
I wrote $$\frac{d\vec L}{dt}=\vec P \wedge \vec r + \vec N \wedge \vec r = -Mg\frac{R}{2}k$$.
By luck I calculated $$L_s$$. Derivating it I obtain $$I\alpha$$ : $$\frac{3MR^2\vec{\alpha}}{4}$$. (I didn't realize $$L_o$$ would be equal to 0. That's because the motion is a pure rotation so there is no translation I think).
So $$\vec \tau = -\frac{3MR^2 \alpha}{4}k$$.
Right now I don't see how I can calculate $$\alpha$$. A little help would be appreciated
I'll try to do the next exercise alone.

 

[Doc Al]

So $$\vec \tau = -\frac{3MR^2 \alpha}{4}k$$.

How did you calculate the rotational inertia?

Right now I don't see how I can calculate $$\alpha$$.

Figure out the torque.

 

[fluidistic]

To calculate $$I$$ I used Steiner's theorem.
If I'm not wrong the moment of inertia of a disk rotating about an axis passing by its center is $$\frac{MR^2}{2}$$. Using Steiner we have that $$I_{A}=I_G+Md^2$$.
I made an error, you're right : $$Md^2=4MR^2$$.
So $$I=\frac{9MR^2}{2}$$.

Figure out the torque.

Ok I will. A bit later though...
Thank you.

 

[Doc Al]

I made an error, you're right : $$Md^2=4MR^2$$.

This is still incorrect. (Where are you getting the 4?)

 

[fluidistic]

Sorry my fault, I took 2R instead of R the distance between the CM and A. I got confused with point B... nevermind.
So $$I=\frac{3MR^2}{2}$$.

 

[Doc Al]

Good. Now find the torque.

 

[fluidistic]

$$\vec \tau = \vec r \wedge \vec F=Ri \wedge -Mgj=-RMgk$$ which is worth $$\frac{3MR^2 \vec \alpha }{2} \Leftrightarrow \vec \alpha=-\frac{2}{3R}k$$.
Later I'll answer to question a).

 

[Doc Al]

Looks OK, but you dropped off a g.

 

[fluidistic]

Oops. Ok now I get it right.

As $$\vec a_T= \vec \alpha r$$ I get that $$\vec a_T=\frac{4g}{3}j$$ when the disk has just been released. Is it right? (I guess no because I should get $$\vec a_T=-\frac{4g}{3}j$$...)

 

[Doc Al]

As $$\vec a_T= \vec \alpha r$$ I get that $$\vec a_T=\frac{4g}{3}j$$ when the disk has just been released. Is it right? (I guess no because I should get $$\vec a_T=-\frac{4g}{3}j$$...)

Good. Don't get hung up on the sign of the acceleration, that just depends on your convention. It's rotating clockwise, so the tangential acceleration is down and thus negative.

 

[fluidistic]

Thanks for all your help Doc Al. I also think that in the last formula, it is $$\vec g$$ so it is -gj.
Sorry for all the time I took to solve the problem and all the errors. I might say it's in part due to the fact that temperature here is very hot today, more than 35°C and humidity is high so it's uncomfortable to think well.

 

[fluidistic]

For the b) I have a question.
My attempt would be to find out $$\omega (t)$$ which I think is simply $$\int \alpha dt$$.
So that I can use the relation $$\vec v_B=\vec r_B \wedge \vec \omega_B$$. But I doubt it will help since I don't know at what time it will reach its lower position.
Maybe considering the energy of the body? It is a pure rotation so $$E=\frac{I\omega ^2}{2}$$ but it makes no sense at all... $$E_{initial}=E_{final}$$ but $$\omega$$ changes and $$I$$ is a constant so it is not possible. I don't understand why I can't apply the law of conservation of energy. Can you (or someone else) help me?

 

[Doc Al]

For the b) I have a question.
My attempt would be to find out $$\omega (t)$$ which I think is simply $$\int \alpha dt$$.

That method is the hard way. Note that α is not a constant.

Maybe considering the energy of the body? It is a pure rotation so $$E=\frac{I\omega ^2}{2}$$ but it makes no sense at all... $$E_{initial}=E_{final}$$ but $$\omega$$ changes and $$I$$ is a constant so it is not possible. I don't understand why I can't apply the law of conservation of energy.

Mechanical energy is conserved. Why do you think you can't apply it?

 

[fluidistic]

Mechanical energy is conserved. Why do you think you can't apply it?

If it is conserved then $$E=\frac{I\omega ^2}{2}$$ is not true because as $$I$$ is a constant, it implies that $$\omega$$ is also a constant, but as you said $$\alpha$$ is not constant and thus $$\omega$$ also varies.
So as $$E$$ is a constant in this problem, it must equals something else than $$\frac{I\omega ^2}{2}$$ but as the motion is a pure rotation I must say I'm missing something.
Thanks once again. You've been very useful to me.

 

[Doc Al]

So as $$E$$ is a constant in this problem, it must equals something else than $$\frac{I\omega ^2}{2}$$ but as the motion is a pure rotation I must say I'm missing something.

That's just the kinetic energy, not the total energy. It's the total mechanical energy that's conserved. (Don't forget gravity!)

 

[fluidistic]

That's just the kinetic energy, not the total energy. It's the total mechanical energy that's conserved. (Don't forget gravity!)

Ahaha, I missed the obvious.
One last question and I get the answer to part b)
From the conservation of the mechanical energy I got that $$\omega _f=\sqrt{\omega _i^2+ \frac{4MgR}{I}}$$. How do I get $$\omega _i$$?
Is it from $$\alpha _i$$ that I already got? If so, I'm lost.

 

[Doc Al]

From the conservation of the mechanical energy I got that $$\omega _f=\sqrt{\omega _i^2+ \frac{4MgR}{I}}$$.

Where did the 4 come from?

How do I get $$\omega _i$$?

Assume the disk is released from rest.

 

[fluidistic]

Assume the disk is released from rest.

Oh... yes of course. Second time I make a similar error. The angular acceleration can be different from 0 rad/s^2 while the angular velocity is worth 0 rad/s.

Where did the 4 come from?

The difference of height between point B final position versus point B initial position is a diameter that is twice the radius (2R). So I took the origin of the system as being point B final position.
Thus $$E_i=\frac{I\omega _i^2}{2}+Mg2R$$.
$$E_f=\frac{I\omega _f^2}{2}$$.
Hence $$\frac{I\omega _i^2}{2}+Mg2R=\frac{I\omega _f^2}{2} \Leftrightarrow I\omega _f=I \omega _i^2+4MgR$$. From it I reached what I wrote.
(I believe that point B reaches the bottom of the picture I uploaded, thus the difference of height of point B is 2R.)

 

[Doc Al]

The difference of height between point B final position versus point B initial position is a diameter that is twice the radius (2R).

That's true, but what matters is the change in gravitational PE, which depends on the change in position of the center of mass, not point B.

 

[fluidistic]

That's true, but what matters is the change in gravitational PE, which depends on the change in position of the center of mass, not point B.

Thank you Doc Al, you're like a teacher.
Now I understand : when I have to consider the whole system which is a disk in this case, I must think about it as if it was only a point : the center of mass when it comes to potential energy.
I got the answer. (change the 4 I had for a 2).

 

[fluidistic]

Now part c)
When they say the "disk's position" thanks to you I think they talk about its center of mass in my case.
I feel very unsure about how to proceed. I think that the acceleration of point B is the acceleration of the center of mass plus the tangential acceleration of point B or something like that. Even knowing this, I need a push to start to write down equations.
It probably has to see with the solution of the differential equation of the motion of a pendulum. The solution is $$a(t)=-A\omega ^2 \sin (\omega t + \phi)$$ with $$\omega=\sqrt{\frac{g}{R}}$$ if I remember well but wouldn't it be the tangential acceleration and not the centripetal one?

 

[Doc Al]

To specify position, since there's only one degree of freedom, I would use the angle that line A-B makes with the horizontal. Find the tangential and radial components of the acceleration of B as a function of that angle. (The radial/centripetal acceleration will depend on the speed.)

 

[LogicalTime]

check out this MIT lecture at about 36:30, he does the calculation for a hula hoop:

http://ocw.mit.edu/OcwWeb/Physics/8-01Physics-IFall1999/VideoLectures/detail/embed21.htm

 

[fluidistic]

Thanks to both. (Logical time, did you forgot to give the link?)
Anyway I'll retry to do it entirely.
I'm also not convinced that the final mechanical energy is worth $$\frac{I\omega _f ^2}{2}$$ since the center of mass has a velocity so I should add $$+\frac{Mv_{CM}^2}{2}$$ which complicates things. Anyway I'll try alone everything from 0.

 

[LogicalTime]

yep, the link is there now ;)

 

[Doc Al]

I'm also not convinced that the final mechanical energy is worth $$\frac{I\omega _f ^2}{2}$$ since the center of mass has a velocity so I should add $$+\frac{Mv_{CM}^2}{2}$$ which complicates things.

As long as you use the rotational inertia about the axis, then the total KE is given by $$I\omega^2/2$$. You'd only add the KE of the center of mass as a separate term if you use I about the center of mass when calculating the rotational KE.

 

[fluidistic]

yep, the link is there now ;)

Thank you, I will check it out.

As long as you use the rotational inertia about the axis, then the total KE is given by $$I\omega^2/2$$. You'd only add the KE of the center of mass as a separate term if you use I about the center of mass when calculating the rotational KE.

Thanks once again.