- #1
Tac-Tics
- 816
- 7
I'm trying to follow an argument in Pauling's Introduction to QM w/applications to chemistry. He is a bit hand-wavy at parts, and I wanted to see if anyone could clarify.
So we start off with the time-dependent Schrodinger equation. He makes the assumption that [tex]\Psi[/tex], our wavefunction, can be decomposed as the product of two functions, [tex]\psi[/tex], a function of position, and [tex]\phi[/tex], a function of time.
My first question is why can he make that assumption. From the look of it, it seems he's assuming that the position determines the magnitude of the wavefunction and the time determines the complex angle. However, he doesn't state it directly and he doesn't explain why that assumption is legitimate. My only guess is that since we're dealing with a single electron, there is no possibility for it to interfere with anything.
So, with that assumption, he continues to simplify the schrodinger equation into a more manageable form. He reduces it to
[tex]\frac{d^2\psi}{dx^2} = \frac{2m}{\hbar}(V(x) - W)\psi(x)[/tex]
Where W is an arbitrary energy level.
We assume that V(x) is a function such that V(x) grows to infinity at either infinity. We then choose the largest and greatest values for x, called a and b, such that V(a) = W and V(b) = W. That is, where the energy potential equals the energy level.
It's clear from the modified schrodinger equation that the concavity of [tex]\psi[/tex] will reflect the value of psi outside the range [b, a]. That is, if [tex]\psi(x)[/tex] is positive for any x > a, then [tex]\psi[/tex] is concave up at all points x > a.
(However, if I'm right in assuming that [tex]\psi[/tex] is the magnitude of the wavefunction, it would *always* be positive... but Pauling says [tex]\psi[/tex] can be negative at this point...)
So that's as far as I understand well. The next part, he loses me.
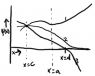
Choose any point c and a value for [tex]\frac{d\psi}{dx}(c)[/tex]. He claims that having chosen these values, [tex]\psi[/tex] will either diverge to infinity, negative infinity, or approach zero (at least... that seems to be his argument).
I can't quite understand his reasoning for this. He gives three examples. In one, the derivative is positive at c, and the function diverges to infinity. In the next, the derivative is negative and fall to negative infinity. The last looks as if the derivative were closer to zero (he doesn't specify the value required), and the function asymptotically approaches zero.
It's frustrating I can't follow his argument, because the idea is fascinating (READ: he has a really cool diagram in the next section that essentially explains why electrons are "bound" to atoms... where the energy levels above a certain threshold (the ionization energy?) become continuous).
Anyway, if anyone could offer some help, I'd be super greatful.
So we start off with the time-dependent Schrodinger equation. He makes the assumption that [tex]\Psi[/tex], our wavefunction, can be decomposed as the product of two functions, [tex]\psi[/tex], a function of position, and [tex]\phi[/tex], a function of time.
My first question is why can he make that assumption. From the look of it, it seems he's assuming that the position determines the magnitude of the wavefunction and the time determines the complex angle. However, he doesn't state it directly and he doesn't explain why that assumption is legitimate. My only guess is that since we're dealing with a single electron, there is no possibility for it to interfere with anything.
So, with that assumption, he continues to simplify the schrodinger equation into a more manageable form. He reduces it to
[tex]\frac{d^2\psi}{dx^2} = \frac{2m}{\hbar}(V(x) - W)\psi(x)[/tex]
Where W is an arbitrary energy level.
We assume that V(x) is a function such that V(x) grows to infinity at either infinity. We then choose the largest and greatest values for x, called a and b, such that V(a) = W and V(b) = W. That is, where the energy potential equals the energy level.
It's clear from the modified schrodinger equation that the concavity of [tex]\psi[/tex] will reflect the value of psi outside the range [b, a]. That is, if [tex]\psi(x)[/tex] is positive for any x > a, then [tex]\psi[/tex] is concave up at all points x > a.
(However, if I'm right in assuming that [tex]\psi[/tex] is the magnitude of the wavefunction, it would *always* be positive... but Pauling says [tex]\psi[/tex] can be negative at this point...)
So that's as far as I understand well. The next part, he loses me.
Choose any point c and a value for [tex]\frac{d\psi}{dx}(c)[/tex]. He claims that having chosen these values, [tex]\psi[/tex] will either diverge to infinity, negative infinity, or approach zero (at least... that seems to be his argument).
I can't quite understand his reasoning for this. He gives three examples. In one, the derivative is positive at c, and the function diverges to infinity. In the next, the derivative is negative and fall to negative infinity. The last looks as if the derivative were closer to zero (he doesn't specify the value required), and the function asymptotically approaches zero.
It's frustrating I can't follow his argument, because the idea is fascinating (READ: he has a really cool diagram in the next section that essentially explains why electrons are "bound" to atoms... where the energy levels above a certain threshold (the ionization energy?) become continuous).
Anyway, if anyone could offer some help, I'd be super greatful.