- #1
uno4short
- 7
- 0
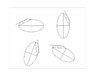
Equation for Ellipse from a chord -- no other parameters!
Known conditions are:
End points of the chord intersect the major and minor axis.
Proximity to nearest parallel tangent.
Known (hypothetical) values are:
The length of the chord is 10.
The chord is 1.25 from the nearest parallel tangent.
This is where I get hung. I cannot seem to find any that apply. So...
The goal -- "find equation" to solve any *one* of the following:
What are the (x,y) and (x',y') coordinate values for the foci on the ellipse?
What is the (x,y) coordinate values for the center of the ellipse relative to the chord?
What is the angle from the center of the ellipse to the center of that chord relative to the major or minor axis?
What is the angle of the chord relative to the major or minor axis?
What is the length of either the major or minor axis?
Basicly, I am looking to find any defining value anywhere on that ellipse so that I can make use of standard equations.
This is one of many sample diagrams I've used to help analize the problem:
http://img124.imagevenue.com/img.php?image=29019_ChordEllipse_122_392lo.jpg
It is clear that, given the length of the chord and the distance to the parallel tangent, there can be only one solution (excluding the mirror image of the ellipse which for all practical purposes equates to "one and the same").
I have no values for a or b or C or anything that I can use to relate back to the standard equations. No line slopes to work with, so where can I go from here?
Homework Statement
Known conditions are:
End points of the chord intersect the major and minor axis.
Proximity to nearest parallel tangent.
Known (hypothetical) values are:
The length of the chord is 10.
The chord is 1.25 from the nearest parallel tangent.
Homework Equations
This is where I get hung. I cannot seem to find any that apply. So...
The goal -- "find equation" to solve any *one* of the following:
What are the (x,y) and (x',y') coordinate values for the foci on the ellipse?
What is the (x,y) coordinate values for the center of the ellipse relative to the chord?
What is the angle from the center of the ellipse to the center of that chord relative to the major or minor axis?
What is the angle of the chord relative to the major or minor axis?
What is the length of either the major or minor axis?
Basicly, I am looking to find any defining value anywhere on that ellipse so that I can make use of standard equations.
The Attempt at a Solution
This is one of many sample diagrams I've used to help analize the problem:
http://img124.imagevenue.com/img.php?image=29019_ChordEllipse_122_392lo.jpg
It is clear that, given the length of the chord and the distance to the parallel tangent, there can be only one solution (excluding the mirror image of the ellipse which for all practical purposes equates to "one and the same").
I have no values for a or b or C or anything that I can use to relate back to the standard equations. No line slopes to work with, so where can I go from here?
Last edited: