- #1
- 2,567
- 4
The main problem I have with this question is just the wording:
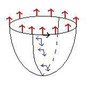
If [itex]M[/itex] is an oriented manifold by means of the restriction of the form [itex]dx \wedge dy[/itex], describe explicitly the induced orientation on [itex]\partial M[/itex] -- i.e. clockwise or counterclockwise in the plane [itex]z = 1[/itex].
I don't understand the underlined part. Perhaps it means that the orientation for each [itex](x, y) \in M[/itex], [itex]\mu _{(x, y)}[/itex] is the one such that [itex]dx \wedge dy (x, y)[/itex] is the volume element of [itex]M_{(x, y)}[/itex] determined by the standard inner product and orientation [itex]\mu _{(x, y)}[/itex]. Am I interpreting it correctly, or am I reading much more into what's actually said there, and if it's the latter, what is the correct interpretation? Thanks.
If [itex]M[/itex] is an oriented manifold by means of the restriction of the form [itex]dx \wedge dy[/itex], describe explicitly the induced orientation on [itex]\partial M[/itex] -- i.e. clockwise or counterclockwise in the plane [itex]z = 1[/itex].
I don't understand the underlined part. Perhaps it means that the orientation for each [itex](x, y) \in M[/itex], [itex]\mu _{(x, y)}[/itex] is the one such that [itex]dx \wedge dy (x, y)[/itex] is the volume element of [itex]M_{(x, y)}[/itex] determined by the standard inner product and orientation [itex]\mu _{(x, y)}[/itex]. Am I interpreting it correctly, or am I reading much more into what's actually said there, and if it's the latter, what is the correct interpretation? Thanks.