- #1
Physics lover
- 249
- 25
- Homework Statement
- The question is in Attempt at a solution.
- Relevant Equations
- x=tanA/b
Homework Statement: The question is in Attempt at a solution.
Homework Equations: x=tanA/b
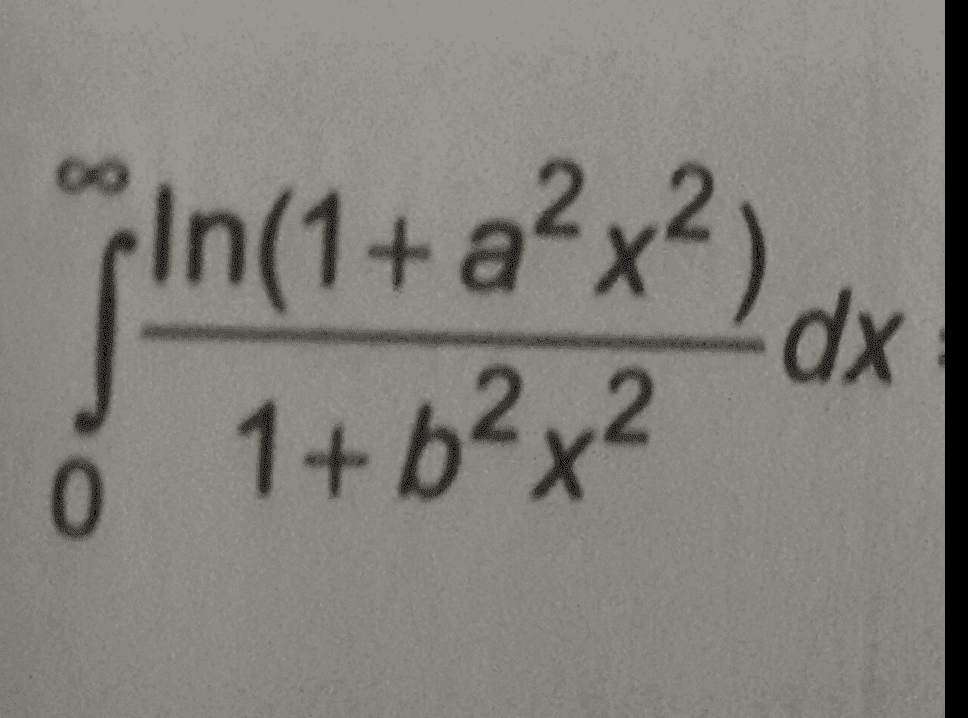
I tried by substituting x=tanA/b but it did'nt helped.Now I cannot think of any other thing to do.Help.
Homework Equations: x=tanA/b
I tried by substituting x=tanA/b but it did'nt helped.Now I cannot think of any other thing to do.Help.
