- #1
BlackMamba
- 187
- 0
I think I have a trick question here. The section we are covering is addition of vectors by means of components. Here is my problem:
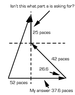
You are on a treasure hunt and your map says, "Walk due east for 52 paces, then walk 26.6 degrees north of west for 42 paces, and finally walk due north for 25 paces."
a) What is the magnitude of the component of your displacement in the direction due north?
b) What is the magnitude of the component of your displacement in the direction of due east?
I believe I figured out part (b) correctly getting an answer of 37.6 paces. But isn't the answer for part (a) already given? Wouldn't the answer to part (a) just be 25 paces??
My drawing is attached.
You are on a treasure hunt and your map says, "Walk due east for 52 paces, then walk 26.6 degrees north of west for 42 paces, and finally walk due north for 25 paces."
a) What is the magnitude of the component of your displacement in the direction due north?
b) What is the magnitude of the component of your displacement in the direction of due east?
I believe I figured out part (b) correctly getting an answer of 37.6 paces. But isn't the answer for part (a) already given? Wouldn't the answer to part (a) just be 25 paces??
My drawing is attached.