- #1
Math Amateur
Gold Member
MHB
- 3,990
- 48
I am reading Loring W.Tu's book: "An Introduction to Manifolds" (Second Edition) ...
I need help in order to fully understand Tu's Proposition 3.21 ... ...
Proposition 3.21 reads as follows:

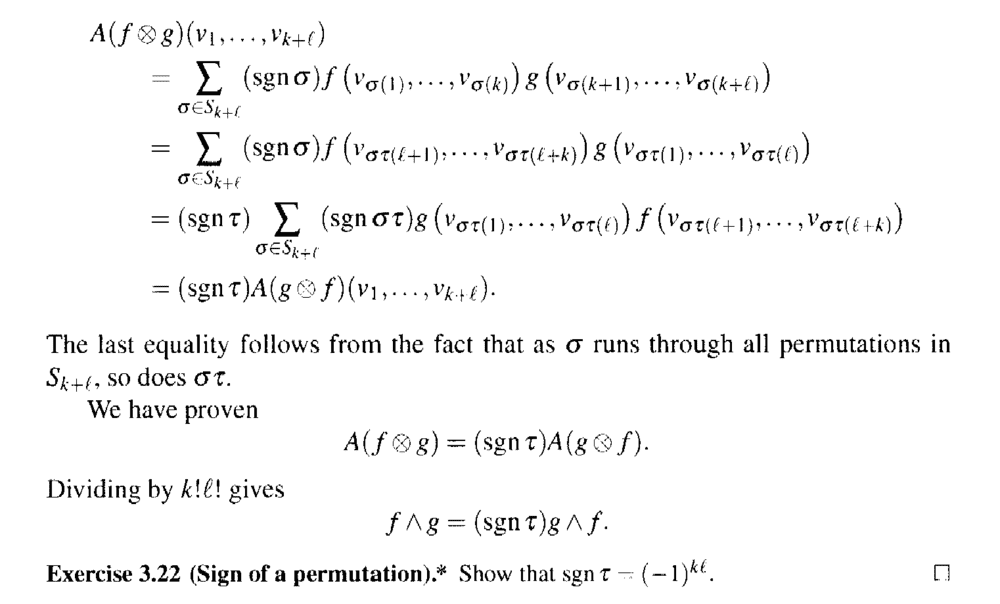
In the above proof by Tu we read the following:
" ... ...
... ##= \sum_{ \sigma_{ k + l } } ( \text{ sgn } \sigma ) f( v_{ \sigma \tau (l+1) }, \cdot \cdot \cdot , v_{ \sigma \tau (l+k) }) g ( v_{ \sigma \tau (1) }, \cdot \cdot \cdot , v_{ \sigma \tau (l) })####= ( \text{ sgn } \tau ) \sum_{ \sigma_{ k + l } } ( \text{ sgn } \sigma \tau ) g ( v_{ \sigma \tau (1) }, \cdot \cdot \cdot , v_{ \sigma \tau (l) }) f( v_{ \sigma \tau (l+1) }, \cdot \cdot \cdot , v_{ \sigma \tau (l+k) })##
... ... ... "
Can someone please explain/demonstrate how/why we have that##\sum_{ \sigma_{ k + l } } ( \text{ sgn } \sigma ) f( v_{ \sigma \tau (l+1) }, \cdot \cdot \cdot , v_{ \sigma \tau (l+k) }) g ( v_{ \sigma \tau (1) }, \cdot \cdot \cdot , v_{ \sigma \tau (l) })####= ( \text{ sgn } \tau ) \sum_{ \sigma_{ k + l } } ( \text{ sgn } \sigma \tau ) g ( v_{ \sigma \tau (1) }, \cdot \cdot \cdot , v_{ \sigma \tau (l) }) f( v_{ \sigma \tau (l+1) }, \cdot \cdot \cdot , v_{ \sigma \tau (l+k) })##Help will be much appreciated ... ...
Peter
============================================================================
*** EDIT ***I have been reflecting on my question/problem in the above post ... ... and I think I have resolved the problem ...Since ##\text{ sgn } \tau## is ##+1## or ##-1## then ##\text{ sgn } \tau \tau = 1## ...Therefore we have##\text{ sgn } \sigma = \text{ sgn } \sigma \tau \tau = \text{ sgn } \tau \text{ sgn } \sigma \tau##which answers the question ... ...Is that correct ... ... ?
Peter
I need help in order to fully understand Tu's Proposition 3.21 ... ...
Proposition 3.21 reads as follows:
In the above proof by Tu we read the following:
" ... ...
... ##= \sum_{ \sigma_{ k + l } } ( \text{ sgn } \sigma ) f( v_{ \sigma \tau (l+1) }, \cdot \cdot \cdot , v_{ \sigma \tau (l+k) }) g ( v_{ \sigma \tau (1) }, \cdot \cdot \cdot , v_{ \sigma \tau (l) })####= ( \text{ sgn } \tau ) \sum_{ \sigma_{ k + l } } ( \text{ sgn } \sigma \tau ) g ( v_{ \sigma \tau (1) }, \cdot \cdot \cdot , v_{ \sigma \tau (l) }) f( v_{ \sigma \tau (l+1) }, \cdot \cdot \cdot , v_{ \sigma \tau (l+k) })##
... ... ... "
Can someone please explain/demonstrate how/why we have that##\sum_{ \sigma_{ k + l } } ( \text{ sgn } \sigma ) f( v_{ \sigma \tau (l+1) }, \cdot \cdot \cdot , v_{ \sigma \tau (l+k) }) g ( v_{ \sigma \tau (1) }, \cdot \cdot \cdot , v_{ \sigma \tau (l) })####= ( \text{ sgn } \tau ) \sum_{ \sigma_{ k + l } } ( \text{ sgn } \sigma \tau ) g ( v_{ \sigma \tau (1) }, \cdot \cdot \cdot , v_{ \sigma \tau (l) }) f( v_{ \sigma \tau (l+1) }, \cdot \cdot \cdot , v_{ \sigma \tau (l+k) })##Help will be much appreciated ... ...
Peter
============================================================================
*** EDIT ***I have been reflecting on my question/problem in the above post ... ... and I think I have resolved the problem ...Since ##\text{ sgn } \tau## is ##+1## or ##-1## then ##\text{ sgn } \tau \tau = 1## ...Therefore we have##\text{ sgn } \sigma = \text{ sgn } \sigma \tau \tau = \text{ sgn } \tau \text{ sgn } \sigma \tau##which answers the question ... ...Is that correct ... ... ?
Peter
Attachments
Last edited:



