- #1
Mr.Tibbs
- 24
- 0
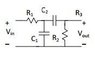
For the circuit shown below with R1 = 100 Ω, R2 = 3 kΩ, C1
= 100 pF and C2 = 139 nF, plot the output voltage as a function of time for 2 cycles
when the input voltage is vin(t) = 5V+1Vsin(2[itex]\pi[/itex]ft) where (a) f=1 Hz and (b) f=1 MHz
2. Homework Equations
voltage divider:
[itex]\frac{R_{2}}{R_{1}+R_{2}}[/itex]
3. Attempt
It's been a while since I've done this so this is what I have.
To solve for the voltage across capacitor one first I used a voltage divider:
v[itex]_{1}[/itex] = [itex]\frac{1}{1+j\omega R_{1}C_{1}}[/itex] = 1-6.283E-8j
I then rinse and repeated for the output.
v[itex]_{out}[/itex] = v[itex]_{1}[/itex] [itex]\frac{j\omega R_{2}C_{2}}{1+j\omega R_{2}C_{2}}[/itex] = 6.86498E-6+j2.62E-3
after this I'm lost because I don't even know if my output is correct. I understand that there can be a real component at low frequencies but I don't think my answer is correct to begin with. . .
= 100 pF and C2 = 139 nF, plot the output voltage as a function of time for 2 cycles
when the input voltage is vin(t) = 5V+1Vsin(2[itex]\pi[/itex]ft) where (a) f=1 Hz and (b) f=1 MHz
2. Homework Equations
voltage divider:
[itex]\frac{R_{2}}{R_{1}+R_{2}}[/itex]
3. Attempt
It's been a while since I've done this so this is what I have.
To solve for the voltage across capacitor one first I used a voltage divider:
v[itex]_{1}[/itex] = [itex]\frac{1}{1+j\omega R_{1}C_{1}}[/itex] = 1-6.283E-8j
I then rinse and repeated for the output.
v[itex]_{out}[/itex] = v[itex]_{1}[/itex] [itex]\frac{j\omega R_{2}C_{2}}{1+j\omega R_{2}C_{2}}[/itex] = 6.86498E-6+j2.62E-3
after this I'm lost because I don't even know if my output is correct. I understand that there can be a real component at low frequencies but I don't think my answer is correct to begin with. . .