- #1
- 1,104
- 960
Imagine a right circular cone with smooth surface. The cone is stated such that its axis is parallel to the standard gravitational field g. And you have a piece a thin homogeneous chain. Then you connect the tips of the chain to obtain a loop. You put this loop on the cone:
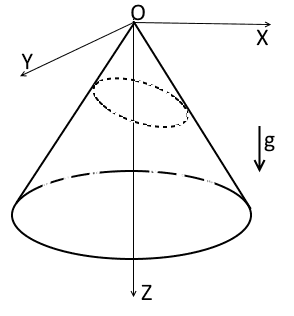
It is clear, there is a state such that whole the loop forms the circle and rests in the horizontal plane. Are there another equilibriums? How many equilibriums does this system have?
(I know the answer and the solution. It is just for those who doesn't know what to do on weekends evenings :) I enjoyed solving this problem and share it with PF :)
It is clear, there is a state such that whole the loop forms the circle and rests in the horizontal plane. Are there another equilibriums? How many equilibriums does this system have?
(I know the answer and the solution. It is just for those who doesn't know what to do on weekends evenings :) I enjoyed solving this problem and share it with PF :)