- #1
~angel~
- 150
- 0
Hey...I'm having a lot of trouble with a few questions for university physics.
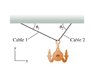
1. A chandelier with mass m is attached to the ceiling of a large concert hall by two cables. Because the ceiling is covered with intricate architectural decorations (not indicated in the figure, which uses a humbler depiction), the workers who hung the chandelier couldn't attach the cables to the ceiling directly above the chandelier. Instead, they attached the cables to the ceiling near the walls. Cable 1 has tension T_1 and makes an angle of theta_1 with the ceiling. Cable 2 has tension T_2 and makes an angle of theta_2 with the ceiling.
Find an expression for T_1, the tension in cable 1, that does not depend on T_2.Express your answer in terms of some or all of the variables m, theta_1, and theta_2, as well as the magnitude of the acceleration due to gravity g.
The image of the chandelier is below.
I would prefer if people could type the working out of the solution as well.
Thank you.
1. A chandelier with mass m is attached to the ceiling of a large concert hall by two cables. Because the ceiling is covered with intricate architectural decorations (not indicated in the figure, which uses a humbler depiction), the workers who hung the chandelier couldn't attach the cables to the ceiling directly above the chandelier. Instead, they attached the cables to the ceiling near the walls. Cable 1 has tension T_1 and makes an angle of theta_1 with the ceiling. Cable 2 has tension T_2 and makes an angle of theta_2 with the ceiling.
Find an expression for T_1, the tension in cable 1, that does not depend on T_2.Express your answer in terms of some or all of the variables m, theta_1, and theta_2, as well as the magnitude of the acceleration due to gravity g.
The image of the chandelier is below.
I would prefer if people could type the working out of the solution as well.
Thank you.