Trigonometry to Find Stronghold in Minecraft

[Nexor]

Cheating in the game :)

I play this game, Minecraft, and I have to find a place called stronghold, which can only be found by throwing "eye of enders" in the air, that go in the direction of where the stronghold is hidden. The only problem is, those eyes are very rare and difficult to get, so I thought something about this: by throwing only 2 ender eyes, I can get the coordinates of the stronghold. The picture might be more understandable. You guys think this can be done by using trigonometry? What formula should I use?? :)

Thanks in advance,
Nexor.

EDIT: x3,y3 would be the stronghold's location. :)

 

[rustynail]

I play this game, Minecraft, and I have to find a place called stronghold, which can only be found by throwing "eye of enders" in the air, that go in the direction of where the stronghold is hidden. The only problem is, those eyes are very rare and difficult to get, so I thought something about this: by throwing only 2 ender eyes, I can get the coordinates of the stronghold. The picture might be more understandable. You guys think this can be done by using trigonometry? What formula should I use?? :)

Thanks in advance,
Nexor.

EDIT: x3,y3 would be the stronghold's location. :)

If you can think of a way to measure angles using minecraft, it would be possible using the very simple law of sines. But I don't think the eye of enders give you any measures of angles or distances.

 

[HallsofIvy]

Why even throw two? Just continue in the direction given by the first until you get to the right place. Yes, if it is invisible or hard to see even when you are on top of it, "triangulating" like that will give the position to look.

Since you know two angles and two angles, you can find the third: $180- \theta_1- \theta_2$. And then, knowing one distance (between the two points where you "throw the eyes") you can use the sine law:
$$\frac{a}{sin(A)}= \frac{b}{sin(B)}= \frac{c}{sin(C)}$$
to find the other two lengths.

But that is assuming your directions are perfect. If there is even a slight error in your angles, the result may be well off.

 

[Nexor]

Thanks guys! That's the formula I wanted! :) thank you! :D Now I can get to the stronghold D

 

[CellCoree]

I would advise against cheating in any game, as it goes against the spirit of the game and can also have negative consequences in the long run. However, to answer your question, yes, trigonometry can be used to find the stronghold in Minecraft. The formula you would need to use is the law of cosines, which states that in a triangle, the square of one side is equal to the sum of the squares of the other two sides minus twice the product of those two sides and the cosine of the angle between them. In this case, you would have a triangle with two known sides (the distance between you and the ender eye and the distance between the ender eye and the stronghold), and one unknown angle (the angle between those two sides). By rearranging the formula, you can solve for the unknown angle and then use that to determine the coordinates of the stronghold. 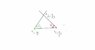However, once again, I would advise against cheating and instead encourage you to play the game as intended.