- #1
Math Amateur
Gold Member
MHB
- 3,990
- 48
- TL;DR Summary
- I am quite perplexed by Sohrab's proof of the Lindelof Covering Theorem ... any clear explanations of the strategy and tactics of the proof are very welcome ...
I am reading Houshang H. Sohrab's book: "Basic Real Analysis" (Second Edition).
I am focused on Chapter 4: Topology of ##\mathbb{R}## and Continuity ... ...
I need help in order to fully understand the proof of Proposition 4.1.1...Proposition 4.1.1, some preliminary notes and its proof read as follows:
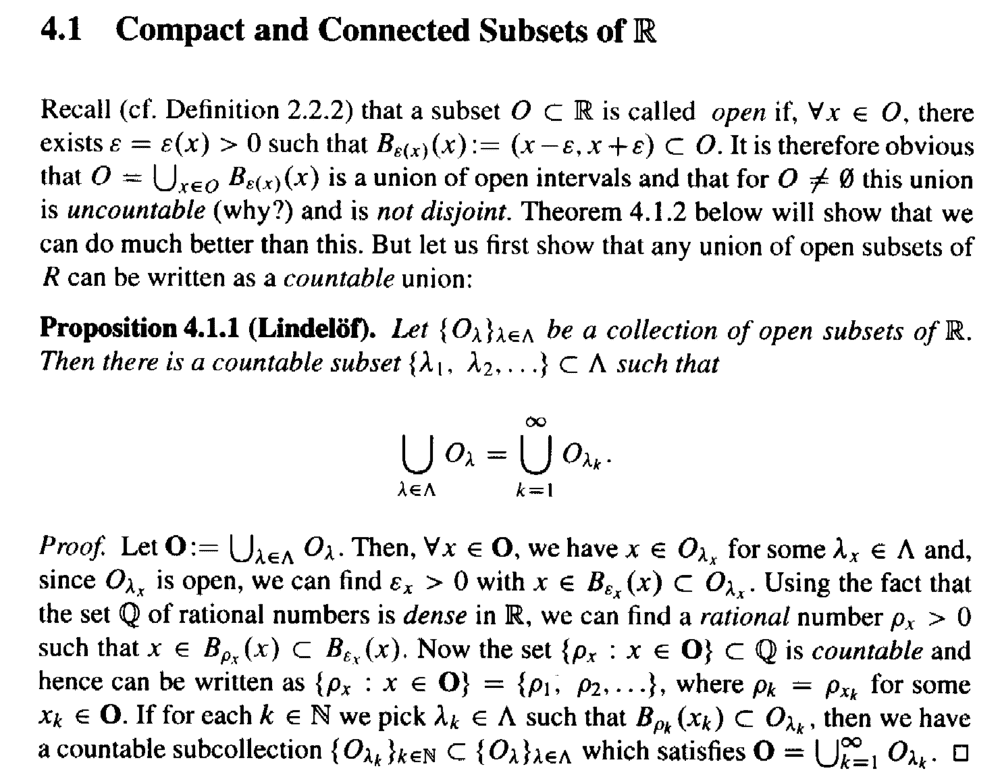 My questions are as follows:Question 1
My questions are as follows:Question 1
In the above proof by Sohrab we read the following:
" ... ... Now the set ##\{ \rho_x \ : \ x \in O \} \subset \mathbb{Q}## is countable ... ... "
But ... it seems to me that since the ##x##'s are uncountable that the number of ##\rho_x## is uncountable ... but that many (at times infinitely many ... ) have the same values since each is equal to a rational number and these are countable ...
... so in fact there are an uncountably infinite number of open balls ##B_{ \rho_x } (x)## ... there are just a countable number of different values for the radii of the open balls ...
Is my interpretation correct ... ?
Question 2
In the above proof by Sohrab we read the following:
" ... ... If for each ##k \in \mathbb{N}## we pick ##\lambda_k \in B_{ \rho_k } (x_k) \subset O_{ \lambda_k }##, then we have a countable subcollection ##\{ O_{ \lambda_k } \}_{ k \in \mathbb{N} } \subset \{ O_\lambda \}_{ \lambda \in \Lambda }## which satisfies ##O = \bigcup_{ k = 1 }^{ \infty } O_{ \lambda_k }## ... ..."Can someone please explain/demonstrate clearly (preferably in some detail) how the process described actually results in a countable subcollection where ##O = \bigcup_{ k = 1 }^{ \infty } O_{ \lambda_k }## ...
In addition to answers to the two questions, any explanations/clarifications of the overall strategy and tactics of the proof would be very gratefully received ...
Peter
I am focused on Chapter 4: Topology of ##\mathbb{R}## and Continuity ... ...
I need help in order to fully understand the proof of Proposition 4.1.1...Proposition 4.1.1, some preliminary notes and its proof read as follows:
In the above proof by Sohrab we read the following:
" ... ... Now the set ##\{ \rho_x \ : \ x \in O \} \subset \mathbb{Q}## is countable ... ... "
But ... it seems to me that since the ##x##'s are uncountable that the number of ##\rho_x## is uncountable ... but that many (at times infinitely many ... ) have the same values since each is equal to a rational number and these are countable ...
... so in fact there are an uncountably infinite number of open balls ##B_{ \rho_x } (x)## ... there are just a countable number of different values for the radii of the open balls ...
Is my interpretation correct ... ?
Question 2
In the above proof by Sohrab we read the following:
" ... ... If for each ##k \in \mathbb{N}## we pick ##\lambda_k \in B_{ \rho_k } (x_k) \subset O_{ \lambda_k }##, then we have a countable subcollection ##\{ O_{ \lambda_k } \}_{ k \in \mathbb{N} } \subset \{ O_\lambda \}_{ \lambda \in \Lambda }## which satisfies ##O = \bigcup_{ k = 1 }^{ \infty } O_{ \lambda_k }## ... ..."Can someone please explain/demonstrate clearly (preferably in some detail) how the process described actually results in a countable subcollection where ##O = \bigcup_{ k = 1 }^{ \infty } O_{ \lambda_k }## ...
In addition to answers to the two questions, any explanations/clarifications of the overall strategy and tactics of the proof would be very gratefully received ...
Peter