- #1
Measle
- 4
- 0
(mentor note: this is a homework problem with a solution that the OP would like to understand better)
In Taylor's Complex Variables,
Example 1.4.10
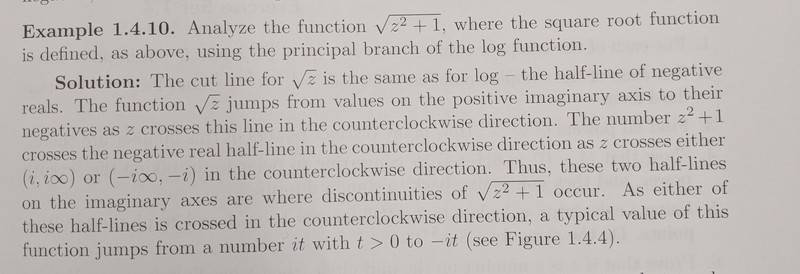
Can someone help me understand this? I don't know what they mean by (i, i inf), or how they got it and -it
In Taylor's Complex Variables,
Example 1.4.10
Can someone help me understand this? I don't know what they mean by (i, i inf), or how they got it and -it
Attachments
Last edited by a moderator: