Confusion about the independent variable of function in magnetostaics.

[Adesh]

When we calculate the curl of magnetic field, that is the curl of Biot-Savart equation for magnetic field. Please consider these

.

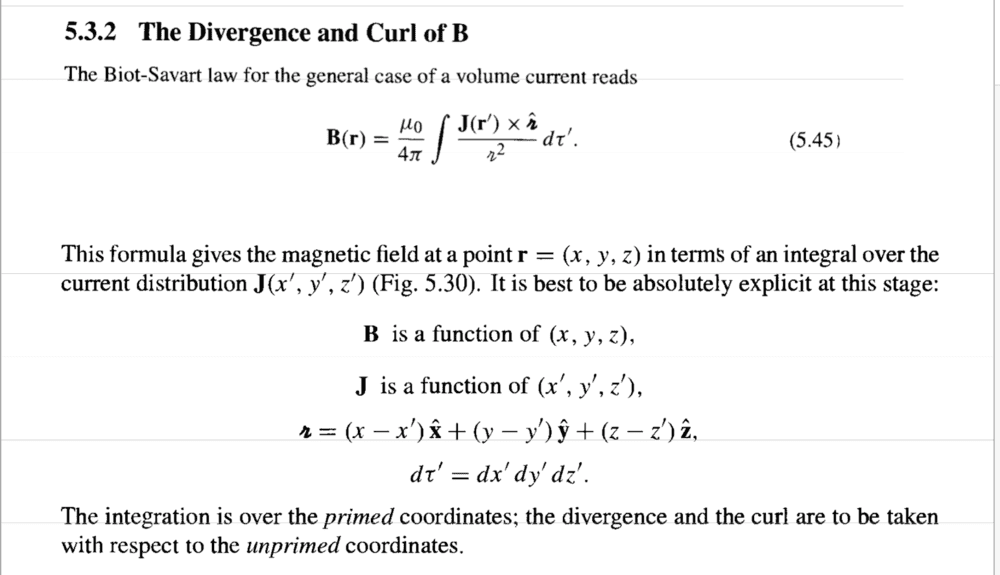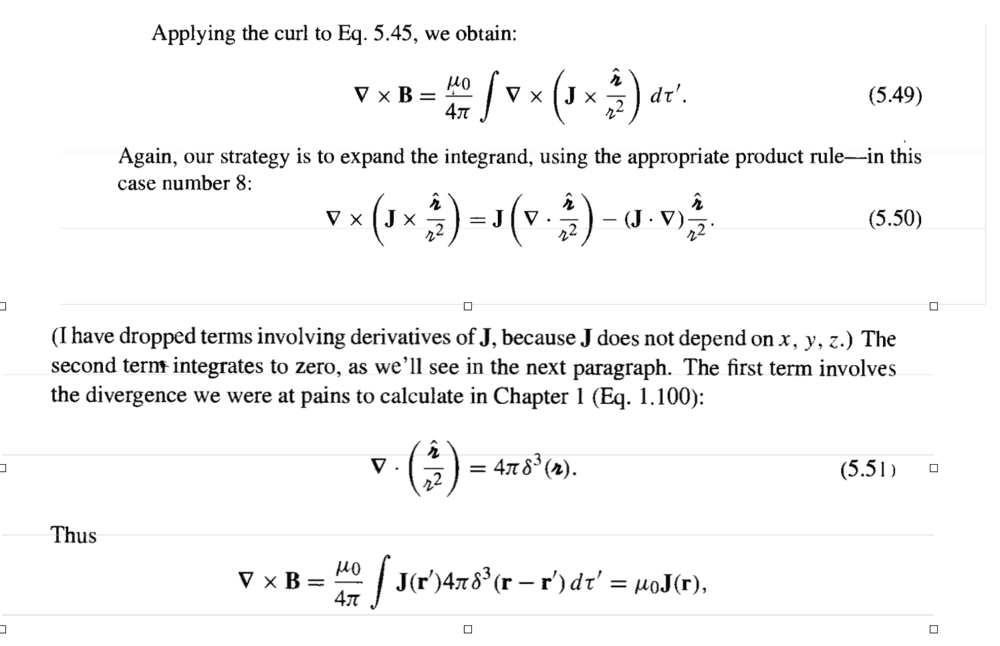
The working of last equation $$ \nabla \times \mathbf {B} = \frac
{\mu_0} {4\pi}
\int \mathbf {J} (\mathbf r') 4\pi \delta ^3 (\mathbf r - \mathbf r') dV' = \mu_0 \mathbf J (\mathbf r) $$
is totally understandable by me, I know that $\delta ^3 (\mathbf r - \mathbf r')$ is zero everywhere except when $\mathbf r' = \mathbf r$ and integral of $\delta ^3$ is just $1$ therefore we got $\mathbf J$ evaluated at $\mathbf r$.

But my problem is, if you see the first screen shot there it is very clearly written $$\mathbf J \textrm{is a function of x' , y' , z'}$$ but you see in the last equation we got $\mathbf J (\mathbf r)$ that is $\mathbf J (x, y , z)$ (unprimed variables). So, what does it really mean when the book wrote $\mathbf J (\mathbf r)$? How can we ever measure $\mathbf J$ at $\mathbf r$ when it is a function of $\mathbf r'$?

When in the integration we made the argument "at $\mathbf r' = \mathbf r$ we get intgeral of $\delta^3$ as 1 and therefore we get $\mathbf J (\mathbf r)$ " did we made some mistake?

 

[Dale]

r’ is just a dummy variable inside the integral. It still represents position.

 

[PeroK]

This looks like a very similar question to this one:

https://www.physicsforums.com/threads/why-f-is-no-longer-a-function-of-x-and-y.984009/

In general:
$$\int f(\vec r')\delta^3(\vec r - \vec r') dV' = f(\vec r)$$

 

[Adesh]

r’ is just a dummy variable inside the integral. It still represents position.

Yes it represents the position and I agree with that but $\mathbf r’$ and $\mathbf r$ are totally from different realms although they represent the same thing.

 

[Adesh]

This looks like a very similar question to this one:

https://www.physicsforums.com/threads/why-f-is-no-longer-a-function-of-x-and-y.984009/

In general:
$$\int f(\vec r')\delta^3(\vec r - \vec r') dV' = f(\vec r)$$

Last time by your great efforts I understood that $\mathbf J$ was a variable of $\mathbf r’$ which although represents the position but is totally different from $\mathbf r$ and hence it’s derivative with respect to $\mathbf r$ is also zero. So, how can we measure $\mathbf J$ at $\mathbf r$ when it does not depend on it.

 

[PeroK]

Yes it represents the position and I agree with that but $\mathbf r’$ and $\mathbf r$ are totally from different realms although they represent the same thing.

They are not from different realms. $\mathbf r$ is a point somewhere in space. You could mark that spot with an $X$. And $\mathbf r’$ is your integration variable, rangeing all over space, including the point $X$.

The function $\delta^3(\mathbf r - \mathbf r’)$ is zero everywhere, except at the point $X$, where the integration variable coincides with $\mathbf r$ .

 

[Adesh]

They are not from different realms. $\mathbf r$ is a point somewhere in space. You could mark that spot with an $X$. And $\mathbf r’$ is your integration variable, rangeing all over space, including the point $X$.

The function $\delta^3(\mathbf r - \mathbf r’)$ is zero everywhere, except at the point $X$, where the integration variable coincides with $\mathbf r$ .

What does this notation $\mathbf J (\mathbf r)$ means? Does it mean the value of J at r ?

 

[PeroK]

What does this notation $\mathbf J (\mathbf r)$ means? Does it mean the value of J at r ?

Yes. This is just another example of defining a function of one variable by integrating over a joint function of that variable and a dummy integration variable. The same as:
$$f(x) = \int g(x, y) dy$$

 

[Adesh]

Yes. This is just another example of defining a function of one variable by integrating over a joint function of that variable and a dummy integration variable. The same as:
$$f(x) = \int g(x, y) dy$$

How can we measure J at r? How your example $$ f (x) = \int g(x,y) dy $$ relates to our situation now?

 

[PeroK]

How can we measure J at r? How your example $$ f (x) = \int g(x,y) dy $$ relates to our situation now?

If you really don't see that, are you sure you have the mathematical prerequisites for EM? Griffiths has 50 pages of mathematics at the start of that book. You really do need to be on top of all that mathematics - at lesat to the point where you can understand it.

All of your questions are based on not understanding the concept of a dummy integration variable in defining a function. The simplest example of which is the one I gave.

Your example is a vector function of a vector variable, which is a generalisation of this basic idea.

 

[Adesh]

If you really don't see that, are you sure you have the mathematical prerequisites for EM? Griffiths has 50 pages of mathematics at the start of that book. You really do need to be on top of all that mathematics - at lesat to the point where you can understand it.

All of your questions are based on not understanding the concept of a dummy integration variable in defining a function. The simplest example of which is the one I gave.

Your example is a vector function of a vector variable, which is a generalisation of this basic idea.

I understand the basics that are required for that book. I don’t how I got so much confused here.

I fully understand that when you wrote $$ f(x) = \int g(x,y) dy$$ you’re making our $f$ to depend on $x$.

 

[Dale]

Yes it represents the position and I agree with that but $\mathbf r’$ and $\mathbf r$ are totally from different realms although they represent the same thing.

A function of position is a function of position regardless of if you label position r, r’, or (x,y,z). I have no idea what you mean by “different realms”. It is just position. J is a function of position irrespective of what symbol you label position with.

 

[Adesh]

A function of position is a function of position regardless of if you label position r, r’, or (x,y,z). I have no idea what you mean by “different realms”. It is just position.

Differentiating $\mathbf J (\mathbf r’)$ with respect to $\mathbf r$ going to give us 0 and that shows r and r’ are from different realms.

 

[Dale]

Differentiating $\mathbf J (\mathbf r’)$ with respect to $\mathbf r$ going to give us 0 and that shows r and r’ are from different realms.

This is nonsense. There is no calculus textbook that even talks about “realms”.

If they are from “different realms” (whatever that means) then how can you have r-r’?

 

[Adesh]

This is nonsense. There is no calculus textbook that even talks about “realms”.

If they are from “different realms” (whatever that means) then how can you have r-r’?

Different realms mean they x-axes of different coordinate systems although they represent the same thing that is position that is numbers.

 

[PeroK]

Differentiating $\mathbf J (\mathbf r’)$ with respect to $\mathbf r$ going to give us 0 and that shows r and r’ are from different realms.

The whole integrand depends on $\mathbf r’$ and $\mathbf r$. Some components of the integrand may be a function of one or the other. The components of the integrand that don't depend on $\mathbf r$ have zero derivative wrt $\mathbf r$.

But, that's largely irrelevant here. Here you are integrating wrt $\mathbf r’$.

We've had two long threads about this now.

 

[Dale]

Different realms mean they x-axes of different coordinate systems although they represent the same thing that is position that is numbers.

This is wrong. The axes and the positions are the same. The point r=(a,b,c) labels the same physical point as r’=(a,b,c)

You didn’t answer my question. If they are different realms then how can you write r-r’.

 

[Adesh]

I highly apologise for my inability to understand this issue. I seem to be understanding nothing but believe me it’s really hard feeling to live with. I’m making long threads/discussions and I sincerely apologise for that, especially to @PeroK.

I will not be too demanding but I request you to please explain this situation with some other model. I request you to explain my non-existing doubt with the help of some other example. If possible please do that and it will be over forever.

 

[Adesh]

You didn’t answer my question. If they are different realms then how can you write r-r’.

Because their units are same and hence we can add and subtract them.

 

[Dale]

Because their units are same and hence we can add and subtract them.

Then your idea of “realms” is meaningless. You can use one “realm” wherever you can use another. The “realms” can be treated as equivalent operationally.

As it is a nonstandard concept not found in textbooks you need to drop this “realms” concept

 

[Adesh]

I think my problem is same as “why you cannot subrtract $x$ from $x^2$, after all they represent the algebraic numbers only?” and I don’t know how I ran into this mud. My brain is out of it’s mind.

 

[Adesh]

Then your idea of “realms” is meaningless. You can use one “realm” wherever you can use another. The “realms” can be treated as equivalent operationally.

As it is a nonstandard concept not found in textbooks you need to drop this “realms” concept

Okay let’s drop it.

 

[Dale]

I think my problem is same as “why you cannot subrtract $x$ from $x^2$, after all they represent the algebraic numbers only?”

You certainly can add $x$ and $x^2$ as long as $x$ is unitless. I don’t see the relevance to this r and r’ confusion at all.

Suppose I have some arbitrary function $f(x)$. If I want to compute the anti derivative $F(x)$ I can do that by $F(x)=\int_0^x f(x’) dx’$

That is all that is happening here. It is just a dummy variable for integration.

 

[Adesh]

All right! Let’s say $f(x) = x^2 + 2x$ and now consider this integral $$ \int f(x) \delta (x-y) dx$$ we know that it equals to $f(y)$ which is just $y^2 + 2y$. Hence, $f$ can be evaluated at y although it is a variable of x. Am I right here?

 

[Dale]

Yes.

 

[PeroK]

All right! Let’s say $f(x) = x^2 + 2x$ and now consider this integral $$ \int f(x) \delta (x-y) dx$$ we know that it equals to $f(y)$ which is just $y^2 + 2y$. Hence, $f$ can be evaluated at y although it is a variable of x. Am I right here?

$f$ is a function. It's not tied to any variable. $f(x)$ is a number that depends on $x$; and $f(y)$ is a number that depends on $y$. And $f(a)$ is a number that depends on $a$ etc.

And dare I point out that $x$ is a dummy integration variable in any case!

 

[Adesh]

Thank you everyone.