Counterweight calculation

[Alistair Vowles]

I hope this will be an easy one to answer that I could just use a little help with.

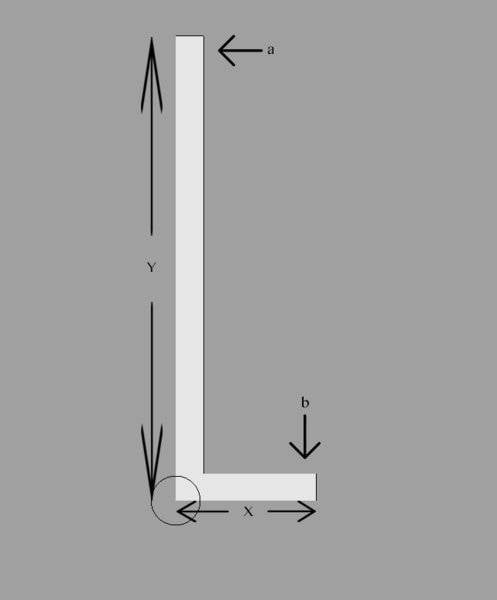
I have an L shaped bar where 'X' is on a flat surface. The pivot point is the circle.

Can someone help me with a formula to calculate the force needed at point 'b' , given a certain force applied at point 'a' , to not allow the bar to pivot.

Force 'a' will be a varying force (wind) so I want to make sure I have enough counterweight for force 'b' to allow for this variable.

The fig is not to scale and I plan to have the length of 'X' to be adjustable to decrease the force needed at point 'b' .

I hope this makes sense and thank you for the help !

 

[CWatters]

b=a*y/x

 

[CWatters]

If you want an explanation...

If you don't want it to move/accelerate then the net torque must be zero.

Let's define counter clockwise as positive. Then...

ay - bx = 0

Rearrange to give...

ay = bx
Then
b = ay/x

 

[Alistair Vowles]

Thankyou very much. This is perfect and exactly what I needed.

 

[Kenwstr]

Um, force being wind resistance is spread all the way along y but can be taken as applying at the average point (y/2) (roughly) to simplify the calculation.

So, b = ay/x/2


Be aware though that if the base is on the ground or a surface, there is a velocity gradient increasing with distance from the surface which is the reason for (roughly). If the structure is very tall, this could be a significant consideration. If it's an engineering question, you will need at least double, probably quadruple (according to regulation) b to provide a safety factor. This is especially important for aero forces as they are proportional to velocity squared and wind is gusty.

You may already have taken all this into account, it's not stated.