- #1
askor
- 169
- 9
Please refer to the below image (Example 5).
Do anyone know how 5x^4 > 1 > 0?
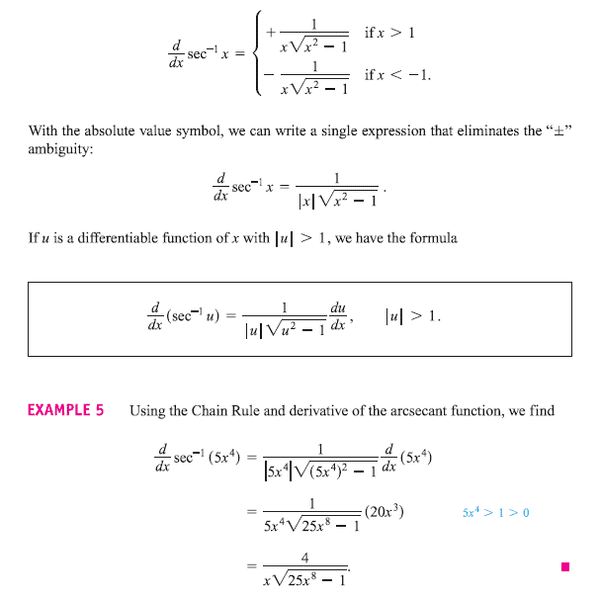
Do anyone know how 5x^4 > 1 > 0?
Last edited:
askor said:Please refer to the below image (Example 5).
Do anyone know how 5x^4 > 1 > 0?
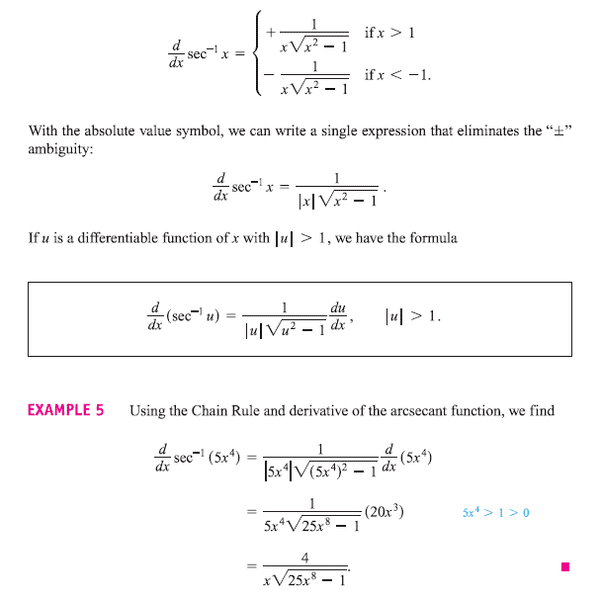
Math_QED said:If ##5x^4 < 1## there would be a negative number under the root thus this is not part of the domain of the function.Therefore they assume ##5x^4 \geq 1##.
fresh_42 said:I suspect that the domain here are the real numbers, although not explicitly mentioned. But for ##|u| < 1## the root ##\sqrt{u^2-1}## becomes negative, and square roots of negative numbers like ##\sqrt{-1}## aren't real. ##|u|=1## is forbidden since the denominator would be zero.
For ##u=5x^4## this translates to the requirement ##|u|=|5x^4|=5x^4 > 1##.
Yes.askor said:So, if ##|u| < 1## and ##|u| = 1## are forbidden, what the value of ##|u|## should be?
Is it ##|u| > 1##?
No.If yes, isn't ##|u| > 1## is equal to ##-1 > u > 1## (from what I learned about inequality property)?
I know you don't mean what you said. If |u| < 1, then ##u^2 - 1 < 0##, so ##\sqrt{u^2 - 1}## isn't real.fresh_42 said:I suspect that the domain here are the real numbers, although not explicitly mentioned. But for ##|u| < 1## the root ##\sqrt{u^2-1}## becomes negative
fresh_42 said:, and square roots of negative numbers like ##\sqrt{-1}## aren't real. ##|u|=1## is forbidden since the denominator would be zero.
For ##u=5x^4## this translates to the requirement ##|u|=|5x^4|=5x^4 > 1##.
By which branch of the graph of y = sec-1(x) you're on. See the graph here: http://www.wolframalpha.com/input/?i=y=arcsec(x)askor said:How do I know if x > 1 or x < -1?
The derivative of inverse secant is equal to -1 divided by the square root of x squared minus 1. This can also be written as -1/(sqrt(x^2 - 1)).
To find the derivative of inverse secant, you can use the formula d/dx(sec^-1(x)) = -1/(sqrt(x^2 - 1)). Alternatively, you can also use the chain rule and the derivative of secant to find the derivative of inverse secant.
The inverse of secant is also known as arcsecant or sec^-1. It is the inverse function of secant and represents the angle whose secant is equal to a given value.
The derivative of inverse secant is important in calculus and other areas of mathematics because it allows us to calculate the rate of change of a function that involves inverse secant. It is also used in solving optimization problems and finding maximum and minimum values.
Yes, the derivative of inverse secant can be simplified using trigonometric identities. For example, by using the identity sec^2(x) = 1 + tan^2(x), we can simplify the derivative to -tan(x)/sqrt(x^2 - 1).