- #1
Guillem_dlc
- 184
- 15
- Homework Statement
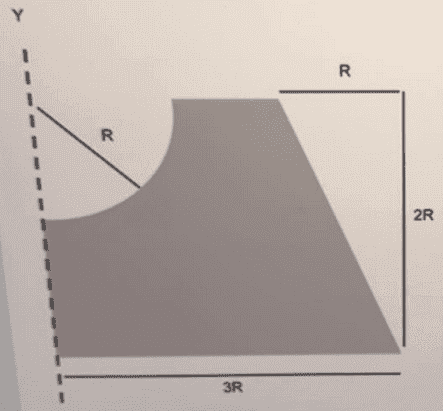
- Determine the volume of the shaded area around the Y-axis by using the theorem of Pappus Guldinus, where value of R = 143,3 cm.
a) Determine the area of the shaded section.
b) Determine the center of gravity of the shaded section.
c) Detrmine the volume by using the theorem of Pappus Guldinus.
- Relevant Equations
- theorem of Pappus Guldinus, center of gravity
Determine the volume of the shaded area around the Y-axis by using the theorem of Pappus Guldinus, where value of R = 143,3 cm.
a) Determine the area of the shaded section.
b) Determine the center of gravity of the shaded section.
c) Detrmine the volume by using the theorem of Pappus Guldinus.
My attempt at the solution:
a) ##A_T=2R\cdot 2R-\dfrac14 \cdot \pi \cdot R^2+\dfrac{R\cdot 2R}{2}##
##A_T=4R^2-\dfrac{\pi \cdot R^2}{4}+R^2=4\cdot 143,3^2-\dfrac{\pi \cdot 143,3^2}{2}+143,3^2=86546,39\, \textrm{cm}^2=\boxed{8,65\, \textrm{m}^2}##
b) I consider three figures to calculate the centres of gravity:
Figure 1 (Rectangular triangle with side R and hypotenuse 2R) --> ##\bar{x}=2R+\dfrac{R}{3}=2\cdot 143,3+\dfrac{143,3}{3}=334,36\, \textrm{cm}##
##\bar{y}=\dfrac{2R}{3}=\dfrac{2\cdot 143,3}{3}=95,53\, \textrm{cm}##
Figure 2 (Square of side 2R) --> ##\bar{x}=\dfrac{2R}{2}=R=143,3\, \textrm{cm}##
##\bar{y}=\dfrac{2R}{2}=R=143,3\, \textrm{cm}##
Figure 3 (sector of circle)--> ##\bar{x}=\dfrac{4r}{3\pi}=\dfrac{4\cdot 143,3}{3\pi}=60,82\, \textrm{cm}##
##\bar{y}=2R-\dfrac{4R}{3\pi}=2\cdot 143,3-\dfrac{4\cdot 143,3}{3\pi}=225,78\, \textrm{cm}##
Then:
##\bar{x}=\dfrac1A\sum_{i=1}^n \bar{x_i}A_i=\dfrac{334,36\cdot 143,3^2+143,3\cdot 4\cdot 143,3^2-60,82\cdot \frac{\pi \cdot 143,3^2}{2}}{86546,39}=192,67##
This last result would be correct?
Thanks
a) Determine the area of the shaded section.
b) Determine the center of gravity of the shaded section.
c) Detrmine the volume by using the theorem of Pappus Guldinus.
My attempt at the solution:
a) ##A_T=2R\cdot 2R-\dfrac14 \cdot \pi \cdot R^2+\dfrac{R\cdot 2R}{2}##
##A_T=4R^2-\dfrac{\pi \cdot R^2}{4}+R^2=4\cdot 143,3^2-\dfrac{\pi \cdot 143,3^2}{2}+143,3^2=86546,39\, \textrm{cm}^2=\boxed{8,65\, \textrm{m}^2}##
b) I consider three figures to calculate the centres of gravity:
Figure 1 (Rectangular triangle with side R and hypotenuse 2R) --> ##\bar{x}=2R+\dfrac{R}{3}=2\cdot 143,3+\dfrac{143,3}{3}=334,36\, \textrm{cm}##
##\bar{y}=\dfrac{2R}{3}=\dfrac{2\cdot 143,3}{3}=95,53\, \textrm{cm}##
Figure 2 (Square of side 2R) --> ##\bar{x}=\dfrac{2R}{2}=R=143,3\, \textrm{cm}##
##\bar{y}=\dfrac{2R}{2}=R=143,3\, \textrm{cm}##
Figure 3 (sector of circle)--> ##\bar{x}=\dfrac{4r}{3\pi}=\dfrac{4\cdot 143,3}{3\pi}=60,82\, \textrm{cm}##
##\bar{y}=2R-\dfrac{4R}{3\pi}=2\cdot 143,3-\dfrac{4\cdot 143,3}{3\pi}=225,78\, \textrm{cm}##
Then:
##\bar{x}=\dfrac1A\sum_{i=1}^n \bar{x_i}A_i=\dfrac{334,36\cdot 143,3^2+143,3\cdot 4\cdot 143,3^2-60,82\cdot \frac{\pi \cdot 143,3^2}{2}}{86546,39}=192,67##
This last result would be correct?
Thanks