Exploring Differential of Vector Component vs Change During Parallel Transport

[Shirish]

I'm reading 'Core Principles of Special and General Relativity' by Luscombe - the part on parallel transport.

A vector $v$ given at every point of $\gamma(\lambda)$ with tangent vector $U^{\beta}$ is said to be parallel transported if the covariant derivative vanishes at all points of $\gamma(\lambda)$: $$U^{\mu}\nabla_{\mu}v^{\alpha}=U^{\mu}(\partial_{\mu}v^{\alpha}+\Gamma^{\alpha}_{\ \ \rho\mu}v^{\rho})=0$$

I guess $U^{\beta}$ and $v$ are vector fields instead of vectors as claimed in the quote. Till here I can understand, but then it's written:

Armed with the knowledge of parallel transport, we can complete the picture of the covariant derivative. Let $\delta A^{\mu}\equiv -\Gamma^{\mu}_{\ \ \beta\nu}A^{\beta}\text{d}x^{\nu}$ denote the change in $A^{\mu}$ that occurs in parallel transport through $\text{d}x^{\nu}$ (see fig below).

I want to clarify my understanding of the part in bold. My current interpretation is: we can talk about the value of connection coefficients $\Gamma^{\mu}_{\ \ \beta\nu}$ _only in reference to some chart_, so let that chart be $(U,x)$.

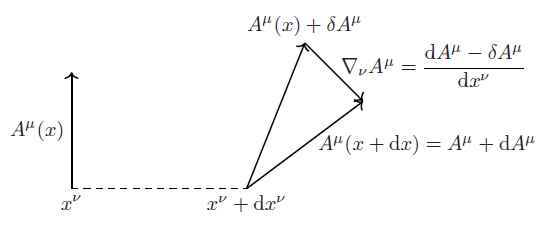
Initially we consider a vector field $A$ with components $A^{\mu}$ and we start at some point $p\in U$ with coordinates $x^{\nu}$. The change in $A^{\mu}$, according to the text, is $-\Gamma^{\mu}_{\ \ \beta\nu}A^{\beta}(p)\text{d}x^{\nu}$.

But from parallel transport condition, we have $\partial_{\nu}A^{\mu}(p)=-\Gamma^{\mu}_{\ \ \beta\nu}A^{\beta}(p)$ and so, from what the book says, $$\begin{align}
\delta A^{\mu}\equiv\partial_{\nu}A^{\mu}(p)\ \text{d}x^{\nu}&=\text{d}A^{\mu}(p)
\end{align}$$

It seems like I can interpret the differential of $A^{\mu}$ at $p$, i.e. $\text{d}A^{\mu}(p)$ as the change in value of $A^{\mu}$ as I incrementally move from $p=x^{\nu}$ to $p'\equiv x^{\nu}+\text{d}x^{\nu}$.

But if you look at the figure, $A^{\mu}+\text{d}A^{\mu}$ and $A^{\mu}+\delta A^{\mu}$ are different, and so $\text{d}A^{\mu}$ and $\delta A^{\mu}$ are different, contradicting the equation I wrote above.

Where am I going wrong?

 

[PeterDonis]

if you look at the figure, $A^{\mu}+\text{d}A^{\mu}$ and $A^{\mu}+\delta A^{\mu}$ are different

That's because they are describing two different things.

$A^{\mu}+\text{d}A^{\mu}$ is describing the actual value of the vector field $A^\mu$ at $x^\mu + \text{d} x^\mu$, as compared with the value of the vector field $A^\mu$ at $x^\mu$.

$A^{\mu}+\delta A^{\mu}$ is describing what you would get if you took the value of the vector field $A^\mu$ at $x^\mu$ and parallel transported it to $x^\mu + \text{d} x^\mu$.

They are different because the covariant derivative of $A^\mu$ is not zero.

 

[Shirish]

That's because they are describing two different things.

$A^{\mu}+\text{d}A^{\mu}$ is describing the actual value of the vector field $A^\mu$ at $x^\mu + \text{d} x^\mu$, as compared with the value of the vector field $A^\mu$ at $x^\mu$.

$A^{\mu}+\delta A^{\mu}$ is describing what you would get if you took the value of the vector field $A^\mu$ at $x^\mu$ and parallel transported it to $x^\mu + \text{d} x^\mu$.

They are different because the covariant derivative of $A^\mu$ is not zero.

I understand and agree. In that case, where did I go wrong in concluding equation $(1)$?

 

[PeterDonis]

where did I go wrong in concluding equation $(1)$?

You assumed that $A^\mu + \text{d} A^\mu$ is what you get by parallel transporting $A^\mu$ from $x^\mu$ to $x^\mu + \text{d} x^\mu$. It isn't.

 

[strangerep]

[...] where did I go wrong in concluding equation $(1)$?

Once again, Luscombe's exposition can be a bit confusing. He says

Figure 14.4
$A^\mu (x)$ is parallel transported from $x^\nu$ to $x^\nu + dx^\nu$, picking up an extra component $\delta A^\mu$ . The covariant derivative is the difference $A^\mu(x + dx^ν ) − (A^\mu (x) + \delta A^\mu)$.

What's really meant is the difference between the parallel-transported version of $A^\mu$ and the coordinate-translated version. Your equation is correct if and only if $A^\mu(x)$ is in fact a covariantly-constant vector field along the $\nu$'th coordinate direction.

I'm starting to think you need a couple of other textbooks besides Luscombe that cover the same subject material -- so you can see how other experts explain these things.

 

[Shirish]

You assumed that $A^\mu + \text{d} A^\mu$ is what you get by parallel transporting $A^\mu$ from $x^\mu$ to $x^\mu + \text{d} x^\mu$. It isn't.

I'm afraid I still don't get it. The book mentions that the vector $A$ is being parallel transported through $\text{d}x^{\nu}$, which means that the covariant derivative of $A$ vanishes through whatever part of the $x^{\nu}$ coordinate curve it's being parallel transported. So in that case, the vectors $A^{\mu}+\delta A^{\mu}$ and $A^{\mu}+\text{d}A^{\mu}$ should coincide, as opposed to what's shown in the figure, correct?

Because as I showed in the OP, $\delta A^{\mu}$ as defined by the author equals $\text{d}A^{\mu}$ if we assume the parallel transport condition. I can only guess then that in the quote "Let $\delta A^{\mu}\equiv -\Gamma^{\mu}_{\ \ \beta\nu}A^{\beta}\text{d}x^{\nu}$ denote the change in $A^{\mu}$ that occurs in parallel transport through $\text{d}x^{\nu}.$", the parallel transport bit is dicey.

 

[Shirish]

Once again, Luscombe's exposition can be a bit confusing. He says

What's really meant is the difference between the parallel-transported version of $A^\mu$ and the coordinate-translated version. Your equation is correct if and only if $A^\mu(x)$ is in fact a covariantly-constant vector field along the $\nu$'th coordinate direction.

I'm starting to think you need a couple of other textbooks besides Luscombe that cover the same subject material -- so you can see how other experts explain these things.

Thank you! And yeah even on Math SE I got the same advice - to switch to some other book. I'll look up a suitable replacement!

One more thing regarding the coordinate-translated version - need to clarify the physical interpretation of it. Forgetting everything about parallel transport and covariant derivatives and all for the time being - let $A$ be a vector field, $(U,x)$ a chart and $p,p'$ have coordinates $x^{\nu}$ & $x^{\nu}+\text{d}x^{\nu}$ respectively. Then if $A^{\mu}$ are the coordinates of $A(p)$, then $A^{\mu}+\text{d}A^{\mu}$ will be the coordinates of $A(p')$, right? If so, I'll try to come up with a proof of that.

 

[PeterDonis]

The book mentions that the vector $A$ is being parallel transported through $\text{d}x^{\nu}$,

The book is comparing the actual value of the vector field at $x^\mu + \text{d} x^\mu$, which is $A^\mu + \text{d} A^\mu$, with the value that would be obtained by parallel transporting the vector at $x^\mu$ to $x^\mu + \text{d} x^\mu$, which is $A^\mu + \delta A^\mu$. So there are two vectors being compared, and only one is obtained by parallel transport.

the covariant derivative of $A$ vanishes through whatever part of the $x^{\nu}$ coordinate curve it's being parallel transported.

You are looking at it backwards. What the book is trying to explain is that the covariant derivative does not vanish, if the actual value of the vector field at $x^\mu + \text{d} x^\mu$ is different from the value you would get by parallel transporting the vector at $x^\mu$ to $x^\mu + \text{d} x^\mu$.

It seems like you already understand this, so the real issue appears to be that the book's wording isn't very clear. If that's the issue, I agree it isn't very clear.

 

[PeterDonis]

if $A^{\mu}$ are the coordinates of $A(p)$, then $A^{\mu}+\text{d}A^{\mu}$ will be the coordinates of $A(p')$, right?

No. $A^\mu$ is a vector field, not coordinates. The coordinates of $p$ are $x^\mu$, and the coordinates of $p'$ are $x^\mu + \text{d} x^\mu$. "The coordinates of $A(p)$" makes no sense; what makes sense is something like "the value of the vector field $A$ at the point $p$ is $A(p)$".

 

[Shirish]

No. $A^\mu$ is a vector field, not coordinates. The coordinates of $p$ are $x^\mu$, and the coordinates of $p'$ are $x^\mu + \text{d} x^\mu$. "The coordinates of $A(p)$" makes no sense; what makes sense is something like "the value of the vector field $A$ at the point $p$ is $A(p)$".

Sorry, I meant components of the vector field. Typo

 

[PeterDonis]

I meant components of the vector field

So you're asking if $A^\mu + \text{d} A^\mu$ are the components of the vector field at the point $p'$? Yes, that's correct.

 

[Shirish]

So you're asking if $A^\mu + \text{d} A^\mu$ are the components of the vector field at the point $p'$? Yes, that's correct.

Thanks for clarifying! I'll attempt to prove that. I guess the trick will lie in relating the basis vectors at $p$ and $p'$ somehow.

 

[PeterDonis]

I'll attempt to prove that.

There's nothing to prove; it's true by definition--the book is defining $A^\mu + \text{d} A^\mu$ to be the value of the vector field $A$ at the point $p'$.

 

[Shirish]

There's nothing to prove; it's true by definition--the book is defining $A^\mu + \text{d} A^\mu$ to be the value of the vector field $A$ at the point $p'$.

Hm, so I'm not to interpret $\text{d}A^{\mu}$ as the differential of the smooth function $A^{\mu}$?

I was interpreting the relation as $$A^{\mu}(p')=A^{\mu}(p)+\text{d}A^{\mu}(p)$$ so if the last term is indeed the differential of $A^{\mu}$ at $p$, then this relation probably needs to be proved and the author can't simply pull it out of thin air (or simply say that the above relation is a "definition") without proving it.

 

[PeterDonis]

so I'm not to interpret as the differential of the smooth function ?

First, $A^\mu$ is a smooth vector field, not a smooth function.

Second, it depends on what you mean by "differential". See below.

I was interpreting the relation as $$A^{\mu}(p')=A^{\mu}(p)+\text{d}A^{\mu}(p)$$

Yes, that's what the book means when it says $A^\mu (x + \text{d} x) = A^\mu + \text{d} A^\mu$.

if the last term is indeed the differential of $A^{\mu}$ at $p$, then this relation probably needs to be proved

What do you think "the differential of $A^{\mu}$ at $p$" means, and why would it not be true by definition that $A^\mu (x + \text{d} x) = A^\mu + \text{d} A^\mu$?

 

[Shirish]

What do you think "the differential of $A^{\mu}$ at $p$" means, and why would it not be true by definition that $A^\mu (x + \text{d} x) = A^\mu + \text{d} A^\mu$?

So the way I'm thinking about it is:
$$\text{d}A^{\mu}(p)=\bigg(\frac{\partial A^{\mu}}{\partial x^{\nu}}\bigg)_p\text{d}x^{\nu}$$
where $\{\text{d}x^{\nu}\}$ is the chart-induced cotangent basis at $p$. So essentially the equation
$$A^{\mu}(p')=A^{\mu}(p)+\text{d}A^{\mu}(p)$$ translates to
$$A^{\mu}(p')=A^{\mu}(p)+\bigg(\frac{\partial A^{\mu}}{\partial x^{\nu}}\bigg)_p\text{d}x^{\nu}$$
$A^{\mu}(p')$ and $A^{\mu}(p)$ can be considered smooth functions defined at least locally in $U$ (where $(U,x)$ is the chart), so $A^{\mu}(p), A^{\mu}(p')\in C^{\infty}(U)$. The last term on RHS is an element of the cotangent space at $p$, so $T^*_p(M)$. In effect, we seem to be adding an element of $T^*_p(M)$ to an element of $C^{\infty}(U)$ to get another element of $C^{\infty}(U)$, which seems strange.

Maybe I'm missing something really simple, but the above equation isn't immediately obvious to me.

 

[PeterDonis]

So the way I'm thinking about it is:
$$\text{d}A^{\mu}(p)=\bigg(\frac{\partial A^{\mu}}{\partial x^{\nu}}\bigg)_p\text{d}x^{\nu}$$
where $\{\text{d}x^{\nu}\}$ is the chart-induced cotangent basis at $p$.

No. When the book says the coordinates of the point $p'$ are $x^\mu + \text{d} x^\mu$, $\text{d} x^\mu$ is not the cotangent basis at $p$. It's just a way of writing the infinitesimal difference in the coordinates between $p$ and $p'$. The notation $\text{d} x^\mu$ is unfortunate since it is the same notation that is also used to denote the cotangent basis at $p$, but that's the notation the book chose.

Similarly, when the book writes $\text{d} A^\mu$, it does not mean the differential of some function at $p$. (Note that the book does not use the functional notation $dA^\mu (p)$ that you are using.) It just means the infinitesimal difference between the value of the vector field $A$ at $p$ and the value of the vector field $A$ at $p'$.

 

[Shirish]

No. When the book says the coordinates of the point $p'$ are $x^\mu + \text{d} x^\mu$, $\text{d} x^\mu$ is not the cotangent basis at $p$. It's just a way of writing the infinitesimal difference in the coordinates between $p$ and $p'$. The notation $\text{d} x^\mu$ is unfortunate since it is the same notation that is also used to denote the cotangent basis at $p$, but that's the notation the book chose.

Similarly, when the book writes $\text{d} A^\mu$, it does not mean the differential of some function at $p$. (Note that the book does not use the functional notation $dA^\mu (p)$ that you are using.) It just means the infinitesimal difference between the value of the vector field $A$ at $p$ and the value of the vector field $A$ at $p'$.

Aiiii NOW I get it. I swear differential geometry notation is a colossal pain in the neck! And I'll probably switch to another book now.

 

[strangerep]

What's really meant is the difference between the parallel-transported version of $A^\mu$ and the coordinate-translated version. Your equation is correct if and only if $A^\mu(x)$ is in fact a covariantly-constant vector field along the $\nu$'th coordinate direction.

One more thing regarding the coordinate-translated version - need to clarify the physical interpretation of it.

The "coordinate-translated version" is just the effect of a dumb boring change of coordinates $x^\mu \to x'^\mu = x^\mu + \alpha^\mu$. Its "physical content" is negligble because it's not covariant when applied to vector fields, tensor fields, etc, (which is why we have to introduce a notion of "parallel transport" in the first place).

Forgetting everything about parallel transport and covariant derivatives and all for the time being - let $A$ be a vector field, $(U,x)$ a chart and $p,p'$ have coordinates $x^{\nu}$ & $x^{\nu}+\text{d}x^{\nu}$ respectively. [...]

I get the feeling you're overthinking this. To see the effect of a coordinate translation, just do a multivariate Taylor expansion:
$$\begin{array}{rcl} A'^\mu(x')
&=&\displaystyle \frac{\partial x'^\mu}{\partial x^\lambda} \, A^\lambda(x+\alpha)
~=~ \delta^\mu_\lambda \, A^\lambda(x+\alpha)
~=~ A^\mu(x+\alpha) \\
&=&\displaystyle A^\mu(x) ~+~ \alpha^\sigma \left[ \frac{\partial A^\mu}{\partial x^\sigma} \right]_x ~+~ \dots
\end{array}$$

 

[Shirish]

The "coordinate-translated version" is just the effect of a dumb boring change of coordinates $x^\mu \to x'^\mu = x^\mu + \alpha^\mu$. Its "physical content" is negligble because it's not covariant when applied to vector fields, tensor fields, etc, (which is why we have to introduce a notion of "parallel transport" in the first place).I get the feeling you're overthinking this. To see the effect of a coordinate translation, just do a multivariate Taylor expansion:
$$\begin{array}{rcl} A'^\mu(x')
&=&\displaystyle \frac{\partial x'^\mu}{\partial x^\lambda} \, A^\lambda(x+\alpha)
~=~ \delta^\mu_\lambda \, A^\lambda(x+\alpha)
~=~ A^\mu(x+\alpha) \\
&=&\displaystyle A^\mu(x) ~+~ \alpha^\sigma \left[ \frac{\partial A^\mu}{\partial x^\sigma} \right]_x ~+~ \dots
\end{array}$$

I'm definitely prone to overthinking. I think Taylor expansion would probably need an analytic structure on the manifold (e.g. see here). So that means we assume analytic ($C^{ \omega}$) structure on spacetime manifolds in standard GR? Also, I'm not sure about the $\partial x'^{ \mu}/\partial x^{\lambda}$ terms since $x$ are the coordinates of one point and $x'$ are the coordinates of a different point. That delta function relation follows immediately if these represented coordinates of the same point, but under different chart maps.

Not arguing your point - just saying that from what I know, "stuff at one point can only be related to stuff at the same point". This is closely related to this question I'd asked on Math SE (still unanswered unfortunately):
https://math.stackexchange.com/ques...-points-in-a-small-neighborhood-of-a-point-in

 

[PeterDonis]

The "coordinate-translated version" is just the effect of a dumb boring change of coordinates

I'm not sure the book means it to be a change of coordinate chart. I think the book means it to be a dumb boring change of which point is being considered, using the same coordinate chart. So point $p$, in the chart, has coordinates $x^\mu$, and point $p'$, in the same chart, has coordinates $x^\mu + \text{d} x^\mu$.

 

[strangerep]

I'm definitely prone to overthinking. I think Taylor expansion would probably need an analytic structure on the manifold (e.g. see here). So that means we assume analytic ($C^{\omega}$) structure on spacetime manifolds in standard GR?

Yes, GR assumes a differentiable manifold, as I mentioned earlier.

Also, I'm not sure about the $\partial x'^{\mu}/\partial x^{\lambda}$ terms since $x$ are the coordinates of one point and $x'$ are the coordinates of a different point. That delta function relation follows immediately if these represented coordinates of the same point, but under different chart maps.

Are you familiar with the distinction between "active" and "passive" transformations?

Imagine a coordinate grid laid over a blank manifold. In my Taylor expansion example I showed a passive transformation, where we simply rename the points by overlaying the manifold with a new coordinate grid. The active transformation is essentially the inverse of this, where we keep the old coordinate grid but slide the manifold's points underneath that grid.

So yes, I was always "relating stuff at one point to stuff at the same point".

 

[PeterDonis]

Are you familiar with the distinction between "active" and "passive" transformations?

I don't think the book quoted in the OP is doing either.

 

[strangerep]

[...] "active" and "passive" transformations [...]

I don't think the book quoted in the OP is doing either.

Yet, previously, you said:

I'm not sure the book means it to be a change of coordinate chart. I think the book means it to be a dumb boring change of which point is being considered, using the same coordinate chart. So point $p$, in the chart, has coordinates $x^\mu$, and point $p'$, in the same chart, has coordinates $x^\mu + \text{d} x^\mu$.

That sure sounds like an active transformation to me.

But maybe we shouldn't waste too much time guessing what Luscombe "really" meant. I mentioned passive transformations only to prod the OP into thinking about how coordinate derivatives are not covariant, hence the need for a covariant derivative.

 

[PeterDonis]

That sure sounds like an active transformation to me.

I don't think it's a transformation of the manifold at all. It's not "moving any points". It's just comparing vectors at different points on a fixed manifold.