You're welcome! Keep up the good work!

[bsodmike]

First of all, is there a set means for evaluating decay energy be it an alpha, beta, or gamma decay?

Considering a means for evaluating the http://en.wikipedia.org/wiki/Decay_energy" :

$$Q=\left(m_0c^2\right)_{\text{after}}-\left(m_0c^2\right)_{\text{before}}$$

I evaluated this disintegration of Tritium into Helium-3:

$$^{3}_{1}T\rightarrow~^{3}_{2}He~+~e^-~+~\overline{v}_e$$

Considering the Tritium isotope mass is 3.0160492 u, and Helium-3 isotope mass is 3.0160293 u, $$\Delta m=1.99\times 10^{-5}$$ u.

Hence, $$Q=(1.99\times 10^{-5}\text{u})(939.494~\text{MeV/uc\textsuperscript{2}})(\text{c\textsuperscript{2}})=18.7~\text{keV}$$

I have http://en.wikipedia.org/wiki/Tritium" .

However, considering the following process:

$$^{137}_{55}Cs\rightarrow~^{137}_{56}Ba~+~e^-~+~\overline{v}_e$$

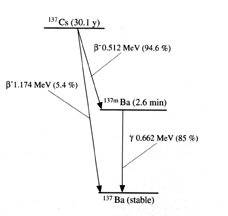
This has been explained as,

Cs137 decays in two ways, (1) beta-decay (maximum energy=0.518 Mev) to Ba137* followed by a gamma-transition to the ground state, and (2) beta-decay (maximum energy=1.2 Mev) directly to the ground state.

and as,

The Cs-137 parent isotope beta decays (~95%) with a 30.17y half-life to produce Ba-137m which in turn decays with a 2.55min. half-live, generating a 661.6 keV gamma ray emission.

Using the earlier method I am obtaining a result of 3.9 MeV if simply considering the rest mass of both caesium-127 (136.91 u) and barium-137 (http://en.wikipedia.org/wiki/Isotopes_of_barium" ). Hence, $$Q=(4.188\times 10^{-3}\text{u})(939.494~\text{MeV/uc\textsuperscript{2}})(\text{c\textsuperscript{2}})=3.9~\text{MeV}$$

I can however see that the beta-decay to Ba-137m is simply taken from $$E=m_0c^2$$ of an electron; thus, $$E=(9.11\times 10^{-31}~\text{kg})(3.00\times 10^{8}~\text{ms\textsuperscript{-1}})^2=8.20\times 10^{-14}~\text{J}$$. Since, $$1~\text{eV}=1.60\times 10^{-19}~\text{J}$$, this results in,

$$E=(8.20\times 10^{-14}~\text{J})\left(\dfrac{1~\text{eV}}{1.60\times 10^{-19}~\text{J}}\right)=0.511~\text{MeV}$$

I would appreciate an elucidation of the above problem; thanks in advance to contributors.

Cheers
Mike.

 

[fatra2]

Hi there,

From what I see, you seem to understand most of the problem. The decay energy comes only from the missing mass question. When a nucleus decays, following anyone of the four potential modes, mass will be transformed into energy following E=mc2.

In experimental physics, the problem comes with a three body decay, like for a beta decay. In this case, as you mentioned, the nucleus emits a beta particle and a neutrino. The energy of the decay is transferred to both the particles, and not necessarily equally. Therefore, the beta particle will have a kinetic energy of up to the decay energy.

Cheers

 

[bsodmike]

Hi there,

From what I see, you seem to understand most of the problem. The decay energy comes only from the missing mass question. When a nucleus decays, following anyone of the four potential modes, mass will be transformed into energy following E=mc2.

In experimental physics, the problem comes with a three body decay, like for a beta decay. In this case, as you mentioned, the nucleus emits a beta particle and a neutrino. The energy of the decay is transferred to both the particles, and not necessarily equally. Therefore, the beta particle will have a kinetic energy of up to the decay energy.

Cheers

Thanks for your reply Fatra. Would you be able to let me know why I seem to be achieving a correct result (following the calculation for estimating decay energy) for $$\beta^-$$-decay of Tritium, but not for Caesium?

In the same application for Caesium, how come I am computing closer to 3.9 MeV (which from the included references in my original post, seems quite incorrect)?

 

[fatra2]

Hi there,

Your calculations are correct. The 0.511MeV is the minimum energy needed to create a beta particle.

Ok, let's see what happens in this Cs decay. Cs decays into Ba, emitting a beta particle and a neutrino. The Ba nucleus is left into an excited state at 0.6616MeV above the ground state.

The potential energy freed from the decay (missing mass) is around:$$\Delta E = \Delta m c^2 \approx 3.019MeV$$. To this, you must take the energy needed to create the beta particle (0.511MeV) and the neutrino (>0.1eV or nothing really). On top of that, the Ba nucleus is left into an excited state of 0.6616MeV, which leaves you with more or less 1.5MeV of maximum kinetic energy for the beta particle.

I did these calculations with very little precision. But we get very close to the 1.176MeV maximum energy, given by the "Physics and Chemistry Handbook - 86 Edition".

Cheers

 

[bsodmike]

Hi there,

Your calculations are correct. The 0.511MeV is the minimum energy needed to create a beta particle.

Ok, let's see what happens in this Cs decay. Cs decays into Ba, emitting a beta particle and a neutrino. The Ba nucleus is left into an excited state at 0.6616MeV above the ground state.

The potential energy freed from the decay (missing mass) is around:$$\Delta E = \Delta m c^2 \approx 3.019MeV$$. To this, you must take the energy needed to create the beta particle (0.511MeV) and the neutrino (>0.1eV or nothing really). On top of that, the Ba nucleus is left into an excited state of 0.6616MeV, which leaves you with more or less 1.5MeV of maximum kinetic energy for the beta particle.

I did these calculations with very little precision. But we get very close to the 1.176MeV maximum energy, given by the "Physics and Chemistry Handbook - 86 Edition".

Cheers

Thank you Fatra. I have just referred up the same book and did indeed find the listing of 1.176 MeV under Cs-137. Referring another source I can see how the end-point energy is far greater than $$E_{\text{max}}=1.176 \text{MeV}$$.

I should have in fact set my original equation out as,

$$^{137}_{55}Cs\rightarrow~^{137}_{56}Ba~+~e^-~+~Q$$

Setting $$Q=E_{\text{max}}$$ should balance the mass-energy of the reaction above, as you pointed out.

 

[fatra2]

I should have in fact set my original equation out as,

$$^{137}_{55}Cs\rightarrow~^{137}_{56}Ba~+~e^-~+~Q$$

Not really. Your first equation was not incorrect, fact is that this one neither. If you want to be perfectly correct, with all the conservation principles, you need to combine the two.

Cheers

 

[bsodmike]

Not really. Your first equation was not incorrect, fact is that this one neither. If you want to be perfectly correct, with all the conservation principles, you need to combine the two.

Cheers

Fatra,

I was looking at the information http://books.google.com/books?id=aM...uclear physics&pg=PA148#v=onepage&q=&f=false". You can see how they have described it there.

Would you be able to give me the completely correct, combined equation? Would it be,

$$^{137}_{55}Cs\rightarrow~^{137}_{56}Ba~+~e^-~+~\overline{v}_e~+Q$$

 

[fatra2]

Looks right!

 

[bsodmike]

Looks right!

Thanks Fatra :)