- #1
lubo
- 36
- 0
Homework Statement
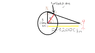
A circle with a radius (r) of 6000km.
From the centre of the circle to a point in the distance G where another person is standing is R = 40000 km.
Calculate the angle (θ) that the observer has to stand at point (O), to allow the observer to see the distant point G. If the observer stands at point N he cannot see point (G)
Point G can be visible by constructing a right angled triangle B O G and you should be able to use trigonometry.
Please see attached for the drawing. Sorry it is not the best quality. We have B at the centre of triangle, N is the top of the circle, O is the point the observer stands to see point G and G where the observer would like to see.
Homework Equations
SOH CAH TOA
A2=B2+C2
Triangles ad up to 180°
The Attempt at a Solution
you can find B - N - G (big triangle) from Pythagoras = √(400002 + 6000) = 40447.5 km.
For the triangle (BOG) where the observer stands and looks to G, because it is at an angle from the centre of the circle to (O) I am stuck how to calculate it.
Sine Rule cannot be used as I have no angles.
I could maybe use the Cosine rule c2= a2+b2 - 2ab Cos C to find the angle.
C= cos-1 ((-c2 + a2+b2)/(2ab)) but the angle does not look like it is right angled so therefore I am not sure how I can work out O - G.
If I try and use a small triangle B - N - O -B then I have the same radius for both parts of the triangle and of course they will cancel out as c2 - b2 = a = Zero if both b and c are the same i.e. 6000.
I hope this makes sense as it is quite hard to explain as I cannot copy the picture.