- #1
Michael_Light
- 113
- 0
Homework Statement
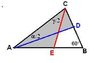
Given a triangle ABC with ∠ B = 60°. The bisectors of angle A and C intersect BC and AB at D and E respectively. Prove that CD + AE = AC.
Homework Equations
The Attempt at a Solution
I stuck on this question for hours already... what is the trick of proving this? Please help me.