- #1
Reverend Shabazz
- 19
- 1
Hello all,
I am reading through the Jackson text as a hobby and have reached a question regarding the Hamiltonian transformation properties. I will paste the relevant section from the text below:
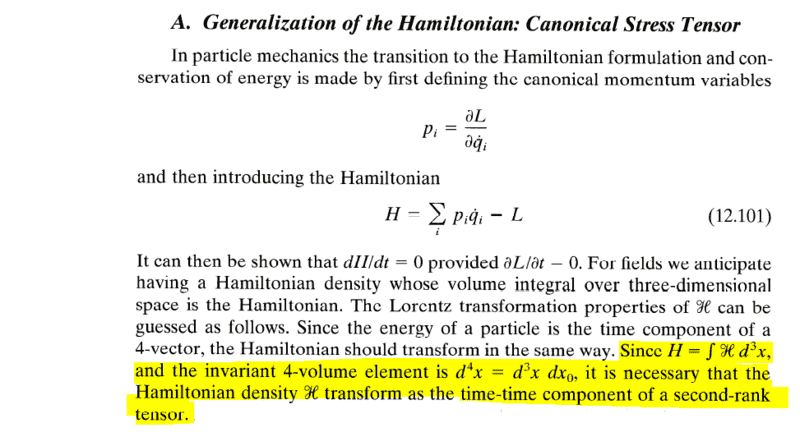
I don't understand what he's getting at in the sentence I highlighted.
To attempt to see what he is saying, I tried to show that by transforming the Hamiltonian density ##\mathcal {H} -> \mathcal {H'}## and multiplying it by the transformed 3-d volume element ##d'^3x##, the resulting Hamiltonian ##H'=\mathcal {H'}*d'^3x## is basically equivalent to the time component of the transformed four-momentum, but it did not equate (I used a simple case of constant velocity in the ##x## direction with ##E##&##B## fields in the ##y## and ##z## directions).
Could someone please provide some insight into this problem?
Thank you much
I am reading through the Jackson text as a hobby and have reached a question regarding the Hamiltonian transformation properties. I will paste the relevant section from the text below:
I don't understand what he's getting at in the sentence I highlighted.
To attempt to see what he is saying, I tried to show that by transforming the Hamiltonian density ##\mathcal {H} -> \mathcal {H'}## and multiplying it by the transformed 3-d volume element ##d'^3x##, the resulting Hamiltonian ##H'=\mathcal {H'}*d'^3x## is basically equivalent to the time component of the transformed four-momentum, but it did not equate (I used a simple case of constant velocity in the ##x## direction with ##E##&##B## fields in the ##y## and ##z## directions).
Could someone please provide some insight into this problem?
Thank you much
