- #1
scissors
- 22
- 0
1.) I have a diagram of a simple circuit, a square wave generator (Vin) a resistance R, and capacitance C.
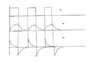
Then the square wave function of Vin vs t (10^-4 sec) is shown.
Vo starts at a max, goes down at 0.5 stays down till 1.0, and then goes up to the max, goes down at 1.5.
Now I have R = 1 kOHMs an C = .01 microFarads, and I am to sketch a graph of Vc versus time and Vr versus time over these same intervals.
I am using the equation Vc = Vo(1-e^-t/RC), but is this correct for a square wave? And what is the equation for Vr? My notes don't have one, but I'm sure it would be somewhat similar to Vc.
I have a second question, but I'm going to try to read my notes once again before posting that. Once again, thanks for any assistance!
Then the square wave function of Vin vs t (10^-4 sec) is shown.
Vo starts at a max, goes down at 0.5 stays down till 1.0, and then goes up to the max, goes down at 1.5.
Now I have R = 1 kOHMs an C = .01 microFarads, and I am to sketch a graph of Vc versus time and Vr versus time over these same intervals.
I am using the equation Vc = Vo(1-e^-t/RC), but is this correct for a square wave? And what is the equation for Vr? My notes don't have one, but I'm sure it would be somewhat similar to Vc.
I have a second question, but I'm going to try to read my notes once again before posting that. Once again, thanks for any assistance!