- #1
Timtam
- 42
- 0
Hi does anyone know a way to calculate the Minor losses related just to flow Geometry isolated from Major frictional losses, all the k tables I can find combine the frictional losses with the geometry losses eg see below blurb from
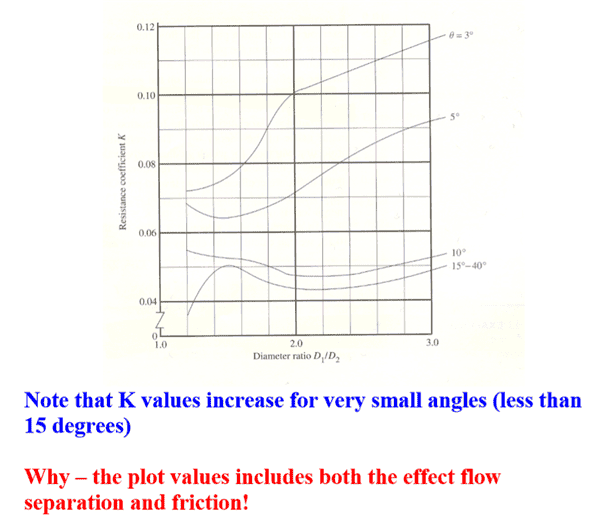
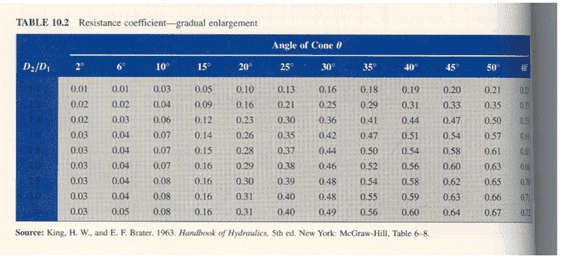

but I was hoping to obtain a first principles formula that could equate these losses solely to area and velocity change
The reason being is I am assuming that major losses component of the K values must be based on particular viscosity fluid but I would like to calculate this for different viscosity fluids ?
Also for the Darcy Weisbach if I want to calculate just the frictional losses would I use the average diameter of a convergent or divergent section of pipe ?
If such a formula exists it ok to just plain add these two effects ?
Am I making life to difficult for myself is there an easier way ?
but I was hoping to obtain a first principles formula that could equate these losses solely to area and velocity change
The reason being is I am assuming that major losses component of the K values must be based on particular viscosity fluid but I would like to calculate this for different viscosity fluids ?
Also for the Darcy Weisbach if I want to calculate just the frictional losses would I use the average diameter of a convergent or divergent section of pipe ?
If such a formula exists it ok to just plain add these two effects ?
Am I making life to difficult for myself is there an easier way ?