- #1
PhDeezNutz
- 693
- 440
- Homework Statement
- More of a conceptual question than a homework question. Maximizing radiation southward and minimizing it northward.
- Relevant Equations
- (See picture in attempt at solution)
For two antennas separated along the north-south direction, operating with the same amplitude ##E_0##, same wavelength ##\lambda##, separated by a distance ##d##, and with a phase difference ##\delta## the formula for radiated intensity is
$$I = 4I_0 \cos^2 \left(\pi \frac{d}{\lambda}\sin \theta + \frac{\delta}{2} \right)$$
Homework Statement: More of a conceptual question than a homework question. Maximizing radiation southward and minimizing it northward.
Homework Equations: (See picture in attempt at solution)
For two antennas separated along the north-south direction, operating with the same amplitude ##E_0##, same wavelength ##\lambda##, separated by a distance ##d##, and with a phase difference ##\delta## the formula for radiated intensity is
$$I = 4I_0 \cos^2 \left(\pi \frac{d}{\lambda}\sin \theta + \frac{\delta}{2} \right)$$
I'm going off these notes
https://scholar.harvard.edu/files/schwartz/files/lecture18-antennas.pdf
which uses this picture
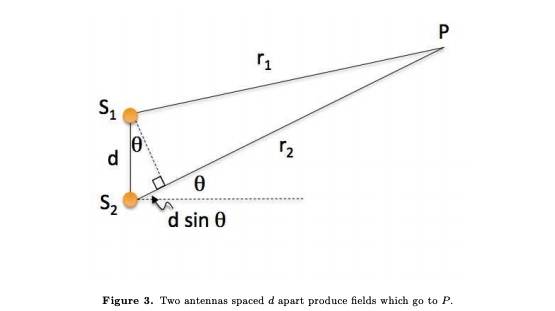
My attempt at a solution is as follows, and it seems to corroborate what they are saying. That said, I will challenge it at the end on the grounds of rotational symmetry.
At the very least we want ##I\left( \theta = \frac{\pi}{2} \right) = 0## and ##I\left( \theta = \frac{3\pi}{2} \right) = 4I_0##
$$4I_0 \cos^2 \left( \pi \frac{d}{\lambda} + \frac{\delta}{2} \right) = 0$$
$$4I_0 \cos^2 \left( -\pi \frac{d}{\lambda} + \frac{\delta}{2} \right) = 0$$
Then we have
$$\pi \frac{d}{\lambda} + \frac{\delta}{2} = \frac{\pi}{2}$$
$$-\pi \frac{d}{\lambda} + \frac{\delta}{2} = 0$$
Therefore
$$\delta = \frac{\pi}{2}$$
substituting back in we find
$$d = \frac{\lambda}{4}$$
This answer corroborates their answer on the bottom of page 6/ top of page 7. Their graph is
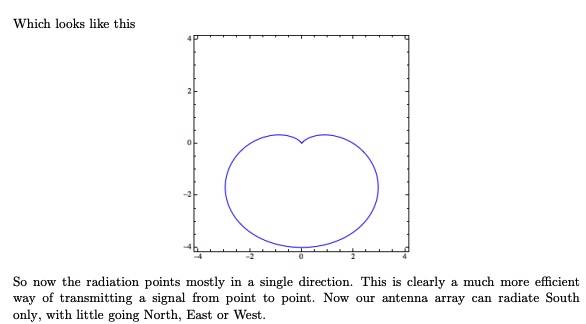
Mathematically everything checks out but I'm challenging it on physical grounds. Intuitively you think you would get the same interference pattern in both directions; If your rotate this system 180 degrees it should be the same system.
Can someone help me reconcile this?
As always, any help is appreciated.
Homework Equations: (See picture in attempt at solution)
For two antennas separated along the north-south direction, operating with the same amplitude ##E_0##, same wavelength ##\lambda##, separated by a distance ##d##, and with a phase difference ##\delta## the formula for radiated intensity is
$$I = 4I_0 \cos^2 \left(\pi \frac{d}{\lambda}\sin \theta + \frac{\delta}{2} \right)$$
I'm going off these notes
https://scholar.harvard.edu/files/schwartz/files/lecture18-antennas.pdf
which uses this picture
My attempt at a solution is as follows, and it seems to corroborate what they are saying. That said, I will challenge it at the end on the grounds of rotational symmetry.
At the very least we want ##I\left( \theta = \frac{\pi}{2} \right) = 0## and ##I\left( \theta = \frac{3\pi}{2} \right) = 4I_0##
$$4I_0 \cos^2 \left( \pi \frac{d}{\lambda} + \frac{\delta}{2} \right) = 0$$
$$4I_0 \cos^2 \left( -\pi \frac{d}{\lambda} + \frac{\delta}{2} \right) = 0$$
Then we have
$$\pi \frac{d}{\lambda} + \frac{\delta}{2} = \frac{\pi}{2}$$
$$-\pi \frac{d}{\lambda} + \frac{\delta}{2} = 0$$
Therefore
$$\delta = \frac{\pi}{2}$$
substituting back in we find
$$d = \frac{\lambda}{4}$$
This answer corroborates their answer on the bottom of page 6/ top of page 7. Their graph is
Mathematically everything checks out but I'm challenging it on physical grounds. Intuitively you think you would get the same interference pattern in both directions; If your rotate this system 180 degrees it should be the same system.
Can someone help me reconcile this?
As always, any help is appreciated.