- #1
Benny
- 584
- 0
Can someone please help me out with the following question?
Q. A simple harmonic oscillator, of mass m and natural frequency w_0, experiences an oscillating driving force f(t) = macos(wt). Therefore its equation of motion is:
[tex]\frac{{d^2 x}}{{dt^2 }} + \omega _0 ^2 x = a\cos \left( {\omega t} \right)[/tex]
Given that at t = 0 we have x = dx/dt = 0, find the function x(t). Describe the solution if w is approximately, but not exactly, equal to w_0.
I got:
[tex]y\left( t \right) = \frac{a}{{\left( {\omega _0 ^2 - \omega ^2 } \right)}}\left( {\cos \left( {\omega t} \right) - \cos \left( {\omega _0 t} \right)} \right)[/tex]
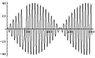
The answer says a couple of things about the behaviour of the solution for w ~ w_0 but I can't figure out how they got it. For instance "for large t it shows beats of maximum amplitude 2((w_0)^2 - w^2)^-1." How is that deduced and how would I determine which are the main characterstics of motion that I need to note. Any help would be appreciated.
Q. A simple harmonic oscillator, of mass m and natural frequency w_0, experiences an oscillating driving force f(t) = macos(wt). Therefore its equation of motion is:
[tex]\frac{{d^2 x}}{{dt^2 }} + \omega _0 ^2 x = a\cos \left( {\omega t} \right)[/tex]
Given that at t = 0 we have x = dx/dt = 0, find the function x(t). Describe the solution if w is approximately, but not exactly, equal to w_0.
I got:
[tex]y\left( t \right) = \frac{a}{{\left( {\omega _0 ^2 - \omega ^2 } \right)}}\left( {\cos \left( {\omega t} \right) - \cos \left( {\omega _0 t} \right)} \right)[/tex]
The answer says a couple of things about the behaviour of the solution for w ~ w_0 but I can't figure out how they got it. For instance "for large t it shows beats of maximum amplitude 2((w_0)^2 - w^2)^-1." How is that deduced and how would I determine which are the main characterstics of motion that I need to note. Any help would be appreciated.
Last edited: