- #1
bolbteppa
- 309
- 41
Given Cartesian [itex](x,y,z)[/itex], Spherical [itex](r,\theta,\phi)[/itex] and parabolic [itex](\varepsilon , \eta , \phi )[/itex], where
[tex]\varepsilon = r + z = r(1 + \cos(\theta)) \\\eta = r - z = r(1 - \cos( \theta ) ) \\ \phi = \phi [/tex]
why is it obvious, looking at the pictures
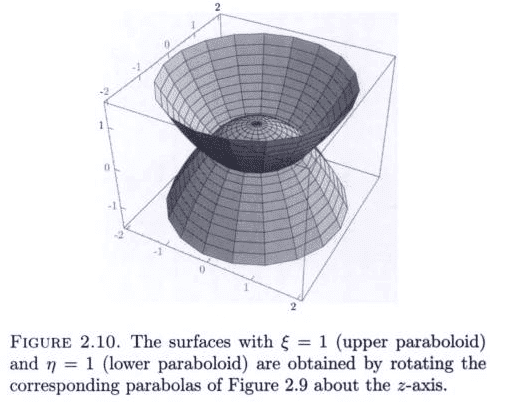
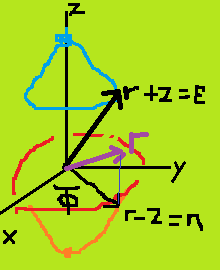
(Is my picture right or is it backwards/upside-down?)
that [itex]x[/itex] and [itex]y[/itex] contain a term of the form [itex]\sqrt{ \varepsilon \eta }[/itex] as the radius in
[tex]x = \sqrt{ \varepsilon \eta } \cos (\phi) \\ y = \sqrt{ \varepsilon \eta } \sin (\phi) \\ z = \frac{\varepsilon \ - \eta}{2}[/tex]
I know that [itex] \varepsilon \eta = r^2 - r^2 \cos^2(\phi) = r^2 \sin^2(\phi) = \rho^2[/itex] ([itex]\rho[/itex] the diagonal in the x-y plane) implies [itex] x = \rho \cos(\phi) = \sqrt{ \varepsilon \eta } \cos (\phi) [/itex] mathematically, but looking at the picture I have no physical or geometrical intuition as to why [itex] \rho = \sqrt{ \varepsilon \eta } [/itex].
[tex]\varepsilon = r + z = r(1 + \cos(\theta)) \\\eta = r - z = r(1 - \cos( \theta ) ) \\ \phi = \phi [/tex]
why is it obvious, looking at the pictures
(Is my picture right or is it backwards/upside-down?)
that [itex]x[/itex] and [itex]y[/itex] contain a term of the form [itex]\sqrt{ \varepsilon \eta }[/itex] as the radius in
[tex]x = \sqrt{ \varepsilon \eta } \cos (\phi) \\ y = \sqrt{ \varepsilon \eta } \sin (\phi) \\ z = \frac{\varepsilon \ - \eta}{2}[/tex]
I know that [itex] \varepsilon \eta = r^2 - r^2 \cos^2(\phi) = r^2 \sin^2(\phi) = \rho^2[/itex] ([itex]\rho[/itex] the diagonal in the x-y plane) implies [itex] x = \rho \cos(\phi) = \sqrt{ \varepsilon \eta } \cos (\phi) [/itex] mathematically, but looking at the picture I have no physical or geometrical intuition as to why [itex] \rho = \sqrt{ \varepsilon \eta } [/itex].