Divergence Theorem in Curvilinear Coordinates: Questions & Explanations

[Master1022]

Homework Statement

Prove the divergence theorem in curvilinear coordinates

Relevant Equations

Vector identities

Hi,

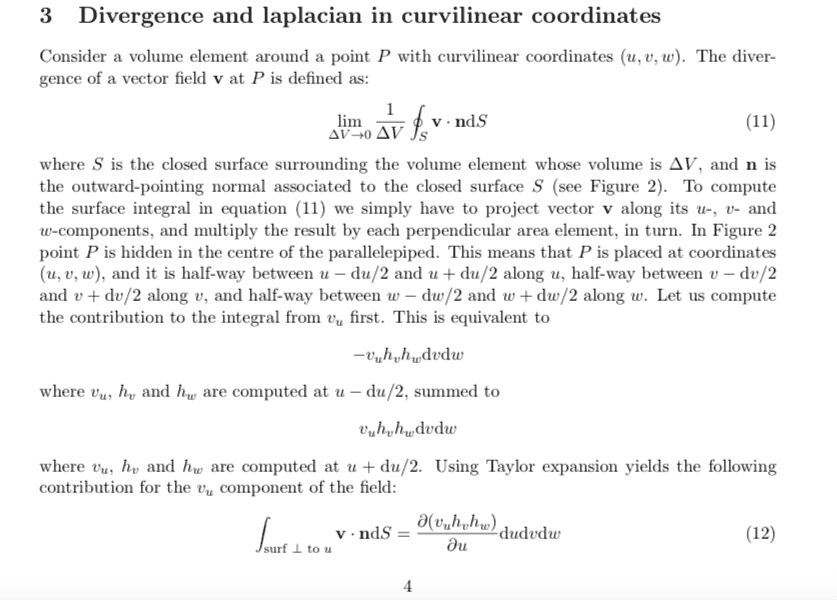
I was trying to gain an understanding of a proof of the divergence theorem in curvilinear coordinates. I have found these online notes here and am looking at the proof on pages 4-5. The method intuitively makes sense to me as opposed to other proofs which fiddle around with vector identities to get the required expression, however I have two main questions:

1) Why are they using lengths $du$ instead of $h_u du$? I thought that in curvilinear coordinates, we would have something like this for the u-direction (I put my centre at the bottom left corner of the box):
$$ (v_u) \cdot (-h_v h_w dw dv) + (v_u + \frac{\partial v_u}{\partial u} (h_u du)) \cdot (h_v h_w dv dw) $$

Current thoughts on why I might be wrong:
- In the grad formula in curvilinear coordinates, each component includes a factor of the reciprocal of the metric coefficient. Should I be including that here? I.e. should I have $\frac{1}{h_u} \frac{\partial}{\partial u}$ which would cancel out the $h_u$?

2) In equation (12), how did the metric coefficients $h_v$ and $h_w$ end up inside the partial derivative? From my expression above, the metric coefficients are outside the partial derivative. Given that each metric coefficients is, in a generalized sense, a function of the three variables $u$, $v$, and $w$, then how can we treat $h_v$ and $h_w$ as constants and move them around?

I probably have some basic understanding errors and any help would be greatly appreciated.

Attached below are images from the notes:

 

[lippyka]

Thanks,The first thing to note is that in curvilinear coordinates, the length of a line element is given by the metric components:$$ ds^2 = h_u^2 du^2 + h_v^2 dv^2 + h_w^2 dw^2 $$So when you are dealing with an integral over a region, you need to take this into account. The reason they are using lengths $du$ instead of $h_u du$ is because they are actually integrating over a region in physical space, not in coordinate space. This means that the area of the region needs to be taken into account. In other words, if you were to map the region in physical space onto the coordinate space, then the area of the region in physical space would be different from the area of the region in coordinate space.For the second question, the metric coefficients are treated as constants because they are assumed to be independent of the variables $u$, $v$, and $w$. In other words, the metric coefficients are assumed to be a function of the coordinates $(u, v, w)$ only, and not of any partial derivatives. Therefore, we can move them around freely.