- #1
srcs
- 4
- 1
This question occurred to me a few days ago and it's been bugging me ever since.
Consider a circle in the coordinate plane with center P(x,y) and radius R, where R < D, D being the distance from the origin to the circle's center.
There are two lines tangent to the circle (T1 and T2) that pass through the origin.
The circle's location can also be considered as a line segment of radius D and some angle Θ with the x axis.
How can I find a general equation in terms of x, y, R, and Θ to determine the angle each tangent line makes with the x axis?

Solution found:
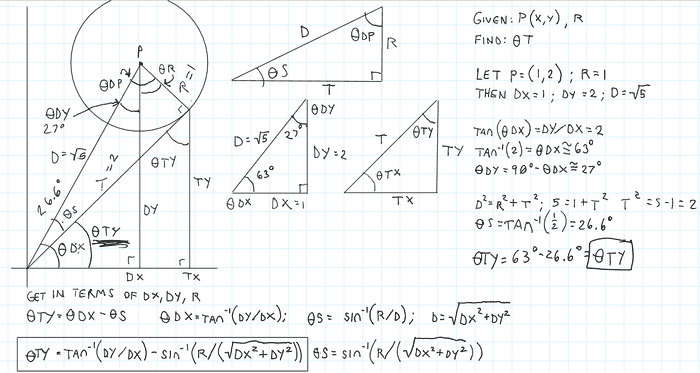
Adding Θs to Θdx should yield the bearing for the other tangent line.
Consider a circle in the coordinate plane with center P(x,y) and radius R, where R < D, D being the distance from the origin to the circle's center.
There are two lines tangent to the circle (T1 and T2) that pass through the origin.
The circle's location can also be considered as a line segment of radius D and some angle Θ with the x axis.
How can I find a general equation in terms of x, y, R, and Θ to determine the angle each tangent line makes with the x axis?
Solution found:
Adding Θs to Θdx should yield the bearing for the other tangent line.
Last edited: