Solve Equation: Get from One to Two?

[rnielsen25]

Hi everybody.

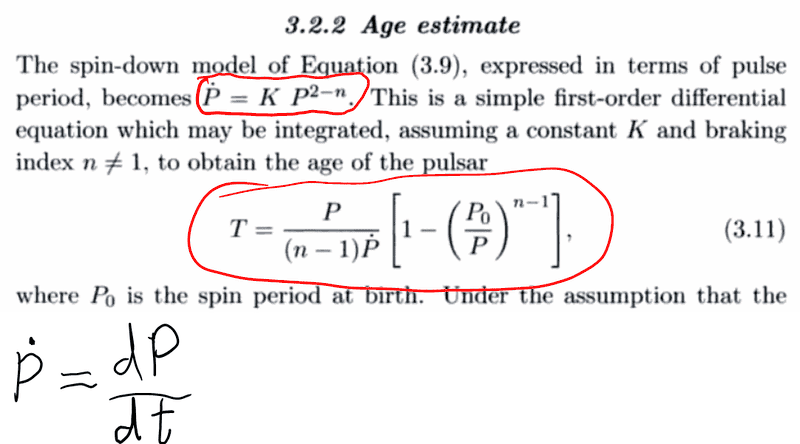
How do you get from equation one to equation two?
For me it doesn't make any sense. If you separate both variables and integrate on both sides, how come you get anything like in equation two? And wouldn't it still be a differential equation since dP/dt still appears in the solution. If anyone happen to know the answer, please help me out.

The reason why I'm asking, is because i want to derive the second formula, which should be done by integrating the differential equation, as they state in the book.

 

[andrewkirk]

First rewrite the DE as $\frac{dP}{dt}=KP^{2-n}$. Then use separation of variables to rewrite this as $P^{n-2}dP=Kdt$. Then integrate both sides.

 

[rnielsen25]

First rewrite the DE as $\frac{dP}{dt}=KP^{2-n}$. Then use separation of variables to rewrite this as $P^{n-2}dP=Kdt$. Then integrate both sides.

Yes. But when you integrate both sides, then you'll get $\frac{P^{n-1}}{n-1}=kt+c_1$
But i cannot see how this relates to the second formula? Could you help me moving on from here?

 

[rnielsen25]

I might have to explain what the formula is describing.
The second equation is describing the age of a pulsar, which is a rotating neutron star, where P is the period of the rotation, and dP/dt is off course the change in period. These neutron stars emit energy by spinning and therefore slows down. By assuming the neutron star is a rotating magnetic dipole, then the change in period is equally to the expression in the first equation.
By solving this differential equation, you should get the age of the neutron star.

 

[rnielsen25]

First rewrite the DE as $\frac{dP}{dt}=KP^{2-n}$. Then use separation of variables to rewrite this as $P^{n-2}dP=Kdt$. Then integrate both sides.

All right, i think I'm on the right track.
I've found this example instead, where ohm is equally to the angular velocity.

I'm going to replace ohm with $\omega$
So what i think they're doing is they have the following equation:

$\frac{d\omega}{dt}=-k\omega^{n}$

By seperating the variables you get the following:

$\omega^{-n}d\omega=-kdt$

Here is what i think they're doing, which is here i need your help about math.
Instead of just integrating normally, they're making a finite integral. But isn't it wrong to do that, when solving a differential equation.

$\int^{\omega}_{\omega_0}(\omega^{-n})d\omega=\int^{\tau}_{0}(-k)dt$
This would explain why the extra constant is missing, and it does also explain, why a $\omega_0$ appears. What do you think?

Or could you integrate normally, and then transform the new constant $c_1$ into $\omega_0$ somehow?

 

[rnielsen25]

$\int^{\omega}_{\omega_0}(\omega^{-n})d\omega=\int^{\tau}_{0}(-k)dt$

The solution would be, if n has to be 3:

$- \frac{\omega^{1-n}-\omega_0^{1-n}}{n-1}=-k\tau$

If you multiply with -1 on both sides, you will se the upper solution, indeed is the same as in the picture.

But what if you tried to solve it with non finite integrals.
The solution would be like this, where t probably is the same as $\tau$, the age of the pulsar.
But what about $c_1$ could you turn that into $\omega_0$ somehow?
$- \frac{\omega^{1-n}}{n-1}=-kt+c_1$

You could say that $c_1=\frac{\omega_0^{1-n}}{n-1}$

But i do prefer the other way, because i then could explain i was integrating from the angular velocity from birth to it's present angular velocity. And i could say i was integrating from time 0, when it was born to the present time, which must be it's age.

 

[rnielsen25]

But assuming using finite integrals is okay. Why do they replace k with $\frac{d\omega}{dt}$ but that is probably more a specialized physicist in this field i have to ask?

 

[Mark44]

Please stop bumping your posts. Per forum rules, members aren't allowed to bump their post sooner than 24 hours.

Here is what i think they're doing, which is here i need your help about math.
Instead of just integrating normally, they're making a finite integral.

The correct term is definite integral, not finite integral. A definite integral has limits of integration -- $\omega_0$ and $\omega$ in the integral below.
An integral with no limits of integration is an indefinite integral.

But isn't it wrong to do that, when solving a differential equation.

$\int^{\omega}_{\omega_0}(\omega^{-n})d\omega=\int^{\tau}_{0}(-k)dt$

No, it's not. Some authors of textbooks on differential equations routinely use this type of integral.

This would explain why the extra constant is missing, and it does also explain, why a $\omega_0$ appears. What do you think?

 

[rnielsen25]

Please stop bumping your posts. Per forum rules, members aren't allowed to bump their post sooner than 24 hours. The correct term is definite integral, not finite integral. A definite integral has limits of integration -- $\omega_0$ and $\omega$ in the integral below.
An integral with no limits of integration is an indefinite integral.
No, it's not. Some authors of textbooks on differential equations routinely use this type of integral.

All right thanks. Could you also help me out understanding, what happens in equation 13,37?
Especially what happens with the constant, and why $\frac{d\Omega}{dt}$ appears.

 

[Mark44]

All right thanks. Could you also help me out understanding, what happens in equation 13,37?
Especially what happens with the constant, and why $\frac{d\Omega}{dt}$ appears.

Eq. 13.37 is
$$\tau = \frac{\Omega^{n - 1}}{\kappa(n - 1)} = -\frac{\Omega}{(n - 1)\dot{\Omega}} = \frac{P}{(n - 1)\dot{P}}$$
This is pretty much an exercise in algebra. Do you see how the first part of this equation comes from Eq. 13.36, together with the assumption that $\Omega_0 >> \Omega$? The symbol >> means "is much greater than."
The second part comes from the diff. equation $\dot{\Omega} = \kappa \Omega^n$. I haven't read what you posted closely enough to see where the last part comes from, but I would guess it's pretty straightforward.

 

[rnielsen25]

Eq. 13.37 is
$$\tau = \frac{\Omega^{n - 1}}{\kappa(n - 1)} = -\frac{\Omega}{(n - 1)\dot{\Omega}} = \frac{P}{(n - 1)\dot{P}}$$
This is pretty much an exercise in algebra. Do you see how the first part of this equation comes from Eq. 13.36, together with the assumption that $\Omega_0 >> \Omega$? The symbol >> means "is much greater than."
The second part comes from the diff. equation $\dot{\Omega} = \kappa \Omega^n$. I haven't read what you posted closely enough to see where the last part comes from, but I would guess it's pretty straightforward.

I didn't thought about solving k in the diff. equation, and replacing it in that way. Thanks for the help. I think i understand everything now, so i will probably close the thread now.