- #1
chickens
- 29
- 0
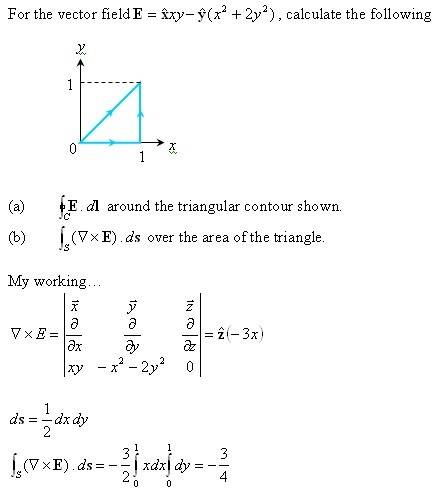
Hi guys... I'm stuck in this question. Its not a homework nor coursework, just practice. The answer for both is the same, that is -1 ...I'm able to get (a) but (b) ... still wondering what went wrong. Please enlighten me. Thanks in advance.
Last edited: