- #1
Saitama
- 4,243
- 93
Homework Statement
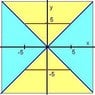
If the distance of any point (x,y) from the origin is defined as
d (x, y) = max {|x|,|y|},
d (x, y) = a non zero constant, then the locus is
(a) a circle
(b) a straight line
(c) a square
(d) a triangle
Homework Equations
The Attempt at a Solution
I don't understand what does the question mean by "d (x, y) = max {|x|,|y|}"?
Can somebody tell what does the notation mean? I never encountered problems like these.
Any help is much appreciated.
Thanks!