- #1
Math Amateur
Gold Member
MHB
- 3,990
- 48
I am reading Tom M Apostol's book "Mathematical Analysis" (Second Edition) ...
I am focused on Chapter 12: Multivariable Differential Calculus ... and in particular on Section 12.9: The Chain Rule ... ...
I need help in order to fully understand Theorem 12.7, Section 12.9 ...
Theorem 12.7 (including its proof) reads as follows:
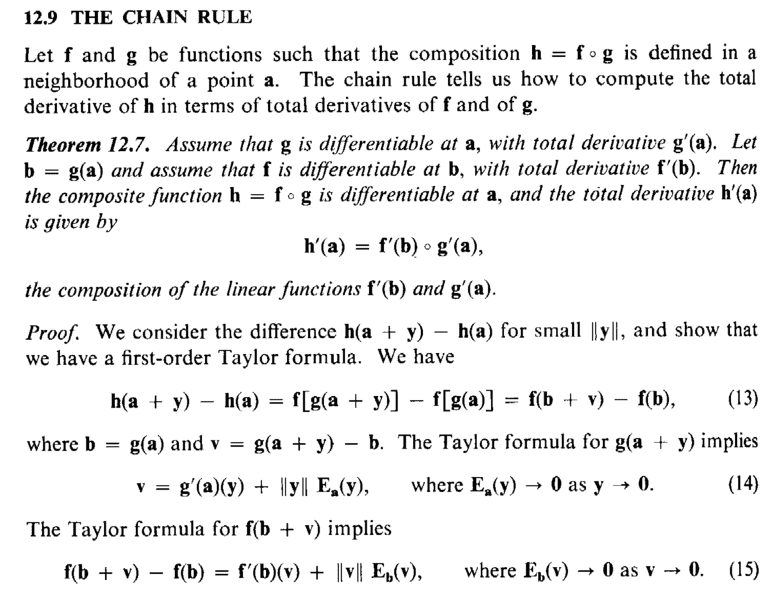
In the proof of Theorem 12.7 we read the following:
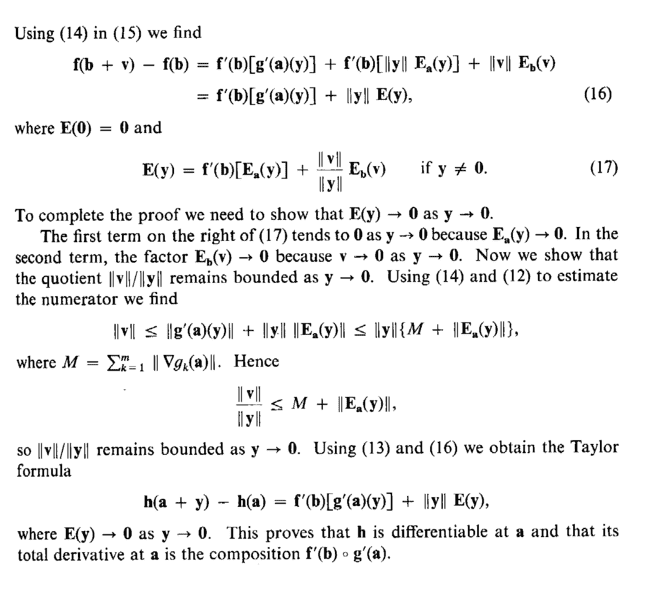
" ... ... Using (14) in (15) we find
##f(b+v) - f(b) = f'(b) [ g'(a) (y) ] + f'(b) [ \| y \| E_a(y) ] + \|v \| E_b(v)####= f'(b) [ g'(a) (y) ] + \| y \| E(y)## ... ... ... (16)Where ##E(0) = 0## and##E(y) = f'(b) [ E_a(y) ] + \frac{ \| v \| }{ \| y \| } E_b (v) \ \ \ \ \text{ if } y\neq 0## ... ... ... (17)... ... ... "
*** EDIT ***
It now occurs to me that in fact Apostol is defining E(y) in equations (16) and (17)
I should have seen this earlier ...
Peter
================================================================My questions are as follows:Question 1
Can someone show how Equation (16) follows ... that is ...
... how exactly does ##f(b+v) - f(b) = f'(b) [ g'(a) (y) ] + \| y \| E(y)##
follow from
##f(b+v) - f(b) = f'(b) [ g'(a) (y) ] + f'(b) [ \| y \| E_a(y) ] + \|v \| E_b(v)##?
Question 2
What is ##E## ... I know what ##E_a## and ##E_b## are ... but what is ##E##?
Similarly ... what is ##E(y)## in (16) and in (17) ... shouldn't it be ##E_a(y)## ... ?
Further ... why (formally and rigorously) does ##E(0) = 0##
Question 3
Can someone please demonstrate how/why
##E(y) = f'(b) [ E_a(y) ] + \frac{ \| v \| }{ \| y \| } E_b (v)##
Help will be appreciated ...
Peter
=========================================================================================
It may help Physics Forum readers of the above post to have access to Apostol's section on the Total Derivative ... so I am providing the same ... as follows:

 Hope that helps ...
Hope that helps ...
Peter
I am focused on Chapter 12: Multivariable Differential Calculus ... and in particular on Section 12.9: The Chain Rule ... ...
I need help in order to fully understand Theorem 12.7, Section 12.9 ...
Theorem 12.7 (including its proof) reads as follows:
In the proof of Theorem 12.7 we read the following:
" ... ... Using (14) in (15) we find
##f(b+v) - f(b) = f'(b) [ g'(a) (y) ] + f'(b) [ \| y \| E_a(y) ] + \|v \| E_b(v)####= f'(b) [ g'(a) (y) ] + \| y \| E(y)## ... ... ... (16)Where ##E(0) = 0## and##E(y) = f'(b) [ E_a(y) ] + \frac{ \| v \| }{ \| y \| } E_b (v) \ \ \ \ \text{ if } y\neq 0## ... ... ... (17)... ... ... "
*** EDIT ***
It now occurs to me that in fact Apostol is defining E(y) in equations (16) and (17)
I should have seen this earlier ...
Peter
================================================================My questions are as follows:Question 1
Can someone show how Equation (16) follows ... that is ...
... how exactly does ##f(b+v) - f(b) = f'(b) [ g'(a) (y) ] + \| y \| E(y)##
follow from
##f(b+v) - f(b) = f'(b) [ g'(a) (y) ] + f'(b) [ \| y \| E_a(y) ] + \|v \| E_b(v)##?
Question 2
What is ##E## ... I know what ##E_a## and ##E_b## are ... but what is ##E##?
Similarly ... what is ##E(y)## in (16) and in (17) ... shouldn't it be ##E_a(y)## ... ?
Further ... why (formally and rigorously) does ##E(0) = 0##
Question 3
Can someone please demonstrate how/why
##E(y) = f'(b) [ E_a(y) ] + \frac{ \| v \| }{ \| y \| } E_b (v)##
Help will be appreciated ...
Peter
=========================================================================================
It may help Physics Forum readers of the above post to have access to Apostol's section on the Total Derivative ... so I am providing the same ... as follows:
Peter
Attachments
-
 Apostol - 1 - Theorem 12.7 - Chain Rule - PART 1 ... .png43.4 KB · Views: 1,045
Apostol - 1 - Theorem 12.7 - Chain Rule - PART 1 ... .png43.4 KB · Views: 1,045 -
 Apostol - 2 - Theorem 12.7 - Chain Rule - PART 2 ... ... .png31 KB · Views: 1,120
Apostol - 2 - Theorem 12.7 - Chain Rule - PART 2 ... ... .png31 KB · Views: 1,120 -
 Apostol - 1 - Section 12.4 - PART 1 ... .png44.6 KB · Views: 433
Apostol - 1 - Section 12.4 - PART 1 ... .png44.6 KB · Views: 433 -
 Apostol - 2 - Section 12.4 - PART 2 ... .png39.7 KB · Views: 444
Apostol - 2 - Section 12.4 - PART 2 ... .png39.7 KB · Views: 444 -
 ?temp_hash=94e326edac58a0ed69338d46334d19ae.png43.4 KB · Views: 603
?temp_hash=94e326edac58a0ed69338d46334d19ae.png43.4 KB · Views: 603 -
 ?temp_hash=94e326edac58a0ed69338d46334d19ae.png31 KB · Views: 552
?temp_hash=94e326edac58a0ed69338d46334d19ae.png31 KB · Views: 552 -
 ?temp_hash=94e326edac58a0ed69338d46334d19ae.png44.6 KB · Views: 424
?temp_hash=94e326edac58a0ed69338d46334d19ae.png44.6 KB · Views: 424 -
 ?temp_hash=94e326edac58a0ed69338d46334d19ae.png39.7 KB · Views: 411
?temp_hash=94e326edac58a0ed69338d46334d19ae.png39.7 KB · Views: 411
Last edited: