Exploring the Expansion of Spacetime: A 3.5D Perspective

[Faradave]

I'm a layman who’s spent a few weeks working through the FAQs and the two substantial sticky threads (most helpful, highly recommended). In order to avoid distraction, I have copied this post from its original location.

When we speak of “the universe”, it is important to acknowledge that we refer to all space (and its contents) at a given instant. (More specifically, we refer to all space for a set of comoving particles.) But the universe “now” applies to just one layer of space within spacetime. This occurs between adjacent layers for the immediate past and the immediate future.

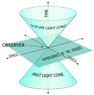
Though the "reality" of it is disputable, it seems undeniable that, with respect to the balloon analogy (BA), time is radial. There is no other reasonable place for it in the model, we are not disavowing time, so it is completely within reason to ask where time belongs.

The whole purpose of the BA is to illustrate the expansion of space. In admitting this, we must concede that the balloon will be bigger at all future times and smaller at all past times. Space, collected for these times, forms concentric, onion-like layers. The next layer out is always larger than the one before it, demonstrating expansion with no obvious limit. Going the other way however, there is a limit, at the center of the onion, which I think we must also admit, is the region of the Big Bang event (a.k.a. Singularity). The universe (now) does not contain the Big Bang event but spacetime must.

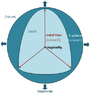
My diagram is adapted from one on the net (not a science site.) The balloon surface is a 3-sphere, a flat representation of all three spatial dimensions. One might imagine a school globe but, instead of an ordinary map on its surface imagine a hologram. Even though its flat, it shows all three dimensions (latitude, longitude and altitude, in this case).

 

[marcus]

Hi Dave,
have you watched Ned Wright's balloon model animation thru to the end?

It shows the balloon contracting as well as expanding.

A lot of today's cosmology research is going into Non-singular models, and one of the most common types studied is analogous to what you have with the balloon contracting (to a critical density where quantum effects briefly reverse the attraction) and rebounding into an expansion phase.

 

[Faradave]

Hi Marcus,
Thanks for your obvious dedication to the Cosmology Forum. I remember reading your posts about the “big bounce” in a sticky thread. Honestly, I am satisfied to work up from the assumption of “a beginning” right now. There will inevitably be questions regarding the start of the bounce. I was more intrigued by your comments:

… x-y-z coordinates as if that was how you imagine space! I think we got that idea from a book published in 1637 by René Descartes…you might find quite a bit of physics after 1850 puzzling…when Georg Riemann gave us the manifold… can be boundaryless and yet have finite volume, and it can be AUTONOMOUS and completely described by internal relationships. It does not have to be embedded inside a higher dimension manifold in order to exist.

I think the balloon analogy (BA) gives those who are willing to step away from the strict Cartesian view a chance to simplify spacetime considerably. We most often encounter spacetime in a conventional 3+1 dimensionality. But this makes time the “odd man” out so to speak. It can be different with a radial-time BA. Time can be the main player.

I begin by considering the apparent differences between the elements of space and of time. Because we experience back and forth translational freedom in space, it is appropriate to depict a spatial element as a Cartesian coordinate. Space is line-like, extending indefinitely in opposite directions. Such displacements may be occur in three ways independently (displacement along one dimension does not require displacement along the other two), thus "3D".

Time, by contrast, seems to have an origin in the singularity. Time is ray-like. It permits only non-negative (forward-only) displacement. What makes it interesting is that these rays may be modeled in a temporal field surrounding the singularity in 4D which comprises makes the entire manifold! We lose the need for a separate “space”.

In that sense, space can be thought completely derivative of time. A location in “spacetime” could be specified by three independent angles from some arbitrary time ray, and a radial distance (a time). It’s a polar coordinate model in which space is any continuous set of locations equidistant from the singularity. Each concentric arc represents a simultaneous space, most conveniently depicted as a 3-sphere, centered on the singularity. Space becomes an abstraction, quite amenable to the rigors of Cosmology and Relativity.

 

[Faradave]

(NASA/WMAP Science Team).

In case a reader is not yet a fan of the radial time version of the balloon analogy (BA) consider this popular, horn-shaped depiction of spacetime. It has the Big Bang (BB) event at the left while time proceeds to the right and space expands (depicted by enlarging cross-sections). Two things to note:

1) I believe the timeline indicated at the bottom should be a right-pointing ray (rather than a double-arrowed line), as our only experience of time is forward. Indeed, the illustration is entitled “Time Line of the Universe” a geometric misnomer, but I don’t expect anyone will soon be calling it the “Time Ray of the Universe”. …Pity.

2) The horn is illustrated from the BB to our “present” time, but should be understood to grow (or deterministically preexist) to the right indefinitely.

3) Though the horn is nicely populated with images of celestial objects, each cross section is a 3-disk in which the volume of familiar objects is “flattened” in a way not clearly recognizable to us. To be sure, it's difficult to draw just the X,Y,Z coordinate axes (having both positive and negative extents) on a 3-disk and still maintain all their customary adjacencies.

4) The question is often asked, “What lies outside the horn?” An answer I found helpful is:

There does not have to be "space outside of space". Nor does there have to be a spatial boundary to the universe. ..it can exist as an autonomous mathematical object of study WITHOUT being embedded in any higher dimension manifold.

It should be understood that the region outside the horn is only necessary for illustration. No such embedding space exists in conventional 3+1 spacetime. Thus, for every cross-section (3-disk), what lies outside its apparent “circumference” is null, nothing, not even space. Which is to say, the circumference represents a single point, a “pole”. Pick any two points on the apparent circumference. There is nothing between them on the outside. Therefore they are adjacent. The 3-disk for a given time slice may be properly curved into a 3-sphere, yielding the “balloon” of the BA for the same moment.

You could imagine everting the horn into a 3-sphere in much the way a half mango is everted. More simply, the handles of a paper fan open to reveal a disk. In either case, time finds itself as radial, a temporal field radiating outward from the origin (like the ribs of the fan).

 

[someGorilla]

Haven't you been taking the balloon analogy too literally? No one knows if space is closed onto itself. The "horn" picture is usually meant to depict some convenient part of the universe, not all of it. Out of any circular (spherical) section of the horn there is just more space, only it's not represented in order to make the picture clear.

 

[Faradave]

Hello someGorilla,

Haven't you been taking the balloon analogy too literally?

I think it’s interesting to push the balloon analogy (BA) a bit to see what it will yield. So far, in reviewing the sticky threads, it's been quite a lot! Clearly, from your question, it’s important to remember that the BA is a model to illustrate Hubble expansion.

No one knows if space is closed onto itself.

While pursuing the BA, I would not deny the value of other models, such as a flat model of the universe. Even a balloon universe is essentially flat, locally. Look how far wave-particle duality has taken us, with mutually exclusive models. So let’s keep thinking about all the possibilities, even the possibility of a universe with negative curvature. I will admit a personal bias toward positive curvature as exhibited by the BA. If nature tends to self similarity, it’s hard to avoid the preponderance of spheres we have encountered.

The ‘horn’ picture is usually meant to depict some convenient part of the universe, not all of it.

That wouldn’t explain the horn's shape. Why not just a straight cylinder? The pinch on the left end clearly seems a region of rapid expansion from something like a singularity. Then its fairly steady for a long time with a flare (acceleration) on the right end, to the present. The picture of the cosmic background radiation (CBR) on the left, I believe represents the entire observable universe at least.

In any case, if you accept Hubble expansion of space, then “running the movie backward” gets you to a finite, even rather small beginning. I think finite space is a lot neater without edges (as a 3-sphere).

 

[Jorrie]

That wouldn’t explain the horn's shape. Why not just a straight cylinder? The pinch on the left end clearly seems a region of rapid expansion from something like a singularity. Then its fairly steady for a long time with a flare (acceleration) on the right end, to the present.

It is only a 'near-straight cylinder' in the middle because it is depicted on a log-log type scale, where power laws yield straight lines. It is not possible to show all the effects on a linear, or even a log-linear scale. As someGorilla hinted, that expanding 'tube' represents only the observable universe, which may be a very small part of a (possibly) finite whole.

Also, if the 'cylinder' does not expand, it must collapse, because zero expansion is unstable, even with dark energy. This is one of the areas where a simple balloon analogy fails, because it can in principle be kept static for ever.

In any case, if you accept Hubble expansion of space, then “running the movie backward” gets you to a finite, even rather small beginning. I think finite space is a lot neater without edges (as a 3-sphere).

Nope, Hubble expansion only says that the observable universe (probably) started with a finite size. The total could have been finite or infinite. Observations could this far not point in one direction or the other.

 

[Faradave]

Hi Jorrie,

Thanks for your valuable calculator. That was a nice piece of work.

I think we can agree that the cross-sections of the “Timeline of the Universe” horn (see thumbnail) are 3-disks (3D represented as flat). You’re correct that they represent the observable universe. To the extent that expansion can be assumed consistent throughout the whole universe, a 3-disk could also be imagined to represent the whole universe at a given time.

A 3-disk slice here depicts a flat universe, convenient to local experience. The question at hand is how to dispose of the non-existing region outside the horn. One way is to interpret each 3-disk as infinite, leaving no room for such a region. Another is to consider the edges of a disk to be in contact, a single point, a pole on the expanding balloon of the BA.

The solutions are in some ways the same. Riemann gives us the equivalence of a finite sphere and an infinite plane. (Works just as well with a finite 3-sphere and infinite 3-plane.) In the animation below, Riemann’s portrait and reflection reveal the one to one correspondence between the points on a finite sphere and the plane beneath it. If you imagine the plane extends forever, then its “edges” correspond to the north pole of the finite sphere. The north pole of the sphere is named “the point at infinity”. If Riemann's portrait goes to the north pole of the sphere, that part of it "appears" at infinity on the plane.

https://www.youtube.com/watch?v=6JgGKViQzbc

I personally happen to prefer the 3-sphere of the BA to a flat 3-plane. I hope to be able to show you in upcoming posts, at least two new (I think) compelling reasons for that.

 

[Jorrie]

Hi Faradave, glad you found the calculator useful.

The question at hand is how to dispose of the non-existing region outside the horn. One way is to interpret each 3-disk as infinite, leaving no room for such a region. Another is to consider the edges of a disk to be in contact, a single point, a pole on the expanding balloon of the BA.

I prefer to view the 'horn' as just one of (infinitely) many 'Riemann portraits' possible on the surface of the 'expanding balloon', i.e. our observable patch. I think it may be confusing to non-experts to attach a 'whole universe' meaning to the horn, especially with the sort of pictures like in the NASA/WMAP graphic that you have shown.

Nevertheless, I'll be interested to hear your other arguments for favoring the balloon analogy over other ones.

 

[Faradave]

On a 3-Surface, We’re All Flatlanders

I would like to address the 3-surface of the balloon analogy (BA). A 3-surface (a type of “hypersurface”) has the thickness of a plane and yet contains a compaction of three dimensions. Most consider a 3-surface a mathematical abstraction of utility in considering four dimensions, since it is difficult to draw or even imagine 4D objects.

So, in 4D Minkowski spacetime (M4), the time coordinate is vertical, while all of space is represented in a horizontal 3-plane (see 1^st thumbnail). For the expanding balloon surface of the BA, the 3-plane of space is curved as a 3-sphere.

It’s understandable that people consider the BA to be “just an analogy” because after all, it’s obvious that you and I are well integrated 3D objects. I’m going to make the case that the 3-plane is a reality. My goal in this is to strengthen our confidence in the utility of the BA to deliver additional insights. I’m not denying the normal 3D reality that we all perceive but I suggest that universal coexistence in a 3-surface is another of nature’s dualities.

My argument for the reality of the 3-surface is simple. You may take it or leave it. Rejecting it will not critically impair our further discussion of the BA. If you accept it, I think you’ll find your considerations of the BA somewhat enhanced.

Recall your last routine chest x-ray. You were positioned between an x-ray source and a detector (2^nd http://www.radiologyinfo.org/en/photocat/gallery3.cfm?pid=1&image=philip21.jpg&pg=chestrad). The x-rays entered one side of your body and, with some variation due to path density, left the other side to hit the detector. The x-rays encountered you as a 3D volume having height, width and depth. Images can be obtained this way from three independent directions.

Now consider how time sees you. Time is at once, perpendicular to your height, width and depth, having no extent along any of those. For example, you don’t age first, on one side of your body, then the other. All your particles age together. Time encounters you as a perfectly flat surface! All your elementary particles have essentially parallel (comoving) timelines. And since they are "simultaneous", the particles share the same 3-surface.

Some may require proof. As an organic life form, you have carbon distributed liberally throughout your composition. Some of that is a radioactive isotope ¹⁴C, with a characteristic half life. These atoms are tiny clocks and everywhere they occur in your body, they decay at exactly the same rate (i.e. have identical half lives).

From a within space perspective, you are an integrated 3D volume. From outside space (i.e. time’s perspective) you exist flat, on the BA 3-surface. Geometric duality!

 

[Jorrie]

Now consider how time sees you. Time is at once, perpendicular to your height, width and depth, having no extent along any of those. For example, you don’t age first, on one side of your body, then the other. All your particles age together. Time encounters you as a perfectly flat surface! All your elementary particles have essentially parallel (comoving) timelines. And since they are "simultaneous", the particles share the same 3-surface.

I think you must be more careful with your wording; e.g. "how time sees you" is essentially meaningless. Same for "Time encounters you as a perfectly flat surface!". That's apart from the fact that it not generally true that all parts of your body age together (have the same age). Here on Earth we are mostly in an upright position, so our heads age faster than our feet (sadly :-). The x-rays may be a bad choice of analogy.

It is not common practice to view the 'outside' and 'inside' of the balloon as the time dimension, but rather as representing the expansion factor (a), i.e. when the balloon radius has grown by 10%, the expansion factor has grown by 10%. Time is a rather more complex function of the radius.

Good as the balloon analogy may be to enhance understanding of basic principles, it may also create misconceptions if you take it too far - one common example is taking the curvature of the balloon surface as representing the curvature of space. They are actually quite unrelated.

 

[Faradave]

Hubble Bubble (HB)?

...careful with your wording; e.g. "how time sees you" ... "Time encounters you as a perfectly flat surface!"

True, but beside my main point. In Minkowski spacetime, time is normal to a flat spatial 3-plane. I assert that you and I (and all the universe) are mapped onto that plane, though in no simple manner. The hyperplane is a flat cross section of the horn (post #4).

It is not common practice to view the 'outside' and 'inside' of the balloon as the time dimension, ... rather more complex

Agreed. (Though, I consider it is actually a(t) which is more “complex” than its parameter.) All I am doing is taking the well-known Minkowski 3-plane (post #10) and curving it into a balloon-like sphere. That clearly makes time radial (and still normal to all points on the 3-surface). The 3-sphere inflates with time. If I need to give it a different name than "balloon analogy", OK. Perhaps I should call this a "Hubble Bubble" (HB)?

Good as the balloon analogy may be to enhance understanding of basic principles, it may also create misconceptions if you take it too far...

True again! But a simple device like HB is a useful tool in approaching some outstanding questions in cosmology. I need one more preparatory post before I can give an example. Then you can decide its worth.

Thanks for your vigilance.

 

[Faradave]

c the Light!

Something’s going to happen … something wonderful!

Experimental physicists have been providing increasingly accurate measures of lightspeed since 1675, to the point where it is now a standard measure. Maxwell, after assembling the equations of electricity and magnetism, was able to derive speed c mathematically. Einstein noted the frame independence of this measure and so used, c = c’ as a principle of nature (his second postulate) to derive special relativity (SR) and which holds true, even in the non-inertial frames of general relativity (GR).

In view of this, I am compelled consider another possible principle of nature:
For a phenomenon, such as speed limit c, to be universal throughout the cosmos (in terms of location, time and motion), it must be related to, thus derivable from, the underlying structure of the cosmos.

A structure I believe reveals this is the Hubble Bubble (HB, closely related to the balloon analogy see post #12 above and https://www.physicsforums.com/showthread.php?t=641966). Space is imagined as an expanding 3-sphere having radial time. A region of interest (see images) is depicted in cross section, as a curved-space “Hubble diagram”, analogous to the Minkowski diagram of flat spacetime. Here, two arcs represent a spatial dimension at times t₁ and t₂, separated by Δt. A particle at rest moves upward, along its radial timeline. Moving particles make an angle to this, which increases with velocity. Each higher velocity experiences a smaller temporal component. A maximum velocity naturally occurs tangent to its arc, as a direct consequence of the unidirectionality of time. In the HB, speed limit c does not restrict backward time travel. Conversely, it is the direction of time from which speed c derives.

That this universal V_max is, in fact, lightspeed is confirmed by experiment but is also evident in that V_max is non-aging. Perpendicular to its departing radius, this trajectory has no time component, as predicted by SR. This property is not evident from the 45° intervals arbitrarily ascribed to it in spatially-flat Minkowski diagrams. Though light does not age, observers measure speed c as the arc length on t₂ divided by apparent time Δt.

*We miss you Arthur.

 

[Faradave]

Get it right.

The Hubble Bubble (HB) derives from its structure, something we want, speed limit c. It also removes something we don’t really want, i (i.e. √(-1) ). That’s because HB affords us the right right triangle from which to calculate the light-like interval.

Although the geometry of space-time is not Euclidean in the ordinary sense, there *is* a geometry which is very similar, but peculiar in certain respects. ...[interval] We give it a different name [than length] because it is in a different geometry, but the interesting thing is only that some signs are reversed and there is a c in it.

If we look again on our region of interest in HB (see post #13 image), focusing on V₀ (at rest) and V_max (speed c), a right triangle may be constructed (below) from the point (A) where both trajectories depart arc t₁ and the two locations (B & C) where they arrive on arc t₂.

Note that the hypotenuse, so formed, is a chord of spatial arc t₂ which, owing to local flatness, may be considered equal in length to that arc. From Pythagoras we find: chord² = time² + interval², where the chord represents a spatial component. Rearranging, we get the familiar relation: interval² = space² - time², but without invoking √(-1) in the manifold.

*(p. 97) His lecture “Space-Time” is published in many forms.

 

[Jorrie]

The Hubble Bubble (HB) derives from its structure, something we want, speed limit c. It also removes something we don’t really want, i (i.e. √(-1) ). That’s because HB affords us the right right triangle from which to calculate the light-like interval.

Dave, I spot three immediate problems with your "get it right".

1. The "Hubble Bubble" term is an existing concept in astronomy and it is not the same as what you are portraying here. So I suggest you choose another name for your representation. In fact to call it anything "Hubble-like" may just create confusion, because we already have many such concepts with well defined meanings, e.g. 'Hubble sphere' or 'Hubble volume'.

2. The spacetime diagram that you depict seems similar (perhaps identical) to the Epstein space-propertime diagram. It has a certain appeal to the the beginner, but it also has a number of drawbacks, e.g. it is a curious and to some extent even invalid mix of "my space' vs. "your time", or vice versa. There are a few more drawback, which I will not go into here. I think there have been some threads about it in the past, e.g. this one.

3. Although it is valid to use a modified balloon analogy with time as the radial parameter, you may be confusing readers by then apparently moving off the surface (the radius of which is growing at the speed of light) in order to draw a form of spacetime diagram. You essentially have to first 'freeze' the balloon's expansion before you can do this. All very confusing, IMO.

I will leave it to the mods to decide if this is a useful line of discussion for this forum

 

[Faradave]

“3.5D” That’s for me.

"Hubble Bubble" term is an existing concept in astronomy… I suggest you choose another name…

Mea culpa! (Sorry, Edwin.) I should have searched the term first. I’m unable to expunge my prior HB references. Too bad no one said anything after my post #12 (Hubble Bubble (HB)?), but there may not be enough readers. Henceforth, I’ll refer generically to a “radial time manifold” and “3.5D” (see https://www.physicsforums.com/showthread.php?t=641966) specific to my adaptation of our so-called 4D.

The spacetime…you depict seems similar (perhaps identical) to the Epstein space-propertime diagram…thread about it

Thanks for the links. With local flatness, all SR models should be consistent. The concept of constant 4-velocity is not new to me. I find it appealing. But the exercise they go through merely illustrates SR, it does not comment on the structure of the manifold, expansion or derive speed c from it. 3.5D does.

Although it is valid to use a modified balloon analogy with time as the radial parameter you may be confusing readers by then apparently moving off the surface (the radius of which is growing at the speed of light) in order to draw a form of spacetime diagram. You essentially have to first 'freeze' the balloon's expansion before you can do this.

It doesn’t have to be confusing to anyone acquainted with a Minkowski diagram (M2).

As you know, by convention, a single spatial dimension is represented by the x-axis with time on the vertical axis. Light intervals are 45°. The origin is (here,now).

The x-axis represents space at time = now. You could draw a horizontal line one unit above it to represent space at time = now + 1. And another a unit above that, etc. It becomes clear that M2 represents a vertical sequence of lines, stacked like a deck of cards. Each card is space “frozen” at a moment in time. And the light interval cuts right through them to arrive at its absorption event in the future.

I adapt this by simply bending the deck of cards downward until the light interval is horizontal. That makes time radial from its own origin, the Big Bang (time = 0). Keeping things nice and neat, the x-axis (and every card in the deck) becomes circular. That’s why I use a cross-section of an onion to illustrate it.

I like Minkowski diagrams, but there are reasons for other versions. Light appears to age as it rises at 45° with respect to the time axis, yet the Lorentz transforms indicate it can’t. M2 doesn’t accommodate expansion of space. The concentric spatial (onion) rings of 3.5D do. Speed c is applied to M2 as a conversion factor, but c derives from 3.5D.

What might be confusing is that the image of an expanding balloon suggests that particles stay within a single expanding layer of space (the one balloon surface). But in spacetime, every moment has its own, new layer of space making a ball rather than a sphere. But whether it’s a flat stack of space layers or a ball of nested layers, particles in spacetime exist as worldlines. Particles don’t travel along the worldlines, they ARE their worldlines.

The film analogy is often used here. Actors images don’t leave the previous frame to go into the next one. The actor exists in every frame of his scene, in every space of his spacetime.

 

[Garth]

A structure I believe reveals this is the Hubble Bubble (HB, closely related to the balloon analogy see post #12 above and https://www.physicsforums.com/showthread.php?t=641966). Space is imagined as an expanding 3-sphere having radial time. A region of interest (see images) is depicted in cross section, as a curved-space “Hubble diagram”, analogous to the Minkowski diagram of flat spacetime. Here, two arcs represent a spatial dimension at times t₁ and t₂, separated by Δt. A particle at rest moves upward, along its radial timeline. Moving particles make an angle to this, which increases with velocity. Each higher velocity experiences a smaller temporal component.

Hi Faradave, have you seen the Relationship between expanding universe and time thread? In particular my post!

Garth

 

[Jorrie]

I like Minkowski diagrams, but there are reasons for other versions. Light appears to age as it rises at 45° with respect to the time axis, yet the Lorentz transforms indicate it can’t. M2 doesn’t accommodate expansion of space. The concentric spatial (onion) rings of 3.5D do. Speed c is applied to M2 as a conversion factor, but c derives from 3.5D.

I'm glad you have changed your terminology away from Hubble terms and also from the balloon analogy, but please also guard against false statements like: "Light appears to age as it rises at 45° with respect to the time axis, yet the Lorentz transforms indicate it can’t."

There are very good reasons why Minkowski spacetime diagrams look as they do and they are fully compatible with the Lorentz transforms. If you think otherwise, I think that you misunderstand what they mean or how they work.

In a way, I feel that threads like yours may be confusing naïve readers, but then, perhaps it serves a good purpose if advisers here can discuss misrepresentations for the benefit of those readers.

My final comment on your 3.5D:

What might be confusing is that the image of an expanding balloon suggests that particles stay within a single expanding layer of space (the one balloon surface). But in spacetime, every moment has its own, new layer of space making a ball rather than a sphere. But whether it’s a flat stack of space layers or a ball of nested layers, particles in spacetime exist as worldlines. Particles don’t travel along the worldlines, they ARE their worldlines.

Yes, but then you essentially have a timeless representation, e.g. light only moves horizontally on a spatial diagram if time is absent; or in other words, the diagram is plotted for the proper time of each particle and each particle has a different rate of proper time. Curios mix of coordinates.

One more thing (says inspector Columbo :), where are spacelike intervals on your representation?