- #1
Stupidboyboy
- 5
- 0
Topology of open set(newbie, I am stuck help!)
Hi just found this found and have some basic questions about topology.
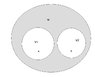
If let say exist a metrix space (M,s) and two points [tex]x \neq y[/tex] in M. Then show that there exists open sets [tex]V_1,V_2 \in \mathcal{T}_s[/tex] such that [tex]x \in V_1[/tex] and [tex]x \in V_2[/tex] and finally [tex]V_1 \cap V_2 = \emptyset[/tex]
I try to solve question 1)
For V_1 to be an open set set then there must exist an open ball such that B(x)_r < r where is the radius of the ball which is M. Have I understood this correctly?
Homework Statement
Hi just found this found and have some basic questions about topology.
If let say exist a metrix space (M,s) and two points [tex]x \neq y[/tex] in M. Then show that there exists open sets [tex]V_1,V_2 \in \mathcal{T}_s[/tex] such that [tex]x \in V_1[/tex] and [tex]x \in V_2[/tex] and finally [tex]V_1 \cap V_2 = \emptyset[/tex]
Homework Equations
The Attempt at a Solution
I try to solve question 1)
For V_1 to be an open set set then there must exist an open ball such that B(x)_r < r where is the radius of the ball which is M. Have I understood this correctly?
Attachments
Last edited: