- #1
nahya
- 27
- 0
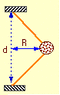
The rock is held by two of the same 40 cm strings with ends 70 cm apart and whirled in a circle between them. Neglect gravity.
--
i found the radius of the circular motion to be 19.36cm = 0.1936m.
now the question is "Now what is the maximum speed the rock can have before the string breaks?"
the hint says, "Find the angle between direction of acceleration and the string tensions. Use this to break the tensions into their components."
well... the direction of acceleration is towards the center...
i can get the the net x-force, which is x = 35cos(theta). i know the acceleration is towards the direction of x, but the magnitude is unknown, because the velocity is unknown, right?
or...
i thought of it this way.
both the strings can endure 35N of tension. they are in the opposite direction, so not 70N.
a = F/m = 35/0.57 =~61.40
v = sqrt(ar) =~ sqrt(61.40 * 0.1936) =~ 3.45.
that's incorrect, apparently.
i thought maybe the radius was the original radius, which is 56.40 (from sqrt(40^2 - 35^2)), but that is also incorrect...
i think the second way of thinking is correct, but maybe I'm not finding the right numbers to plug in.
--
i found the radius of the circular motion to be 19.36cm = 0.1936m.
now the question is "Now what is the maximum speed the rock can have before the string breaks?"
the hint says, "Find the angle between direction of acceleration and the string tensions. Use this to break the tensions into their components."
well... the direction of acceleration is towards the center...
i can get the the net x-force, which is x = 35cos(theta). i know the acceleration is towards the direction of x, but the magnitude is unknown, because the velocity is unknown, right?
or...
i thought of it this way.
both the strings can endure 35N of tension. they are in the opposite direction, so not 70N.
a = F/m = 35/0.57 =~61.40
v = sqrt(ar) =~ sqrt(61.40 * 0.1936) =~ 3.45.
that's incorrect, apparently.
i thought maybe the radius was the original radius, which is 56.40 (from sqrt(40^2 - 35^2)), but that is also incorrect...
i think the second way of thinking is correct, but maybe I'm not finding the right numbers to plug in.
Attachments
Last edited: