Is this condition for infinite roots wrong?

[vopros217]

I found a strange theorem and a doubtful method in Stroud's book "Engineering mathematics":

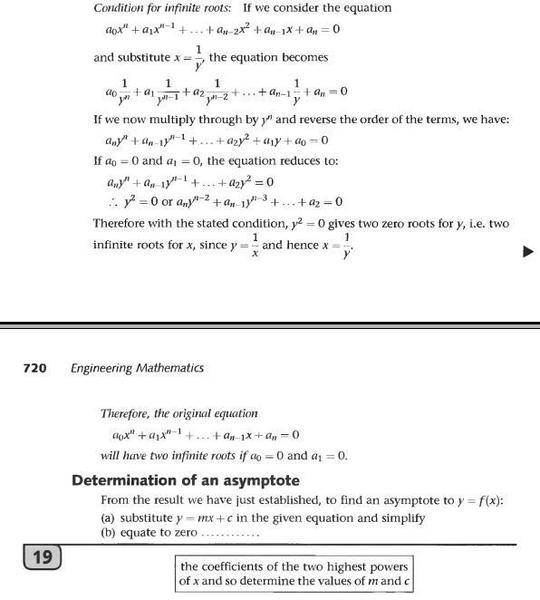
I think, every polynomial equation will have two infinite roots (at +infinity and -infinity).
I also think that this method of the determination of an asymptote gives wrong results if f(x) is a polynomial with a high degree.
Are the theorem and the method in this book wrong?

 

[mfb]

That doesn't make sense. Every polynomial has a largest power, so every polynomial has an infinite number of infinite roots?
Multiplying the equation by yⁿ generates additional solutions for y=0, so it is not surprising that they produce unmathematical solutions for y=0 later. Of course y=0 is a solution to an equation where you multiplied both sides by 0.

 

[micromass]

This looks strange but it is kind of correct. It seems the author absolutely butchered the method though. I think what the author likes to do is to compute infinite point and asymptotes in projective geometry using homogeneous coordinates. That uses a method kind of similar to what is in the OP, but the exact explanation of the book is very doubtful.

Something of the following would be correct though: consider $y = \frac{x+2}{3x + 2}$. Then we have
$$3xy + 2y - x - 2 = 0$$
which gives rise to a homogeneous equation
$$3xy + 2yz - xz - 2z^2=0.$$
The points at infinity correspond to $z=0$, which yields $x=0$ or $y=0$. This gives us a result that the function has two asymptotes: one parallel to $x=0$ and one parallel to $y=0$ which is indeed correct.

 

[mathman]

Statement is strange. if $a_0\ and\ a_1$ are both 0, then the first term should be $a_2x^{n-2}$.

 

[vopros217]

Thank you all. Stroud's textbook is mostly very good, but I stuck on that place.