- #1
Math Amateur
Gold Member
MHB
- 3,990
- 48
I am reading Kenneth Ireland and Michael Rosen's book, "A Classical Introduction to Modern Number Theory" ... ...
I am currently focused on Chapter 12: Algebraic Number Theory ... ...
I need some help in order to follow a basic result in Section 1: Algebraic Preliminaries ...
The start of Section 1 reads as follows:
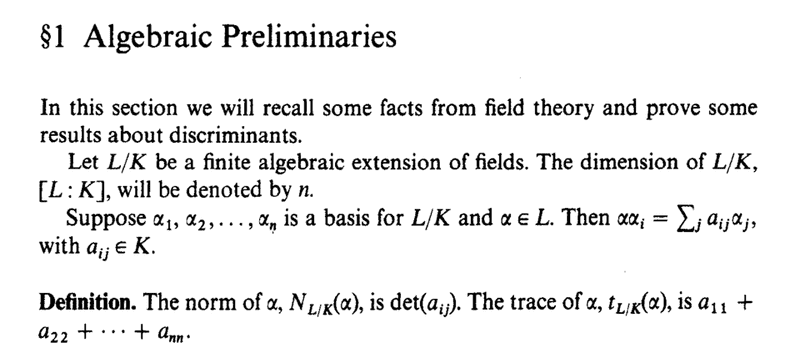
QUESTION 1In the above text by Ireland and Rosen, we read the following:"... ... Suppose ##\alpha_1, \alpha_2, \ ... \ ... \ , \alpha_n## is a basis for ##L/K## and ##\alpha \in L##.
Then ##\alpha \alpha_i = \sum_j a_{ ij } \alpha_j## with ##a_{ ij } \in K## ... ... ""My question is ... ... how do Ireland and Rosen get ##\alpha \alpha_i = \sum_j a_{ ij } \alpha_j ## ... ... ?
My thoughts are as follows ...Given ##L/K##, we have that ##L## is a vector space over ##K##.
... we then let ##\alpha_1, \alpha_2, \ ... \ ... \ , \alpha_n## be a basis for ##L## as a vector space over ##K##
( i take it that that is what I&R mean by "... ... Suppose ##\alpha_1, \alpha_2, \ ... \ ... \ , \alpha_n## is a basis for ##L/K##")... we then let ##\alpha \in L## ... ... then there exist ##a_1, a_2, \ ... \ ... \ , a_n \in K##such that##\alpha = a_1 \alpha_1 + a_2 \alpha_2 + \ ... \ ... \ a_n \alpha_n##so that##\alpha \alpha_i = ( a_1 \alpha_1 + a_2 \alpha_2 + \ ... \ ... \ a_n \alpha_n ) \alpha_i ## ... ... ... (1)... BUT ...
Ireland and Rosen write (see above)##\alpha \alpha_i = \sum_j a_{ ij } \alpha_j####= a_{ i1 } \alpha_1 + a_{ i2 } \alpha_2 + \ ... \ ... \ + a_{ in } \alpha_n## ... ... ... (2)My question is ... how do we get expression (1) equal to (2) ... ...
QUESTION 2In the above text by Ireland and Rosen, we read the following:"... ...The norm of ##\alpha, N_{ L/K } ( \alpha )## is ##\text{ det} (a_{ ij }) ## ... ...I cannot fully understand the process involved in forming the norm ... can someone please explain ... preferably via a simple example ...
Hope someone can help ...Peter
I am currently focused on Chapter 12: Algebraic Number Theory ... ...
I need some help in order to follow a basic result in Section 1: Algebraic Preliminaries ...
The start of Section 1 reads as follows:
QUESTION 1In the above text by Ireland and Rosen, we read the following:"... ... Suppose ##\alpha_1, \alpha_2, \ ... \ ... \ , \alpha_n## is a basis for ##L/K## and ##\alpha \in L##.
Then ##\alpha \alpha_i = \sum_j a_{ ij } \alpha_j## with ##a_{ ij } \in K## ... ... ""My question is ... ... how do Ireland and Rosen get ##\alpha \alpha_i = \sum_j a_{ ij } \alpha_j ## ... ... ?
My thoughts are as follows ...Given ##L/K##, we have that ##L## is a vector space over ##K##.
... we then let ##\alpha_1, \alpha_2, \ ... \ ... \ , \alpha_n## be a basis for ##L## as a vector space over ##K##
( i take it that that is what I&R mean by "... ... Suppose ##\alpha_1, \alpha_2, \ ... \ ... \ , \alpha_n## is a basis for ##L/K##")... we then let ##\alpha \in L## ... ... then there exist ##a_1, a_2, \ ... \ ... \ , a_n \in K##such that##\alpha = a_1 \alpha_1 + a_2 \alpha_2 + \ ... \ ... \ a_n \alpha_n##so that##\alpha \alpha_i = ( a_1 \alpha_1 + a_2 \alpha_2 + \ ... \ ... \ a_n \alpha_n ) \alpha_i ## ... ... ... (1)... BUT ...
Ireland and Rosen write (see above)##\alpha \alpha_i = \sum_j a_{ ij } \alpha_j####= a_{ i1 } \alpha_1 + a_{ i2 } \alpha_2 + \ ... \ ... \ + a_{ in } \alpha_n## ... ... ... (2)My question is ... how do we get expression (1) equal to (2) ... ...
QUESTION 2In the above text by Ireland and Rosen, we read the following:"... ...The norm of ##\alpha, N_{ L/K } ( \alpha )## is ##\text{ det} (a_{ ij }) ## ... ...I cannot fully understand the process involved in forming the norm ... can someone please explain ... preferably via a simple example ...
Hope someone can help ...Peter
Attachments
Last edited: