For which values are the variables independent?

[mathmari]

Hey! :giggle:

We have the table of distribution of $X$, $Y$ and their joint random variable :

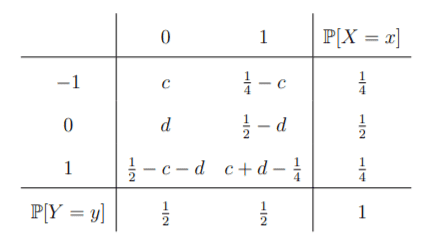
with $$(c,d)\in \left \{(c,d)\in \mathbb{R}^2\mid 0\leq c\leq \frac{1}{4}, \ 0\leq d\leq \frac{1}{2}, \ \frac{1}{4}\leq c+d\leq \frac{1}{2}\right \}$$

I want to calculate the values of $c$ and $d$ such that $X$ and $Y$ are independent.
So do we have to check each combination so that $P[X=m,\ Y=n]=P[X=m]\cdot P[Y=n]$ ?

\begin{align*}&P[X=-1, \ Y=0]=c \ \text{ and } \ P[X=-1]\cdot P[Y=0]=\frac{1}{4}\cdot \frac{1}{2}=\frac{1}{8} \text{ so } c=\frac{1}{8} \\ &P[X=0, \ Y=0]=d \ \text{ and } \ P[X=0]\cdot P[Y=0]=\frac{1}{2}\cdot \frac{1}{2}=\frac{1}{4} \text{ so } d=\frac{1}{4}\end{align*}
We check now also the others if $X$ and $Y$ are indeed independent (or do we not have to? :unsure: )
\begin{align*}&P[X=-1, \ Y=1]=\frac{1}{4}-c=\frac{1}{4}-\frac{1}{8}=\frac{1}{8} \ \text{ and } \ P[X=-1]\cdot P[Y=1]=\frac{1}{4}\cdot \frac{1}{2}=\frac{1}{8} \text{ so correct} \\ &P[X=0, \ Y=0]=d=\frac{1}{4} \ \text{ and } \ P[X=0]\cdot P[Y=0]=\frac{1}{2}\cdot \frac{1}{2}=\frac{1}{4} \text{ so correct} \\ &P[X=0, \ Y=1]=\frac{1}{2}-d=\frac{1}{2}-\frac{1}{4}=\frac{1}{4} \ \text{ and } \ P[X=0]\cdot P[Y=1]=\frac{1}{2}\cdot \frac{1}{2}=\frac{1}{4} \text{ so correct}\\ &P[X=1, \ Y=0]=\frac{1}{2}-c-d=\frac{1}{2}-\frac{1}{8}-\frac{1}{4}=\frac{1}{8} \ \text{ and } \ P[X=1]\cdot P[Y=0]=\frac{1}{4}\cdot \frac{1}{2}=\frac{1}{8} \text{ so correct}\\ &P[X=1, \ Y=1]=c+d-\frac{1}{4}=\frac{1}{8}+\frac{1}{4}-\frac{1}{4}=\frac{1}{8} \ \text{ and } \ P[X=1]\cdot P[Y=1]=\frac{1}{4}\cdot \frac{1}{2}=\frac{1}{8} \text{ so correct} \end{align*}:unsure:

 

[I like Serena]

Hey mathmari!

Seems correct to me. (Nod)

We might skip a couple of them, since we know that if $A$ and $B$ are independent, that $A$ and $B^c$ are also independent.

 

[mathmari]

We might skip a couple of them, since we know that if $A$ and $B$ are independent, that $A$ and $B^c$ are also independent.

You mean to check only with $Y=0$ and not with $Y=1$ ? :unsure:

 

[I like Serena]

You mean to check only with $Y=0$ and not with $Y=1$ ?

Indeed. (Nod)

 

[I like Serena]

Similarly we can skip $X=1$ after checking $X=-1$ and $X=0$.
We can prove it if we want to.