R-Linear and C-Linear Mappings.... Another Question ....

[Math Amateur]

I am reading Reinhold Remmert's book "Theory of Complex Functions" ...

I am focused on Chapter 0: Complex Numbers and Continuous Functions ... and in particular on Section 1.2:$\mathbb{R}$-linear and $\mathbb{C}$]-linear mappings of $\mathbb{C}$ into $\mathbb{C}$ ... ...

I need help in order to fully understand one of Remmert's results regarding $\mathbb{C}$-linear mappings of $\mathbb{C}$ into $\mathbb{C}$ ... ...

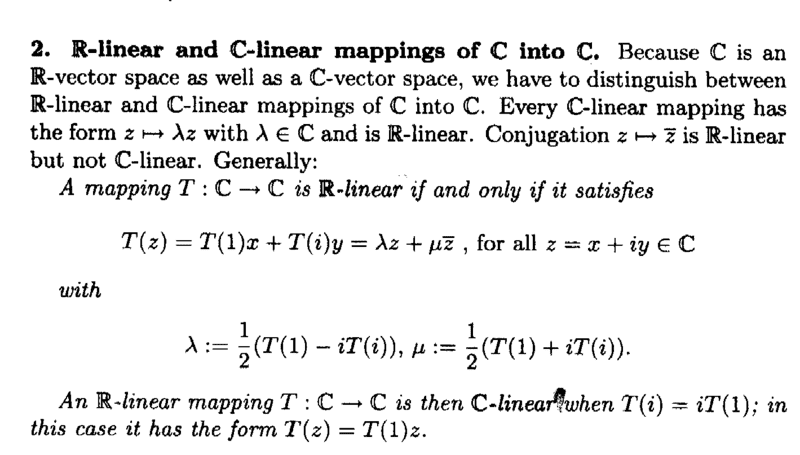
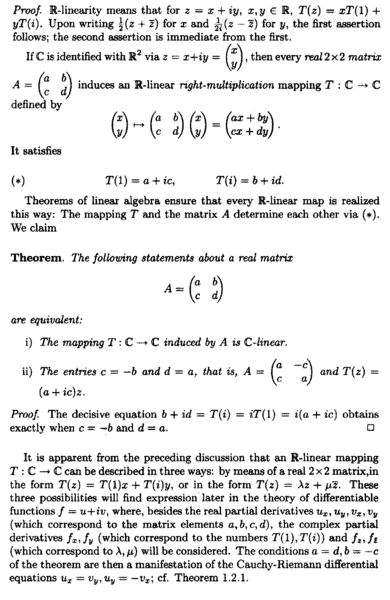
Remmert's section on $\mathbb{R}$-linear and $\mathbb{C}$-linear mappings of $\mathbb{C}$ into $\mathbb{C}$ reads as follows:

In the above text by Remmert we read the following: (... fairly near the start of the text ...)

" ... ... An $\mathbb{R}$-linear mapping $T : \mathbb{C} \to \mathbb{C}$ is then $\mathbb{C}$-linear when $T(i) = i T(1)$; in this case it has the form $T(z) = T(1) z$. ... ... "My questions are as follows:Question 1

How/why exactly is an $\mathbb{R}$-linear mapping $T : \mathbb{C} \to \mathbb{C}$ also $\mathbb{C}$-linear when $T(i) = i T(1)$ ... ... ?
Question 2

Why/how exactly does a $\mathbb{C}$-linear mapping have the form $T(z) = T(1) z$ ... ...
Hope someone can help ...

Peter

 

[fresh_42]

Hi Peter!

What does linearity mean? Can you write down the two conditions for a function $T$ to be, say $\mathbb{F}-$linear?

If so, can you apply it in the case $\mathbb{F}=\mathbb{C}$ on $T(z\cdot 1)\,?$
This should answer your second question.
Next apply the case $\mathbb{F}=\mathbb{R}$ on $T(a\cdot 1 + b \cdot i)\,.$
This should answer your first question.

 

[Math Amateur]

The condition for a function $T : \mathbb{C} \to \mathbb{C}$ to be $\mathbb{F}$]-linear are as follows:

Additivity

$T(w + z) = T(w) + T(z)$ for all $w,z \in \mathbb{C}$ ... ... ... (1)

Homogeneity

$T( \lambda w ) = \lambda T(w)$ for all $\lambda \in \mathbb{F}$ and $w \in \mathbb{C}$ ... ... ... (2)Now apply the above to $\mathbb{F} = \mathbb{C}$ on $T( z \cdot 1 )$

Then ... we have ... $T( z \cdot 1 ) = z T(1)$

But ... $T( z \cdot 1 ) = T(z)$ ...

So ... $T(z) = z T(1) = T(1) z$ ... so, question 2 above is answered ... ...Now try to get answer to question 1 ...

Let $z = a + b i$ ... and $\mathbb{F} = \mathbb{R}$ ...

Then $T(z) = T(a \cdot 1) = a T(1) + b T(i)$ ... ...

BUT ... where to from here ...?Can you help further ...

Peter

 

[fresh_42]

The condition for a function $T : \mathbb{C} \to \mathbb{C}$ to be $\mathbb{F}$]-linear are as follows:

Additivity

$T(w + z) = T(w) + T(z)$ for all $w,z \in \mathbb{C}$ ... ... ... (1)

Homogeneity

$T( \lambda w ) = \lambda T(w)$ for all $\lambda \in \mathbb{F}$ and $w \in \mathbb{C}$ ... ... ... (2)

Now try to get answer to question 1 ...

Let $z = a + b i$ ... and $\mathbb{F} = \mathbb{R}$ ...

Then $T(z) = T(a \cdot 1) = a T(1) + b T(i)$ ... ...

Firstly, here is a typo: $T(z) = T(z \cdot 1)$ but this isn't of interest in the first step.

We have for $z=a+ib \in \mathbb{C}$ that $a,b\in \mathbb{R}$. Now $T$ is $\mathbb{R}-$linear, so we apply first additivity and get $T(z)=T(a+ib)=T(a)+T(ib)$. This might be confusing, as one of the summands isn't real. However, we have the $\mathbb{R}-$linearity on the vector space $\mathbb{R}^2\cong \mathbb{C}$, that is $T((a,b))=T((a,0))+T((0,b))$ and the difference between $(a,b)$ and $a+ib$ is merely a notation one.

Next we apply homogeneity which is allowed, because $a,b \in \mathbb{R}$. Thus we get $T(z)=\ldots = T(a \cdot 1)+ T(b \cdot i)=a\cdot T(1) + b\cdot T(i)$. Here we are stuck, but we have an additional condition from question 1

Question 1

How/why exactly is an $\mathbb{R}$-linear mapping $T : \mathbb{C} \to \mathbb{C}$ also $\mathbb{C}$-linear when $T(i) = i T(1)$ ... ... ?

With that, we have $T(z)=\ldots =a\cdot T(1) + b\cdot T(i) = a \cdot T(1) + b\cdot i\cdot T(1)= (a+bi)\cdot T(1)= z\cdot T(1)$ which is $\mathbb{C}-$homogeneity. From this we also get $T(z_1+z_2)=T(z_1)+T(z_2)$ since the complex addition goes componentwise: the $a_j$ add and the $b_j$ do, and we can proceed in the same way.

Both together is $\mathbb{C}-$linearity.

And from the answer to question 1 we see, that it also holds in the opposite direction: If $T$ is $\mathbb{C}-$linear, then $T(i)=i \cdot T(1)$, simply use $z=i\,.$

 

[Math Amateur]

Thanks fresh_42 ...

I appreciate your help ...

Peter