A problem about non-separable Hilbert space

[prophetlmn]

also see
http://planetmath.org/exampleofnonseparablehilbertspace

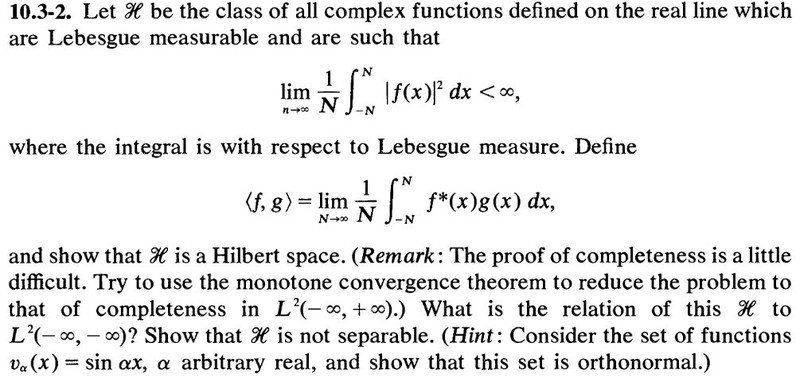
the main difficulty is about the completeness, which is hard to prove, the author's hint seems don't work here, for you can not use the monotone convergence theorem directly , f(x)χ[-N,N]/sqrt[N] is not monotone

 

[micromass]

Let your pre-Hilbert space be $H$ with norm $\|~\|$. It is known that a space is is complete iff every absolute convergent series is convergent.
Thus let $(f_n)_n$ be a sequence in $H$ such that $\sum_n \|f_n\|$ converges (to a number $A$). Thus by monotone convergence:

$$\lim_{N\rightarrow +\infty} \frac{1}{\sqrt{N}}\sum_n \|f_n \chi_{[-N,N]}\|_2 = A$$

Thus for each $N$, we have that the series $\sum_n \|f_n\chi_{[-N,N]}\|_2$ converges. Since $L^2$ is complete, we have that $\sum_n f_n\chi_{[-N,N]} = g_N$ for some $g_N\in L^2$.

Now, if $M>N$, then $g_N\chi_{[-N,N]} = g_M\chi_{[-N,N]}$. Thus we can glue the $g_N$ to a big function $g$. Now

\begin{eqnarray*}
\|g - \sum_{n=1}^m f_n\|
& = & \lim_{N\rightarrow +\infty} \frac{1}{\sqrt{N}}\left\| g\chi_{[-N,N}] - \sum_{n=1}^m f_n\chi_{[-N,N]}\right\|_2\\
& = & \lim_{N\rightarrow +\infty} \frac{1}{\sqrt{N}}\left\| \sum_{n=m}^{+\infty} f_n\chi_{[-N,N]}\right\|_2\\
&\leq & \limsup_{N\rightarrow +\infty} \frac{1}{\sqrt{N}} \sum_{n=m}^{+\infty} \|f_n\chi_{[-N,N]}\|_2 \\
&\leq & \sum_{n=m}^{+\infty} \|f_n\|\\
& \rightarrow & 0
\end{eqnarray*}[/tex]

 

[prophetlmn]

Let your pre-Hilbert space be $H$ with norm $\|~\|$. It is known that a space is is complete iff every absolute convergent series is convergent.
Thus let $(f_n)_n$ be a sequence in $H$ such that $\sum_n \|f_n\|$ converges (to a number $A$). Thus by monotone convergence:

$$\lim_{N\rightarrow +\infty} \frac{1}{\sqrt{N}}\sum_n \|f_n \chi_{[-N,N]}\|_2 = A$$

Thus for each $N$, we have that the series $\sum_n \|f_n\chi_{[-N,N]}\|_2$ converges. Since $L^2$ is complete, we have that $\sum_n f_n\chi_{[-N,N]} = g_N$ for some $g_N\in L^2$.

Now, if $M>N$, then $g_N\chi_{[-N,N]} = g_M\chi_{[-N,N]}$. Thus we can glue the $g_N$ to a big function $g$. Now

\begin{eqnarray*}
\|g - \sum_{n=1}^m f_n\|
& = & \lim_{N\rightarrow +\infty} \frac{1}{\sqrt{N}}\left\| g\chi_{[-N,N}] - \sum_{n=1}^m f_n\chi_{[-N,N]}\right\|_2\\
& = & \lim_{N\rightarrow +\infty} \frac{1}{\sqrt{N}}\left\| \sum_{n=m}^{+\infty} f_n\chi_{[-N,N]}\right\|_2\\
&\leq & \limsup_{N\rightarrow +\infty} \frac{1}{\sqrt{N}} \sum_{n=m}^{+\infty} \|f_n\chi_{[-N,N]}\|_2 \\
&\leq & \sum_{n=m}^{+\infty} \|f_n\|\\
& \rightarrow & 0
\end{eqnarray*}[/tex]

thanks for your help,the proof is very clear,the key point is 'a space is is complete iff every absolute convergent series is convergent',I don't know this before, it's again the old truth 'take a different approach'

 

[prophetlmn]

I still have two little problems
(1)what do you mean by 'Thus by monotone convergence',I mean don't you just use the definition of
$\sum_n \|f_n\|$

(2)why you use limsub in the last three steps
\begin{eqnarray*}
\lim_{N\rightarrow +\infty} \frac{1}{\sqrt{N}}\left\| \sum_{n=m}^{+\infty} f_n\chi_{[-N,N]}\right\|_2\\
&\leq & \limsup_{N\rightarrow +\infty} \frac{1}{\sqrt{N}} \sum_{n=m}^{+\infty} \|f_n\chi_{[-N,N]}\|_2 \\
&\leq & \sum_{n=m}^{+\infty} \|f_n\|\\
\end{eqnarray*}

what's wrong with
\begin{eqnarray*}
\lim_{N\rightarrow +\infty} \frac{1}{\sqrt{N}}\left\| \sum_{n=m}^{+\infty} f_n\chi_{[-N,N]}\right\|_2\\
&\leq & \lim_{N\rightarrow +\infty} \frac{1}{\sqrt{N}} \sum_{n=m}^{+\infty} \|f_n\chi_{[-N,N]}\|_2 \\
&= & \sum_{n=m}^{+\infty} \|f_n\|\\
\end{eqnarray*}

 

[micromass]

I still have two little problems
(1)what do you mean by 'Thus by monotone convergence',I mean don't you just use the definition of
$\sum_n \|f_n\|$

You exchange a series and a limit. This is not always allowed.

(2)why you use limsub in the last three steps
\begin{eqnarray*}
\lim_{N\rightarrow +\infty} \frac{1}{\sqrt{N}}\left\| \sum_{n=m}^{+\infty} f_n\chi_{[-N,N]}\right\|_2\\
&\leq & \limsup_{N\rightarrow +\infty} \frac{1}{\sqrt{N}} \sum_{n=m}^{+\infty} \|f_n\chi_{[-N,N]}\|_2 \\
&\leq & \sum_{n=m}^{+\infty} \|f_n\|\\
\end{eqnarray*}

Because if $x_n\leq y_n$, then this does not imply $\lim_n x_n\leq \lim_n y_n$. This is only true if the limits exist. To solve this, we use limsup.

 

[prophetlmn]

You exchange a series and a limit. This is not always allowed.



Because if $x_n\leq y_n$, then this does not imply $\lim_n x_n\leq \lim_n y_n$. This is only true if the limits exist. To solve this, we use limsup.

so you mean here we should use something like Fatou's lemma?

 

[micromass]

so you mean here we should use something like Fatou's lemma?

Yes.

 

[prophetlmn]

Yes.

So we have
\begin{eqnarray*}
\lim_{N\rightarrow +\infty} \frac{1}{\sqrt{N}}\sum_n \|f_n \chi_{[-N,N]}\|_2\\
&\leq & \sum_n\lim_{N\rightarrow +\infty} \frac{1}{\sqrt{N}} \|f_n \chi_{[-N,N]}\|_2\\
\end{eqnarray*}
?
if so how to prove it?i.e. how you get the following

\begin{eqnarray*}
\limsup_{N\rightarrow +\infty} \frac{1}{\sqrt{N}} \sum_{n=m}^{+\infty} \|f_n\chi_{[-N,N]}\|_2 \\
&\leq & \sum_{n=m}^{+\infty} \|f_n\|\\
\end{eqnarray*}

and I think when you say'You exchange a series and a limit. This is not always allowed' you mean an exchange like this kind?i.e how you get
$$\lim_{N\rightarrow +\infty} \frac{1}{\sqrt{N}}\sum_n \|f_n \chi_{[-N,N]}\|_2 = A$$