- #1
JohanL
- 158
- 0
Homework Statement
3. The Attempt at a Solution [/B]
*****************************************
Can anyone possibly explain step 3 and 4 in this solution?
JohanL said:Homework Statement
3. The Attempt at a Solution [/B]
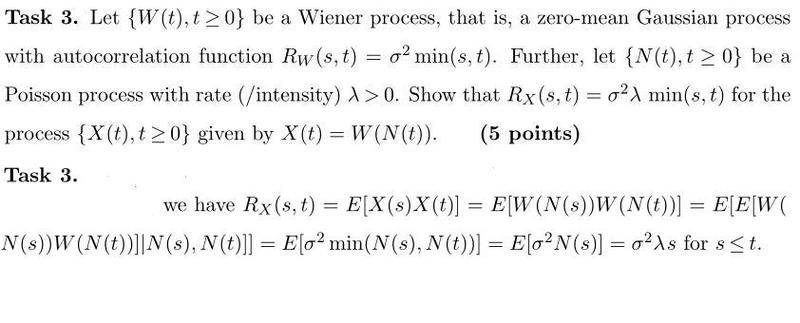
*****************************************
Can anyone possibly explain step 3 and 4 in this solution?
Ray Vickson said:Sometimes (at least when one is starting out) it is better to be less abstract and more explicit. So, let's be explicit.
Assume ##s < t##, so ##N(t) \geq N(s)## (because of the possible arrivals between ##s## and ##t##). Thus
[tex] \begin{array}{rcl}R_X(s,t) &= & \sum_{j=0}^{\infty} \sum_{k=0}^{\infty} P(N(s)=j, N(t) = j+k) E[W(j) W(j+k)] \\
&= &\sigma^2 \sum_j \sum_k P(N(s)=j) P(N(t) = j+k|N(s)=j) \min(j,j+k)
\end{array}
[/tex]
Of course, ##\min(j,j+k) = j## and we also have ##P(N(t) = j+k | N(s) = j) = P(N(t-s) = k)##, by stationarity and independent increments of the Poisson process. Now the rest is easy.
Of course, if ##t < s## we can just interchange the roles of ##s## and ##t## in the argument.
andrewkirk said:Step 3 looks like an application of the Tower Law or 'Law of Total Expectation' (see link). It's a very useful law and well worth spending the time to familiarise yourself with it!
The fourth step is just an application of the given autocorrelation function to the expression inside the outer expectation.
An autocorrelation function is a mathematical tool used in statistics to measure the correlation between a time series and a lagged version of itself. It is often used to identify patterns or relationships between data points over time.
The autocorrelation function for a Wiener process is calculated by taking the covariance between two points in time and dividing it by the product of their individual variances. This process is repeated for different time lags to create a series of autocorrelation values.
A Wiener process, also known as Brownian motion, is a mathematical model used to describe the random movement of particles. It is often used in finance, physics, and other fields to model the behavior of systems that undergo random fluctuations.
The autocorrelation function for a Poisson process is calculated by taking the covariance between two time intervals and dividing it by the product of their individual means. This process is repeated for different time lags to create a series of autocorrelation values.
A Poisson process is a mathematical model used to describe the occurrence of events over time. It is often used in fields such as queuing theory and reliability analysis to model the arrival of customers or failures of a system.