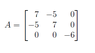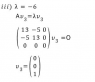Can anyone explain to me why eigenvector here is like this

[sozener1]

Im supposed to find the eigenvectors and eigenvalues of A

I found that eigenvalues are 2 12 and -6

then I found eigen vectors substituting -6 to lambda

and someone has told me I get 0 0 1 for eigenvector which I cannot understand why??

can anyone pleasezzzzzzzz explain why this is?

 

[mfb]

Did you multiply this vector with your matrix? What do you get?
Does this fit to the definition of an eigenvector?

 

[sozener1]

Did you multiply this vector with your matrix? What do you get?
Does this fit to the definition of an eigenvector?

I get 0 0 0

it doesn't fit with the definition of eigenvectors

so does that mean it should be 0 0 0 instead of 0 0 1??

I mean when you try to calculate for v3 = [ v1 v2 v3]


since the last row of the matrix is 0 0 0 should v3 come out as 0?? couldn't v3 be any number??

 

[Mark44]

I get 0 0 0

That's not what I get.

The matrix you showed in the second attachment is not A. It is [A - (-6)I]. Of course if you multiply this matrix times your eigenvector, you'll get the zero vector.

it doesn't fit with the definition of eigenvectors

so does that mean it should be 0 0 0 instead of 0 0 1??

I mean when you try to calculate for v3 = [ v1 v2 v3]


since the last row of the matrix is 0 0 0 should v3 come out as 0?? couldn't v3 be any number??

Assuming that your eigenvalue is λ and that x is an as-yet unknown eigenvector for λ, what you're doing is solving the equation Ax = λx for x. That's equivalent to solving the equation (A - λI)x = 0. In other words, of finding the kernel of the matrix A - λI. This should be something that you have already learned to do.

The kernel here should not consist of only the zero vector - an eigenvector cannot be the zero vector.