Cylindrical pipe holding up a screen (real world project)

[massta]

This is a real world project. I'm building a giant roll-up window shade to be used for a special effects green screen.

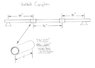
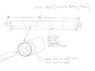
The first real model attempt (without math) failed. See the two pictures of the tube structure being held up by chairs. The only axle was about 3ft of 1.5" pipe at each end. The rest is 8" diameter concrete tubes. We double walled the tubes by slicing the length of one and using it as a coupler inside to hold two tubes end to end. We made about twelve 3/4" wooden disks to help support the structure and secure to the axle. Deflection in the middle was too great.

Second attempt in next post.

 

[massta]

Second attempt will be similar but with an axle pipe running the length of the tube.
I'm nervous this will deflect also.

Here are my drawings of the stages to completed.

Basic drawing:
This might be the easiest place to start. The screen only weights 23lb total and will be even across the entire beam.

Coupler drawing:
Because I can't order large lengths of pipe, sections will need to be welded. This adds weight and weak points. I have some samples of pipe here. Two ends can be welded, then a coupler pipe slid over and welded also.

Outer shell drawing:
Because the screen needs to be rolled up and down, we need an 8" diameter concrete tube which will take 9-10 revolutions to wrap it up. An option here would be to use even larger diameter tubes (note: wall thickness is the same for all diameter tubes).


Math to follow on next post.

 

[massta]

Moment is force times distance.

My thinking is because it's supported at both ends, I can run math for half of the beam.
But what is my force?

Weight:
Support to middle of center pipe: 10.75' x 1.343lb = 13.43lb
One coupler: 1' x 2.73lb = 2.73lb
Outer shell: 10' x 1.2lb = 12.0lb
1/2 of green screen weight: 11.5lb

total half weight of beam: 39.66lb

Moment (M) = Force x Distance
M = 39.66lb x 129in, == 5116.14in-lb

Let me consider the stress of the basic single pipe/beam model:
Stress (S) = M x C/I
C = outside radius of pipe (1")
I = moment of Inertia of pipe

I = pi x OD^4/64
= 3.14159 x 2^4/64 == 0.7854 in^4

and S = 6514.06psi

What will be the deflection?
D = (F x L^3) / (3 x E x I)
Where:
D = Deflection at the center of beam
F = Force at center of beam (39.66lb)
L = Length to center (129in)
E = Young's modulus for steel material (30,000,000 psi)
I = moment of Inertia (0.7854 in^4)
D = (39.66 x 129^3) / (3 x 30,000,000 x 0.7854)
== 1.205 inches

This basic model, if I'm correct, is bending quite a bit.
There is probably some other weight I didn't factor in so this will be a minimum deflection.
Other things to consider:
Strength of welded coupler. Could this increase deflection or decrease it?
Strength of the concrete tubes (with wooden disks, note: there will be concrete tube couplers here as well, just not the entire length like in the first attempt).

My thinking is the concrete tubes will add to the deflection making this attempt a failure on paper.

Any ideas are very welcome. There is another model for rolling up the this green screen. Remember, the screen is very light. The problem is that its very wide: 20ft and needs to be rolled up somehow.

Thanks,
~Shawn

 

[Straw_Cat]

You could use some thick rigid foam panels (the kind used for insulation) to stiffen the tube. 1 sheet of it should do the job nicely, and they don't cost that much. A Stanley Sureform will be useful for shaping the foam so it fits snugly inside the sonotube.
You can make gussets for the joints out of salvaged door skin mahogany (try craigslist for a door, or go to a Habitat For Humanity ReStore if you have one nearby) and glue them on with construction glue.

 

[massta]

I just found this company and it's 4" aluminum pipe with 0.5" max deflection:
http://www.phototechinc.com/102612_pwrroller/102712_pwrroller.htm

costs $400 plus another $175 shipping, ouch

Wish I thought of the rigid foam idea, just don't want to waste any money building another tube and have it fail when I could of just bought one.

Thanks for all the help. ~Shawn

 

[Jupiter6]

I just found this company and it's 4" aluminum pipe with 0.5" max deflection:
http://www.phototechinc.com/102612_pwrroller/102712_pwrroller.htm

costs $400 plus another $175 shipping, ouch

Wish I thought of the rigid foam idea, just don't want to waste any money building another tube and have it fail when I could of just bought one.

Thanks for all the help. ~Shawn

With that span, I think you're going to get deflection no matter what you do if you want to keep it light weight. My first thoughts would be a steel tube within a PVC outer tube.

You want to get the structure radially outwards as far as possible to increase the I.

List the constraints of your project, because steel is certainly cheaper than Alum.

 

[rollingstein]

Moment is force times distance.

What will be the deflection?
D = (F x L^3) / (3 x E x I)
Where:
D = Deflection at the center of beam
F = Force at center of beam (39.66lb)
L = Length to center (129in)
E = Young's modulus for steel material (30,000,000 psi)
I = moment of Inertia (0.7854 in^4)
D = (39.66 x 129^3) / (3 x 30,000,000 x 0.7854)
== 1.205 inches

Shouldn't you be using this equation, but with full beam weight?

That'd give you a computed deflection less than 0.5 inches. What's your design limit?

 

[rollingstein]

I = pi x OD^4/64
= 3.14159 x 2^4/64 == 0.7854 in^4

Isn't that MI for a solid pipe? Your pipe is hollow.MI will be a lot less then (for same OD!).

 

[rollingstein]

Besides that's the worst case scenario: A concentrated center load. A uniformly loaded beam will deflect a lot less I think. Probably ~60% of the concentrated center load case?

Also, wouldn't supporting it on chairs exaggarate the deflection than a real mount? How are you going to mount the ends? A fixed support end condition should give perhaps ~30% of the beam center deflection as a simply supported end point. I'm not sure which one is a realistic assumption for your mounts but I suspect the chair-mount misleads you.

PS. Apologies if any of this is wrong; I'm no structural expert. I'm glad to be corrected.

 

[rollingstein]

Worst case, could you add a set of small support rollers at the center for support? The screen can squeeze between them and the main roller. Not sure if you know what I mean?

 

[256bits]

Worst case, could you add a set of small support rollers at the center for support? The screen can squeeze between them and the main roller. Not sure if you know what I mean?

Probably a good idea. Support the roller from the back and the screen can roll/unroll from the front with the green side out.
I think that limits the deflection of the 2 half lengths to 1/16 of that of the longer beam if this technique is viable.

 

[massta]

@rollingstein, you are correct. I made the mistake of using a solid beam.

For hollow tube:
I = pi * (OD4 - ID4) / 64
Where:
pi = 3.14159
OD = the outside diameter (2 in)
ID = the inside diameter (1.87 in)

so I = 0.185142 in^4
plug in new I for Deflection:
D = (F * L^3) / (3 * E * I)
D = 5.11 in !

answer: steel is too flexible

@strawcat, I like your idea of using foam to support it. You've got me thinking. I wondering how much flex 2"x8"x20' flex. This could work if I cut and placed two 2"x3"x20" strips along the edge to make it a double I-beam inside an 8 inch tube (like a cross). But foam doesn't come in super long pieces and would need to be connected some how.

@jupiter6, some people have suggested PVC but it's very heavy and I suspect flex, but will pick them up again to check at the hardware store tomorrow. This adds to the force.

@rollingstein and 256bits, a center support has been thought out. It would only work if it was spring loaded and moved up and down at the diameter changed when the screen unwound and wound.

Thanks for all your help!
The best answer I think is using foam. If I could get larger concrete tubes, say 12" diamter, then I could fit foam triangles inside to support, much like a bridge. 45 degree triangular support is the best strength to weight ratio. hmmm Seems like a ton of work when I can purchase a beam out of aluminum and not have to make anything, haha. Feel free to keep this going.

 

[rollingstein]

@rollingstein, you are correct. I made the mistake of using a solid beam.


plug in new I for Deflection:
D = (F * L^3) / (3 * E * I)
D = 5.11 in !

answer: steel is too flexible

Isn't this still the wrong formula for D? You seem to be using a cantiliver eq. The beam eq. gives you a lesser deflection. Especially adding in uniform loading and restrained supports I doubt you'd see more than 0.5 inches.

5 inches sounds like a lot.

 

[rollingstein]

Did some number crunching:

Uniform Load of 0.3 lb/in and fixed supports gives max deflection 0.6 inch

Even if you consider all weight (80 lb) concentrated at beam centre I get a deflection of 1.2 inches with fixed supports.

Main problem is roller supports; then deflections jump to the 3-5 inch range.

 

[massta]

I'd like to see your formula.
The only support is at then end, I understand the force is even throughout the beam but it only has one support so the entire force (or 1/2 because there are two supports) are enacted here. That said, all of the weight isn't at the middle, it's spread out. So this is where I could use some help.
~Shawn

 

[Q_Goest]

Hi Shawn. Welcome to the board,
For a tubular structure, moment of inertia I = \pi/64 (D_o⁴-D_i⁴)

For a beam, uniformly loaded with SIMPLY SUPPORTED ends, the equations for stress and maximum deflection are:
The maximum moment is m = w L² / 2
where w = linearly distributed load in units of force per unit length. Make sure to add ALL contributions to weight including the pipe/tube and the stuff it's supporting.
Stress = m D_o/(2 I)
Deflection = 5 w L⁴ / (384 E I)
where E = modulus of elasticity of the pipe/tube material.

For a beam, uniformly loaded with FIXED ends, the equations for stress and maximum deflection are:
The maximum moment is m = w L² / 12
Stress = m D_o/(2 I)
Deflection = w L⁴ / (384 E I)

Obviously, fixing the ends so they can’t deflect will reduce the maximum deflection at the center of the span by 80%, so that's a lot better than simply supported ends. I'd suggest making up a spreadsheet to see how changing various inputs changes the output.

If you need to reduce the deflection of the span further and make it essentially flat, you could use one of the equations above and make a beam that’s bent to that curve, fix the ends as per the equation and then when it’s loaded, it will flatten out.* Of course, if this bent bar were to rotate, it would be horrible, so you would want to keep the bar steady and have some way of having the screen rotate on the bar such that the bar doesn’t need to rotate.

One last option would be to take a pipe/tube and deflect the ends slightly so that you put a moment on the ends of the bar so as to help reduce the sag in the middle. The bar could then rotate if you wish, unlike the other option above. In other words, imagine holding a thin plastic bar horizontally out in front of you and watching it sag in the middle, then twist your hands so the sag comes out of it. You could do the same here and allow the pipe/tube to rotate on bearings but the bearings would be canted slightly so the sag is reduced. Not sure if that would completely eliminate the deflection, I'd have to think about the equations, but that's another possibility.

Additional information here: http://www.engineersedge.com/beam_calc_menu.shtml

*For example, you may have seen 18 wheelers driving down the road pulling a flatbed with no load and noticed they often have a bow to them. The flatbed trailer will bend under load so that it ends up nearly flat.

 

[rollingstein]

If you need to reduce the deflection of the span further and make it essentially flat, you could use one of the equations above and make a beam that’s bent to that curve, fix the ends as per the equation and then when it’s loaded, it will flatten out.* Of course, if this bent bar were to rotate, it would be horrible, so you would want to keep the bar steady and have some way of having the screen rotate on the bar such that the bar doesn’t need to rotate.

Great idea. OTOH, so long as he doesn't rotate this unloaded why should it be horrible?

Under load shouldn't the bent bar be essentially flat be design? i.e. it is only bent unloaded?

 

[rollingstein]

If you need to reduce the deflection of the span further and make it essentially flat, you could use one of the equations above and make a beam that’s bent to that curve, fix the ends as per the equation and then when it’s loaded, it will flatten out.* Of course, if this bent bar were to rotate, it would be horrible, so you would want to keep the bar steady and have some way of having the screen rotate on the bar such that the bar doesn’t need to rotate.

One last option would be to take a pipe/tube and deflect the ends slightly so that you put a moment on the ends of the bar so as to help reduce the sag in the middle. The bar could then rotate if you wish, unlike the other option above. In other words, imagine holding a thin plastic bar horizontally out in front of you and watching it sag in the middle, then twist your hands so the sag comes out of it. You could do the same here and allow the pipe/tube to rotate on bearings but the bearings would be canted slightly so the sag is reduced. Not sure if that would completely eliminate the deflection, I'd have to think about the equations, but that's another possibility.

@Q_Goest

What's the difference between your two ideas? I like them, but they somehow seemed very similar to me. Is it just the difference between elastic and permanent strain?

 

[Q_Goest]

@Q_Goest

What's the difference between your two ideas? I like them, but they somehow seemed very similar to me. Is it just the difference between elastic and permanent strain?

Thanks. It's kinda hard to explain without pictures. But with the first one, you don't allow the bar to rotate and instead you have the screen rotate around the bar. The bar would be bent prior to installation and then when under load it would straighten out, kinda like a flatbed truck.

For the other idea, you have a straight bar that you allow to rotate but you tweak the ends slightly to help eliminate the sag. So you actually put a moment on the ends of the bar which helps to cancel the moment created in the bar by the linearly distributed load. The stresses would never exceed yield so the bar would be straight if you removed it from the installation and laid it on the ground for example.

 

[rollingstein]

Thanks. It's kinda hard to explain without pictures. But with the first one, you don't allow the bar to rotate and instead you have the screen rotate around the bar. The bar would be bent prior to installation and then when under load it would straighten out, kinda like a flatbed truck.

If the inner bar won't rotate it may be more cost-efficient to go for an I beam / box or some similar non symmetric section?

You only need a high MI to resist deflection along the direction gravity acts. A cylinder might be wastefully exuberant.

 

[AlephZero]

A cylinder might be wastefully exuberant.

If you need a cylinder to roll the screen around, you might as well use it to provide stiffness as well.

The bottom line is that I for a thin cylinder is approximately $\pi r^3 t$ but the mass is proportional to $rt$. Also the deflection is proportional to w/I.

So ignoring the weight of the screen compared with the cylinder, changing the thickness will not reduce the deflection (w/I stays the same) but the deflection is proportional to $1/r^2$.

So what you need is a large radius cylinder.

If you try to make an internal non-rotating support, it will need to have a significant depth to get the stiffness, so you will still need a big cylinder radius to fit around it.

If you could split the screen into two parts with the two rollers at a shallow angle, so the two screens overlap when they are unrolled, you could have a central support, and halve the length of each roller, which would make a big difference.

 

[massta]

For the other idea, you have a straight bar that you allow to rotate but you tweak the ends slightly to help eliminate the sag. So you actually put a moment on the ends of the bar which helps to cancel the moment created in the bar by the linearly distributed load. The stresses would never exceed yield so the bar would be straight if you removed it from the installation and laid it on the ground for example.

This idea is great. By deflecting the ends with a special bracket, it will put an opposite deflection in the beam. When the beam has a load it will straighten out. Only problem is the beam will bow when the screen is unrolled, so better to make it as stiff as possible.

Going through the numbers again for a simple supported beam:

Pipe/beam:
OD: 2in
ID: 1.87in
Length between simple supports: 258in
Total weight/load: approx 80lbs (includes all materials) or 0.2899lb/in
L = Length (258in between supports)
E = Young's modulus for steel material (30,000,000 psi)
I = pi/64 * (OD^4 - ID^4); I = 0.18514in^4

Deflection: d = w * OD^4 / (384 * E * I)
d = 0.2899lb/in * (2^4) / (384 * 30,000,000 * 0.18514in^4)
d = an incredibly small number!

making a mistake somewhere...ugh
so deflection has nothing to do with Stress or Moment?

 

[Q_Goest]

This idea is great. By deflecting the ends with a special bracket, it will put an opposite deflection in the beam. When the beam has a load it will straighten out. Only problem is the beam will bow when the screen is unrolled, so better to make it as stiff as possible.

Going through the numbers again for a simple supported beam:

Pipe/beam:
OD: 2in
ID: 1.87in
Length between simple supports: 258in
Total weight/load: approx 80lbs (includes all materials) or 0.2899lb/in
L = Length (258in between supports)
E = Young's modulus for steel material (30,000,000 psi)
I = pi/64 * (OD^4 - ID^4); I = 0.18514in^4

Deflection: d = w * OD^4 / (384 * E * I)
d = 0.2899lb/in * (2^4) / (384 * 30,000,000 * 0.18514in^4)
d = an incredibly small number!

making a mistake somewhere...ugh
so deflection has nothing to do with Stress or Moment?

Sorry, my bad. I had D_o instead of L in the deflection equation. I've edited the post. You should get a deflection of 0.8967"

 

[massta]

Pipe/beam:
OD: 2in
ID: 1.87in
Length between simple supports: 258in
Total weight/load: approx 80lbs (includes all materials) or 0.2899lb/in
L = Length (258in between supports)
E = Young's modulus for steel material (30,000,000 psi)
I = pi/64 * (OD^4 - ID^4); I = 0.18514in^4

Deflection: d = w * L^4 / (384 * E * I)
d = 0.2899lb/in * (258in^4) / (384 * 30,000,000psi * 0.18514in^4)
d = 94.9 in

How did you get 0.8967?
hmmm...

I was using this eq. in my original math:
D = (F x L^3) / (3 x E x I)

 

[Q_Goest]

Deflection: d = w * L^4 / (384 * E * I)
d = 0.2899lb/in * (258in^4) / (384 * 30,000,000psi * 0.18514in^4)

Check again. You should get 0.6022. I accidentally input 285" in length and got .8967".

The Superbowl is being distracting! lol

Oh... and when deflecting the ends of the beam you would maintain a moment on the ends of the beam so that as it rotates it remains relatively straight. You might imagine a couple of rolling element bearings on the end of the beam so that they tend to point the beam upwards toward the center. Those bearings would simply maintain a moment on the end of the beam tending to flatten the curvature. The beam wouldn't change shape as it rotates so the sag in the beam wouldn't change as it turns. Think about it...

 

[Q_Goest]

Deflection: d = w * L^4 / (384 * E * I)
d = 0.2899lb/in * (258in^4) / (384 * 30,000,000psi * 0.18514in^4)
d = 94.9 in

How did you get 0.8967?
hmmm...

I see what you did. You raised the moment of inertia (0.1851 in⁴) to the 4'th power. Those are just units. Understand?

 

[nvn]

massta: You would want to keep the design as simple as possible. Therefore, you would want to use just a simply-supported beam, which is just your steel pipe. Roll your screen directly onto your steel pipe. Rotate the pipe faster, to obtain the same effect.

Therefore, for your current given data, the simply-supported beam midspan deflection would be, y = 76.49 mm, which is fine. This is not a huge deflection, and is OK. If you want the deflection to be less, then simply use a larger-diameter SAE 4130 steel pipe. If you choose a larger-diameter steel pipe, then give us the pipe OD and ID, and we can check your simply-supported deflection calculation.

 

[nvn]

massta: You perhaps could run a tight string line (or strong thread) across the room, then loosen it until it sags 76 mm. Then see if this amount of sag is noticeable or acceptable to you. If not acceptable, gradually tighten the string line, until you determine the maximum amount of sag (deflection) you find acceptable. Next, find what SAE 4130 steel pipe/tube sizes are available to you, then compute the deflection, to see if it exceeds your maximum allowable deflection. In this way, you can determine the minimum steel pipe/tube size (OD and ID) required.